Picture Proof: Quadrilaterals Tessellate through Angle Sum Property
Exploring the concept of tessellation with quadrilaterals by splitting them into triangles based on the angle sum property. The collection of images provides visual proofs demonstrating how all quadrilaterals tessellate effectively.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Note to Teacher Challenge the pupils to draw a quadrilateral that won t tessellate. They could draw these on the whiteboard. You can then replicate it using the Geogebra file. Once they are happy that you can do it every time you can show them a picture proof of the fact that all quadrilaterals tessellate.
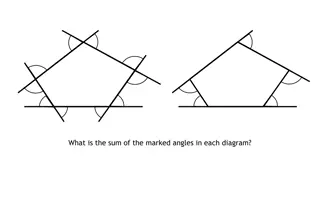
A Picture Proof that all Quadrilaterals Tessellate A quadrilateral can be split into two triangles, each containing 180 , therefore the sum of the internal angles in a quadrilateral is 360 . Let s add more tiles identical to the original above, in a systematic way.
A Picture Proof that all Quadrilaterals Tessellate Wherever four tiles meet, each one offers a different angle. These angles are the interior angles of each tile, which we know sum to 360 , so there are NO GAPS.