Understanding Quadratic Equations and Functions

This collection covers solving quadratic equations by factoring, exploring quadratic function forms, and understanding the attributes of quadratic functions. It also touches on the Zero Product Property, frog jumping functions, and their domains and ranges. The content provides guidance on solving equations, determining coordinates, finding x-intercepts, and interpreting different forms of quadratic functions.

• Quadratic Equations
• Factoring
• Functions
• Zero Product Property
• Graphing

aire_94 Follow

Uploaded on Sep 18, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Quadratic Equations: Solve by factoring Today s Objective: I can solve quadratic equations

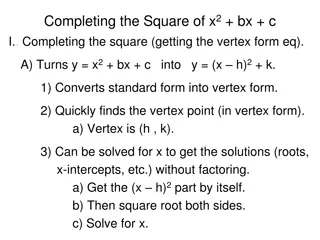
2. Quadratic Function Forms: Review ?(?) = ??2+ ?? + ? ?(?) = ?(? )2+? Standard Form Opens up (a > 0) down (a < 0) y-intercept: (0, c) Vertex: Vertex Form: Opens up (a > 0) down (a < 0) Vertex: (h, k) Axis of symmetry: ? = ? 2?,? ? 2? Axis of symmetry: ? = ? 2?

3. You are part of the tunnel project and need to finish the drawing of the tunnel opening. What are the coordinates of point C? Explain your reasoning x-intercepts Zeros Roots

4. Quadratic Function: Factored Form ?(?) = ?(? ?1)(? ?2) Attributes: Opens: up (a > 0) or down (a < 0) Zeros of the function = Roots (x intercepts) (?1,0) (?2,0) Zeros ? ? = 0

5. Solving Quadratic Equations: by factoring Zero Product Property: 1. Equation equal to 0 2. Factor completely 3. Set factors equal to 0 and solve. 2?2 3? = 9 2?2 3? 9 = 0 If ab = 0, then a = 0 or b = 0 ?2 7? + 12 = 0 2?2 2? 60 = 0 2(?2 ? 30) = 0 2?2 6? + 3? 9 = 0 (? 3)(? 4) = 0 2(? + 5)(? 6) = 0 (2? + 3)(? 3) = 0 ? 4 = 0 ? 3 = 0 ? 6 = 0 ? + 5 = 0 ? 3 = 0 2? + 3 = 0 ? = 3 ? = 4 ? = 5 ? = 6 ? = 1.5 ? = 3

6. Frog Jumping The graph shows a function modeling the height of one frog s jump, where x is the distance in feet from the jump s start. Pg. 229 #9-17, 36, 40, 41 How far did the frog jump? 20.34 feet How high did the frog jump? 0.029?2+ 0.59? = 0 .029? .029? 0.029(10.17)2+ 0.59(10.17) = 0.029? ? 20.34 = 0 3.0 feet What is a reasonable domain and range for this frog jumping function? 0 ? 30 ? 20.34 = 0 0.029? = 0 ? = 0 ? = 20.34 0 ? 5