Problem Solving with Quadratic Functions in Real Life Situations
Wednesday, 18 September 2024
Wednesday, 18 September 2024
Problem solving with quadratic
functions
LO: Find the equation of a quadratic functions in real
life situations.
Quadratic functions are everywhere
Quadratic functions are everywhere
Quadratic functions are everywhere
Quadratic functions are everywhere
Quadratic functions are everywhere
is reached
when
Finding the equation of the function from its graph
(a) How long does the rocket take to reach its maximum height?
The height of a rocket
t
seconds after it is fired upwards is
given by
We see that
a
= –5
The maximum height
t
= 10
H(t)
= 100
t –
5
t
2
metres,
t
≥ 0
.
So, the shape of the graph open
downwards
10
t
H(t)
Re-ordering the terms
H(t)
= 100
t –
5
t
2
H(t)
=
–
5
t
2
+
100
t
maximum height
The maximum height is
reached after 10 seconds
Finding the equation of the function from its graph
(b) Find the maximum height reached by the rocket.
The height of a rocket
t
seconds after it is fired upwards is
given by
Simplifying
H
(10)
= 500
H(t)
= 100
t –
5
t
2
metres,
t
≥ 0
.
H
(10)
= 1000
–
500
10
t
H(t)
500
Expanding
H
(10)
= 100(10)
–
5(10)
2
H
(10)
= 1000
–
5(100)
maximum height
The maximum height reached is 500 m
Finding the equation of the function from its graph
(c) How long does it take for the rocket to fall back to Earth?
The height of a rocket
t
seconds after it is fired upwards is
given by
Solving for
t
t –
20
=
0
H(t)
= 100
t –
5
t
2
metres,
t
≥ 0
.
t
= 0
10
t
H(t)
500
Factorising
H
(
t
)
= 0
0
=
–
5
t
maximum height
The rocket falls back after 20 minutes
The rocket will fall back to Earth
when
0
=
–
5
t
2
+
100
t
(
t
–
20)
t
= 20
20
Consider the following bridge.
AB is the longest vertical support of the bridge
A
B
The vertical supports are 10m apart.
If axes are drawn on the diagram of the bridge with x-axis
the road
Hence, determine the lengths of the other vertical supports.
(
0
,
4
0
)
.
(
1
0
0
,
0
)
.
(
-
1
0
0
,
0
)
.
which contains
its length is 40m
a parabolic arch,
and y-axis on AB,
find the equation of the
parabolic arch.
Finding the equation of the function from its graph
Since the x-intercepts are given, start
with the
equation
in
factorised
form
Using the information provided in the problem, we sketch the
graph.
We know that y = 40 when x = 0
x
-intercepts
(
p, 0
)
(
q, 0
)
= (-100
, 0
)
= (100
, 0
)
f(x)
=
a
(
x – p
)
(
x – q
)
f(x)
=
a
(
x – 100
)
(
x + 100
)
y
-intercept
(0
,
40)
Substitute these values into the
equation to find
a
40
=
a
(
0 + 100
)
(
0 – 100
)
40
=
-10 000a
-0.004
a
=
f(x)
=
– 0.004
(
x – 100
)
(
x + 100
)
f(x)
= –
0.004
x
2
+ 40
(0, 40)
100
x
y
-100
Finding the equation of the function from its graph
Since the vertical supports are 10 m
apart, we are going to calculate
f
(
x
)
The equation of the parabolic arch is
f(x)
= –
0.004
x
2
+ 40
= 39.6
For
x
= 10 or -10
f(x)
= –
0.004(10)
2
+ 40
(0, 40)
100
x
y
-100
= 38.4
For
x
= 20 or -20
f(x)
= –
0.004(20)
2
+ 40
= 36.4
For
x
= 30 or -30
f(x)
= –
0.004(30)
2
+ 40
= 33.6
For
x
= 40 or -40
f(x)
= –
0.004(40)
2
+ 40
= 30
For
x
= 50 or -50
f(x)
= –
0.004(50)
2
+ 40
= 25.6
For
x
= 60 or -60
f(x)
= –
0.004(60)
2
+ 40
= 20.4
For
x
= 70 or -70
f(x)
= –
0.004(70)
2
+ 40
= 14.4
For
x
= 80 or -80
f(x)
= –
0.004(80)
2
+ 40
= 7.6
For
x
= 90 or -90
f(x)
= –
0.004(90)
2
+ 40
A vegetable gardener has 40 metres of fencing to enclose a
rectangular garden plot where one side is an existing brick wall.
If the gardener has 40 metres of
fencing the three sides add up to
40
If the width is
x
metres,
x
40
–
2
x
x
y
40 =
x
+
x
+
y
40
–
2
x
=
y
Calculate the area of the rectangle
A
= x
(40 - 2
x
)
A
= –
2
x
2
+
40
x
find the length and the area of the
garden in terms of
x
A vegetable gardener has 40 metres of fencing to enclose a
rectangular garden plot where one side is an existing brick wall.
Find the width of the garden with an area of 192 m
2
x
40
–
2
x
x
y
x
= 8
A
= –
2
x
2
+
40
x
192
= –
2
x
2
+
40
x
2
x
2
–
40
x +
192
=
0
x
= 12
If the width is 8 metres the
length is 24
If the width is 12 metres the
length is 16
A vegetable gardener has 40 metres of fencing to enclose a
rectangular garden plot where one side is an existing brick wall.
Find the maximum area the garden can have
x
40
–
2
x
x
y
(10, 200)
A
= –
2
x
2
+
40
x
The easiest way to find the
maximum area is to graph the
function
y
= –
2
x
2
+
40
x
where
y
is the area and
x
is the width
Using your GDC you can
calculate the vertex
Is the highest point on the
graph, and tells you the
maximum area occurs when
the width of the garden is 10
metres, the area is 200 m
2
Thank you for using resources from
https://www.mathssupport.org
If you have a special request, drop us an email
info@mathssupport.org
For more resources visit our website
Quadratic functions play a key role in solving real-life problems. This content explores finding the equation of a quadratic function in scenarios such as determining the time taken for a rocket to reach its maximum height, finding the maximum height reached by the rocket, and calculating the time it takes for the rocket to fall back to Earth. Through these illustrations, the practical application of quadratic functions is demonstrated, offering insights into their relevance and usefulness.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Wednesday, 18 September 2024 Problem solving with quadratic functions LO: Find the equation of a quadratic functions in real life situations. www.mathssupport.org
Quadratic functions are everywhere www.mathssupport.org www.mathssupport.org 2 of 48
Quadratic functions are everywhere www.mathssupport.org www.mathssupport.org 3 of 48
Quadratic functions are everywhere www.mathssupport.org www.mathssupport.org 4 of 48
Quadratic functions are everywhere www.mathssupport.org www.mathssupport.org 5 of 48
Quadratic functions are everywhere www.mathssupport.org www.mathssupport.org 6 of 48
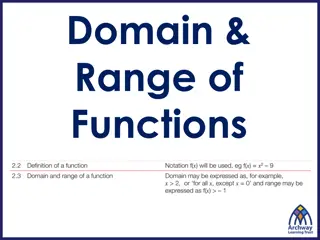
Finding the equation of the function from its graph The height of a rocket t seconds after it is fired upwards is given by H(t) = 100t 5t2metres, t 0. (a) How long does the rocket take to reach its maximum height? H(t) = 100t 5t2 maximum height H(t) Re-ordering the terms H(t) = 5t2 + 100t We see that a = 5 So, the shape of the graph open downwards 10 t The maximum height is reached when t = ? 2? The maximum height is reached after 10 seconds 100 2( 5) = t = 10 www.mathssupport.org 7 of 48
Finding the equation of the function from its graph The height of a rocket t seconds after it is fired upwards is given by H(t) = 100t 5t2metres, t 0. (b) Find the maximum height reached by the rocket. H(10) = 100(10) 5(10)2 H(t) maximum height Expanding H(10) = 1000 5(100) 500 Simplifying H(10) = 1000 500 10 t H(10) = 500 The maximum height reached is 500 m www.mathssupport.org 8 of 48
Finding the equation of the function from its graph The height of a rocket t seconds after it is fired upwards is given by H(t) = 100t 5t2metres, t 0. (c) How long does it take for the rocket to fall back to Earth? The rocket will fall back to Earth when H(t) H(t) = 0 0 = 5t2 + 100t maximum height 500 Factorising (t 20) 0 = 5t Solving for t 10 20 t t = 0 t 20 = 0 t = 20 The rocket falls back after 20 minutes www.mathssupport.org 9 of 48
Consider the following bridge. which contains AB is the longest vertical support of the bridge its length is 40m a parabolic arch, The vertical supports are 10m apart. (0, 40). A B (100, 0). (-100, 0). If axes are drawn on the diagram of the bridge with x-axis the road and y-axis on AB, find the equation of the parabolic arch. Hence, determine the lengths of the other vertical supports. www.mathssupport.org www.mathssupport.org 10 of 48
Finding the equation of the function from its graph Using the information provided in the problem, we sketch the graph. y Since the x-intercepts are given, start with the equation in factorised form f(x) = a(x p)(x q) (0, 40) -100 100 x f(x) = a(x 100)(x + 100) We know that y = 40 when x = 0 Substitute these values into the equation to find a 40 = a (0 + 100)(0 100) 40 = -10 000a a = f(x) = 0.004(x 100)(x + 100) f(x) = 0.004x2 + 40 x-intercepts (p, 0) = (-100, 0) (q, 0) = (100, 0) -0.004 y-intercept (0, 40) www.mathssupport.org 11 of 48
Finding the equation of the function from its graph The equation of the parabolic arch is f(x) = 0.004x2 + 40 y Since the vertical supports are 10 m apart, we are going to calculate f(x) For x = 10 or -10 f(x) = 0.004(10)2 + 40 For x = 30 or -30 f(x) = 0.004(30)2 + 40 (0, 40) -100 100 x = 39.6 For x = 20 or -20 f(x) = 0.004(20)2 + 40 = 38.4 = 36.4 For x = 40 or -40 f(x) = 0.004(40)2 + 40 For x = 60 or -60 f(x) = 0.004(60)2 + 40 For x = 80 or -80 For x = 50 or -50 f(x) = 0.004(50)2 + 40 For x = 70 or -70 f(x) = 0.004(70)2 + 40 For x = 90 or -90 f(x) = 0.004(90)2 + 40 = 30 = 33.6 = 25.6 = 20.4 = 14.4 = 7.6 www.mathssupport.org f(x) = 0.004(80)2 + 40 12 of 48
A vegetable gardener has 40 metres of fencing to enclose a rectangular garden plot where one side is an existing brick wall. If the width is x metres, garden in terms of x find the length and the area of the x If the gardener has 40 metres of fencing the three sides add up to 40 40 = x + x + y y 40 2x 40 2x = y Calculate the area of the rectangle A = x(40 - 2x) x A = 2x2 + 40x www.mathssupport.org www.mathssupport.org 13 of 48
A vegetable gardener has 40 metres of fencing to enclose a rectangular garden plot where one side is an existing brick wall. Find the width of the garden with an area of 192 m2 A = 2x2 + 40x x 192 = 2x2 + 40x 2x2 40x + 192 = 0 y 40 2x x = 12 x = 8 If the width is 8 metres the length is 24 x If the width is 12 metres the length is 16 www.mathssupport.org www.mathssupport.org 14 of 48
A vegetable gardener has 40 metres of fencing to enclose a rectangular garden plot where one side is an existing brick wall. Find the maximum area the garden can have The easiest way to find the maximum area is to graph the function y= 2x2 + 40x where y is the area and x is the width Using your GDC you can calculate the vertex x y 40 2x (10, 200) Is the highest point on the graph, and tells you the maximum area occurs when the width of the garden is 10 metres, the area is 200 m2 x A = 2x2 + 40x www.mathssupport.org www.mathssupport.org 15 of 48
Thank you for using resources from A close up of a cage Description automatically generated For more resources visit our website https://www.mathssupport.org If you have a special request, drop us an email info@mathssupport.org www.mathssupport.org 16 of 48
