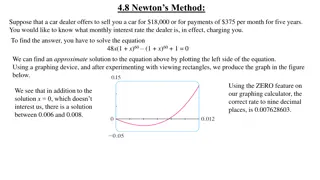
Completing the Square Method: Vertex Form and Solving Equations
The Completing the Square method helps convert quadratic equations from standard form to vertex form, facilitating the quick determination of the vertex point and the solutions without factoring. By completing the square, you transform equations like y = x^2 + bx + c into y = (x − h)^2 + k, enabling easy identification of the vertex at (h, k). The process involves squaring the number, moving it to the other side, and changing its sign, leading to the vertex form. Furthermore, this method also aids in solving equations effectively by isolating and simplifying the squared term to find the value of x.
Uploaded on Sep 12, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Completing the Square of x2+ bx + c I.. Completing the square (getting the vertex form eq). A) Turns y = x2+ bx + c into y = (x h)2+ k. 1) Converts standard form into vertex form. 2) Quickly finds the vertex point (in vertex form). a) Vertex is (h , k). 3) Can be solved for x to get the solutions (roots, x-intercepts, etc.) without factoring. a) Get the (x h)2part by itself. b) Then square root both sides. c) Solve for x.
Completing the Square of x2+ bx + c II.. the number, square the number. Move it on back. A) Take of the b term. 1) write it in (x + )2+ c = y form. B) Square the number you just wrote. 1) Write it on the other side of the = sign. (x + )2+ c = 2+ y [ a # squared is always + ] C) Move the # you just wrote to the other side of the = sign and change its sign (always makes it a negative). Collect. 1) (x + )2+ c = 2+ y 2 D) Now it is in Vertex form: (x + )2+ c = y
Completing the Square of x2+ bx + c Examples: the #, square the #. Move it on back. 1) x2+ 6x + 5 = y 2) x2 12x + 4 = y (x + 3)2+ 5 = 32+ y (x 6)2+ 4 = (-6)2+ y (x + 3)2+ 5 = 9 + y (x 6)2+ 4 = 36 + y 9 36 (x + 3)2 4 = y (x 6)2 32 = y
Completing the Square of x2+ bx + c IV.. Solve using Completing the square (finds solutions). A) Complete the square ( see part II ), but change y to a 0. 1) DON T move it on back. B) Circle the (x h)2part (don t include the a term in circle). C) Isolate the circled part (Steps for Solving Equations). D) Get rid of the exponent with a radical (sq root both sides). E) Simplify the radical & solve for x (radicals give 2 answers).
Completing the Square of x2+ bx + c IV.. Solve using Completing the square (finds solutions). A) Complete the square ( see part II ), but change y to a 0. 1) DON T move it on back. B) Circle the (x h)2part (don t include the a term in circle). C) Isolate the circled part (Steps for Solving Equations). D) Get rid of the exponent with a radical (sq root both sides). E) Simplify the radical & solve for x (radicals give 2 answers). Examples: 5) x2+ 6x + 5 = 0 (x + 3)2+ 5 = 32 step A (x 6)2+ 4 = ( 6)2 (x + 3)2+ 5 = 9 step B (x 6)2+ 4 = 36 5 5 step C 4 4 (x + 3)2 = 4 step D (x 6)2 x + 3 = 2 or 2 step E x 6 = 4 2 or 4 2 3 3 + 6 + 6 x = 1 , 5 x = 6 4 2 6) x2 12x + 4 = 0 = 32