Understanding Parallel and Perpendicular Lines
Students will learn to identify and write equations of parallel and perpendicular lines by understanding the relationship between slopes. Parallel lines have the same slope, while perpendicular lines intersect to form right angles. Through examples and explanations, students will gain the skills to recognize and work with these important concepts in geometry.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
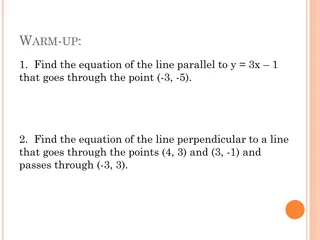
4.3 Writing Equations of Parallel and Perpendicular Lines How can you recognize lines that are parallel or perpendicular? 1. Students will be able to identify and write equations of parallel lines. 2. Students will be able to identify and write equations of perpendicular lines.
1. Students will be able to identify and write equations of parallel lines. Parallel Lines and Slopes Two lines in the same plane that never intersect are parallel lines. Nonvertical lines are parallel if and only if they have the same slopes. That is the key: Parallel lines have the same slopes. All vertical lines are parallel. (this is stated only because vertical lines have no slope)
1. Students will be able to identify and write equations of parallel lines. Remember parallel lines have the same slope. Determine which of the lines are parallel. Find the slope of each line. line a 2 3 1 ( 4)= 1 Line a: ? = line b 5 1 0 1 ( 3)= 1 Line b: ? = 4 line c Line c: ? = 5 ( 4) Lines a and c have the same slope, so they are parallel. 2 ( 3)= 1 5
1. Students will be able to identify and write equations of parallel lines. Write an equation of the line that passes through (5,-4) and is parallel to the line ? = 2? + 3 1. Find the slope the parallel line. The given equation has a slope of 2. So, the parallel line that passes through (5,-4) also has a slope of 2. 2. Use the point-slope form to write the equation of the parallel line in slope-intercept form. ? = 2 ? 5 4 ? = 2? 10 4 ? = 2? 14
You Try!! Remember parallel lines have the same slope. Determine which of the lines are parallel. Find the slope of each line. Line a: ? =4 3 3 0=1 3 line a Line b: ? =2 1 3 0=1 3 line b Line c: ? =0 ( 2) 3 ( 2)=2 line c 5 Lines a and b have the same slope, so they are parallel.
You Try!! Write an equation of the line that passes through (-4,2) and is parallel to the line ? =1 4? + 1 1. Find the slope the parallel line. The given equation has a slope of 1 So, the parallel line that passes through (-4,2) also has a slope of 1 4. 4. 2. Use the point-slope form to write the equation of the parallel line in slope-intercept form. ? =1 4? + 4 + 2 ? =1 4? + 1 + 2 ? =1 4? + 3
2. Students will be able to identify and write equations of perpendicular lines. Perpendicular Lines and Slopes Two lines in the same plane that intersect to form right angles are perpendicular lines. Nonvertical lines are perpendicular if and only if their slopes are negative reciprocals. ? =2 ? = 3 ? =1 ? = 2 ? =1 3 5 2 ? = 5 2 Vertical lines are perpendicular to horizontal lines. (this is stated only because the negative reciprocal of zero is no slope)
2. Students will be able to identify and write equations of perpendicular lines. Remember perpendicular lines have negative reciprocals slopes. Determine which of the lines, if any, are parallel or perpendicular. Line a: ? = 4? + 2 Line b: ? + 4? = 3 Line c: 8? 2? = 16 Write the equations in slope-intercept form. Then compare slopes. Line b: y = 1 Line c: y = 1 Line a: ? = 4? + 2 4? +3 4? 2 4 Lines b and c have slopes of 1 Line a has a slope of 4, the negative reciprocal of 1 so line a is perpendicular to lines b and c. 4, so they are parallel. 4,
2. Students will be able to identify and write equations of perpendicular lines. Write an equation of the line that passes through (-3,1) and is perpendicular to the line ? =1 2? + 3 1. Find the slope of the perpendicular line. The graph of the given equation has a slope 1 because the slopes of perpendicular lines are negative reciprocals, the slope of the perpendicular line that passes through (-3,1) is -2. 2. 2. Use the point-slope form to write the equation of the perpendicular line in slope-intercept form. ? = 2 ? + 3 + 1 ? = 2? 6 + 1 ? = 2? 5
You Try!! Determine which of the lines, if any, are parallel or perpendicular. Line a: 2? + 6? = 3 Line b: y = 3? 8 Line c: 6? + 18? = 9 Write the equations in slope-intercept form. Then compare slopes. Line a: ? = 1 Line c: y = 3? 3 Line b: y = 3? 8 3? 1 2 2 Lines b and c have slopes of 3, so they are parallel. Line a has a slope of 1 so line a is perpendicular to lines b and c. 3, the negative reciprocal of 3,
You Try!! Write an equation of the line that passes through (-3,5) and is perpendicular to the line ? = 3? 1 1. Find the slope of the perpendicular line. The graph of the given equation has a slope 3. because the slopes of perpendicular lines are negative reciprocals, the slope of the perpendicular line is 1 3. 2. Use the point-slope form to write the equation of the perpendicular line in slope-intercept form. ? =1 3? + 3 + 5 ? =1 3? + 1 + 5 ? =1 3? + 6
So lets review! 1. Students will be able to identify and write equations of parallel lines. Parallel lines have the same slopes. 2. Students will be able to identify and write equations of perpendicular lines. Perpendicular lines have negative reciprocal slopes. With parallel or perpendicular lines, once you have the slope find a point and use the point-slope form to write the equation of a line.