Understanding Ratios and Line Segments
Explore the concepts of ratios in relation to line segments, with examples of finding points that divide segments in given ratios. Learn how to determine equations of parallel and perpendicular lines, and apply these concepts to solve problems. Dive into practical scenarios involving wallet bills and rope division to enhance your understanding of ratios and segments.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
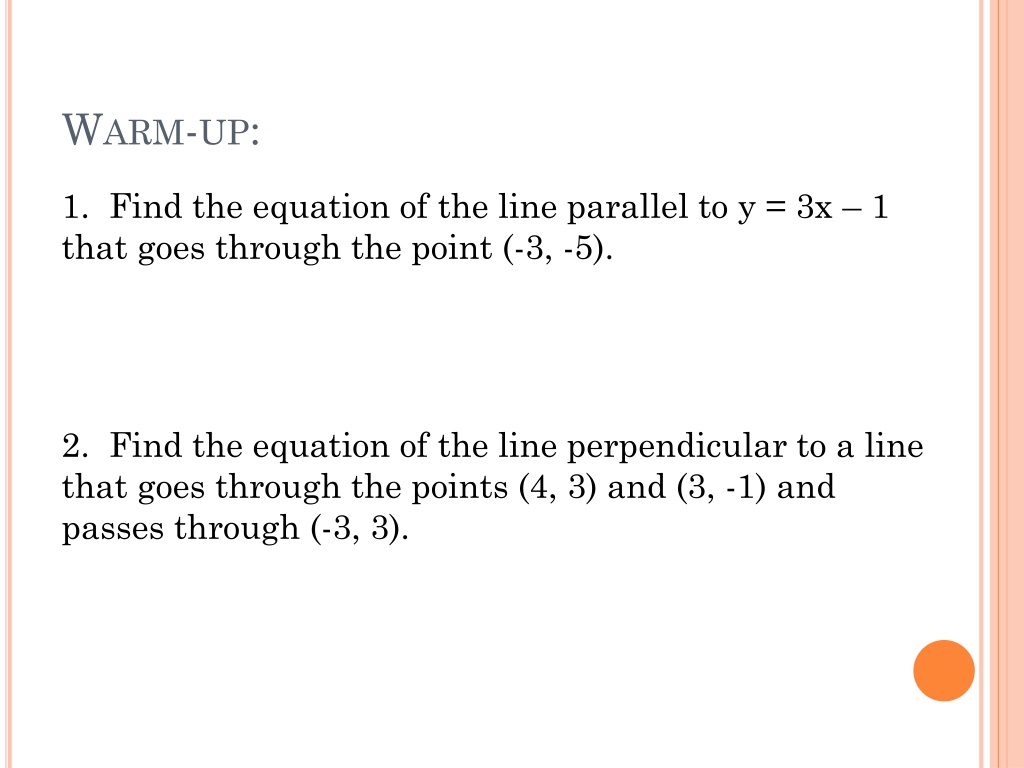
WARM-UP: 1. Find the equation of the line parallel to y = 3x 1 that goes through the point (-3, -5). 2. Find the equation of the line perpendicular to a line that goes through the points (4, 3) and (3, -1) and passes through (-3, 3).
SEGMENT RATIO (PARTITIONING SEGMENT) Unit 11 Notes
WHAT IS A RATIO? A ratio is a comparison of two quantities. A ratio of a to b can be expressed as a:b or ? or a to b ?
LETS PRACTICE Drew has a wallet with 1 $20 bill, 2 $10 bills, 1 $5 bill, and 8 $1 bills. What is the ratio of $1 bills to $10 bills? 1. What is the ratio of $10 bills to the total number of bills in his wallet? 2.
SEGMENT RATIO Point P divides ?? in the ratio 3 to 1. What does this mean? Do you expect point P to be closer to A or closer to B? Why? How does the slope of ?? compare with the slope of ?? ? Why?
THINK ABOUT IT! A 32 foot long piece of rope has a knot tied to divide the rope into a ratio of 3:5. Where should the knot be tied?
EXAMPLE 1 Find the coordinate of point P that lies along the directed line segment from A(3, 4) to B(6, 10) and partitions the segment in the ratio of 3 to 2. Note: Directed line segment tells the direction in terms of which point to start and end. In this case, from A to B.
EXAMPLE 2 Find Q along the directed line segment from R(-3, 3) to S(6, -3) that divides the segment into the ratio 2 to 1.
EXAMPLE 3 Find Q along the directed line segment from R(-2, 4) to S(18, -6) that divides the segment into the ratio 3 to 7.
YOUTRY! Find P along the directed line segment from A(1, 1) to B(7, 3) that divides the segment into the ratio 1:4.
YOU TRY AGAIN! Find P along the directed line segment from A(3, -3) to B(4, 2) that divides the segment into the ratio 3 to 2.
ASSIGNMENT: Partitioning Segment worksheet