Understanding Area and Surface Area in Mathematics
This informative content explains the concepts of area and surface area in mathematics. It emphasizes the distinction between rectangles, rectangular areas, cuboids, and rhombuses to promote accurate calculations. The importance of scale shifts, estimating irregular shapes, and calculating perimeter and area are highlighted. Visual aids and clear explanations aid in grasping these mathematical principles effectively.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
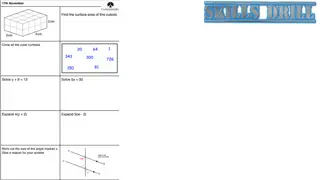
Area of a rectangle Use the mathematical terms rectangle and rectangular (instead of oblong) and rhombus (instead of diamond). Use the mathematical term cuboid (instead of box-like). Specify the shape of an object when you ask students to calculate the area. If it is a rectangle, say so clearly. Otherwise this reinforces a common misconception that the area of any shape is length width. For example, Estimate the abundance of foxgloves in an area 60 m long and 10 m wide is not accurate enough. Tell them it is a rectangular area. The perimeter of a 2-D shape is the distance all around the outside. The area of a 2-D shape is the amount of space inside the shape. It is measured in squared units, mm2, cm2, m2, hectares (1 ha = 10 000 m2) and km2. Shifting between scales and areas is important in geography. You can estimate the area of an irregular shape by drawing around it on cm squared paper and counting the squares. If a shape is close to a rectangle, you can estimate the area by approximating it to a rectangle. The surface area of a 3-D shape is the total area of all the surfaces added together. In maths we use 'area' for 2-D shapes (e.g. a rectangular section of beach) and 'surface area' for 3-D shapes, because it is the area of all the surfaces added together. To calculate the surface area of a cuboid, find the areas of all the faces and add them together.