Understanding Mass Continuity Equations and Convergence-Divergence in Atmospheric Dynamics
Explore the concepts of mass continuity equations in geometric and pressure coordinates, linking them to conservation of mass. Delve into the implications for vertical motion and weather systems' coherence. Understand the role of divergence and convergence in determining vertical motion patterns. Also, examine the significance of positive and negative dipole patterns in vertical motion and pressure variations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
EART30351 Lecture 7
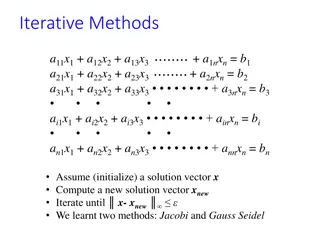
Mass continuity equation 1 Geometric coordinates Mass of element M = x y z z x+ x y x x ? ? ??=?? ?? ? ?? ? ? ? + ?? ? ?? ? ? + ?? ? ?? ? ? + ?? ? ? But ? ?? ? = ? ? + ? ? ? =?? ?? ?
Mass continuity equation 1 Geometric coordinates ? ? ?? =?? Mass of element M = x y z ?? ? ? ? + ??? z ?? ? ? ? + x+ x y 1 ? ? ?? =1 ?? ??+ ?.? = 0 x x ? by conservation of mass. So: ? ? ? ??=?? ?? ? ?? ? ? ? + ?? ? ?? ? ?+ ?? ? ?? ? ? + ?? ? ? 1 ? ?? ??+ ?.? = 0 But ? ?? ? = ? ? + ? ? ? =?? ?? ?
Mass continuity equation 2 Pressure coordinates Mass of element M = g-1 x y p p since ?? ??= ?? x+ x y x x ? ? ??= +1 ? 1 ? ? ? ?? ? ? +1 ? ? ?? ? ? ? ? ? ?? ? ? But ? ?? ? = ? ? + ? ? ? =?? ?? ?
Mass continuity equation 2 Pressure coordinates ? ? ?? =1 ?? ?? ? ? ? + Mass of element M = g-1 x y p ? p 1 ? ? ?? since ?? ??= ?? = ?.? = 0 ? x+ x y x by conservation of mass. So: ?.? = 0 Under the hydrostatic assumption, air behaves like an incompressible fluid! (compressibility of air only important on small scales e.g. sound waves) x ? ? ??= +1 ? 1 ? ? ? ?? ? ? +1 ? ? ?? ? ? ? ? ? ?? ? ? But ? ?? ? = ? ? + ? ? ? =?? ?? ?
Convergence and Divergence Since .V 0, the term divergence is used for the horizontal components only: ??.? = ?? = dp/dt is the vertical velocity in pressure coordinates. Roughly: -g w Patterns of (horizontal) convergence and divergence determine vertical motion ??
Convergence and Divergence Since .V 0, the term divergence is used for the horizontal components only: ??.? = ?? Weather systems are vertically coherent (300 and 700 mb charts qualitatively similar). ?? So, the sign of w and doesn t change in the vertical At the ground, w, = 0 At the tropopause w, 0 since stratosphere restricts vertical motion = dp/dt is the vertical velocity in pressure coordinates. Roughly: -g w Patterns of (horizontal) convergence and divergence determine vertical motion
Convergence-Divergence Dipoles Positive (downward motion) Negative (upward motion) Trop / p < 0 Trop Divergence / p > 0 Convergence Height Pressure Height Pressure / p > 0 Convergence / p < 0 Divergence Convergence aloft means divergence below and vice versa
Divergence of the Geostrophic wind ??=1 1 ? ? ??,? ?? ? = ??,0 Neglecting variation of f with latitude: ?.??=?? ??+?? ??= 0 1 ?2 (?.??=1 = ?? ?? ??? ? ??. ? ? ?? ?? =-?? ?? Actual values around jet stream ~ 3 x 10-5 s-1) ?? ??= ?? ?????, ~5x10-6 s-1. ?
Ageostrophic wind Decompose U into: U = UG + UA Geostrophic and ageostrophic wind. Divergence/convergence therefore depend mainly on departures from geostrophy i.e. UA
Ageostrophic wind Decompose U into: U = UG + UA Geostrophic and ageostrophic wind. From the momentum equation: ?? ??= ? ?? ? By the definition of UG : ?? ??= ?? ?? ??=1 Divergence/convergence therefore depend mainly on departures from geostrophy i.e. UA ?? ?? ?? Ageostrophic wind is proportional to acceleration
Ageostrophic wind around jet stream Acceleration is greatest where wind is greatest, i.e. jet stream UA is perpendicular to the acceleration (x product) UA Jet stream accelerating from left to right (jet entrance) accn
Convergence around jet streak Undisturbed flow, dU/dt = 0 C D UA dU/dt dU/dt Jet UA C D Undisturbed flow, dU/dt = 0 Ageostrophic wind at jet entrance (LHS) points poleward. This means air piles up on the poleward side convergence. As this is near the tropopause it tends to force downward motion and by symmetry we can see a quadrupole pattern
Dines Compensation Tropopause D C C C D D Ground Schematic of synoptic-scale atmospheric circulation overturning cells (showing w rather than vertical velocity) Areas of vertical motion related to vertical dipoles of convergence and divergence
Relation to flow Tropopause J J J H L H Ground Convergence and divergence is greatest at jet stream level: jet stream essentially drives the circulation cells. Convergence aloft => More mass in the column of air => High pressure at surface Divergence aloft => Less mass in the column of air => Low pressure at surface