Understanding Linear Equations in Real-Life Scenarios
Explore the concept of linear equations through real-life examples involving distance, speed, time, and constant rates. Practice solving problems to determine distances covered, areas mowed, and volumes of water coming out of a faucet over specific periods. Engage in workshops and assessments to reinforce learning and apply linear equations in context.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
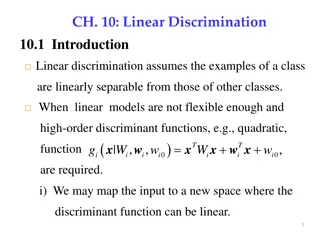
LINEAR EQUATIONS Eureka Math 8thGrade Module 4 Topic B
LESSON 10 Notes, examples(2), workshop
Notes Distance, speed, time Average speed is found by dividing the total distance the total time. d =rt r =d t =d t r Where d = distance, t = time, and r = rate or speed
Example 1 Paul walks 2 miles in 25 minutes. How far can he walk in 137.5 minutes?
Example 2 Dave lives 15 miles from town A. He is driving at a constant speed of 50 miles per hour from his home away from (in the opposite direction of) the city. How far away is Dave from the town after ? hours of driving? Using your equation, how far from town is he after 1 hour?
Workshop Must Do May Do Exponents retest Complete Topic A assessment Lesson 10 cw #1-3 Khan academy Exponents review If tested out of exponents: Crossing the River/Carnival Bears Start Homework
LESSON 11 Examples(2), workshop
Example 1 Pauline mows a lawn at a constant rate. Suppose she mows a 35 sq. ft. lawn in 2.5 minutes. What area can she mow in 10 minutes? t minutes? (time in minutes) Linear Equation: (area in square feet)
Example 2 Water flows at a constant rate out of a faucet. Suppose the volume of water that comes out in 3 minutes is 10.5 gallons. How many gallons come out in t minutes? (time in minutes) Linear Equation: (in gallons)
Workshop Must Do May Do Finish lesson 10 cw #1-3 Lesson 11 cw #1-3 Khan academy Exponents review Topic A assessment corrections If tested out of exponents: Crossing River/Carnival Bears Inky puzzles Integer games
LESSON 12 Warm up, example, notes, workshop
Warm Up Emily tells you that she scored 32 points in a basketball game. Write down all the possible ways she could have scores 32 with only two- and three-point baskets. Use the table to organize your work. If x is the number of two- pointers and y is the number of three-pointers, what equation can we write for the total?
Standard Form of Linear Equations A linear equation in the form: Where A, B, and C represent set numbers, and x and y represent variables or unknowns. + = Ax By C A solution to this equation is an ordered pair (x, y), that makes the equation true. To find solutions, you pick a set number for x or y, substitute, and then solve for the other value.
Slope-Intercept Form of Linear Equations A linear equation in the form: Where m and b represent set numbers, and x and y represent variables or unknowns. = + y mx b m is the constant rate of change (slope) b is the y-intercept (crosses y-axis) (x, y) are solutions that make the equation true
Converting Standard to Slope-Intercept + = -Ax Ax Ax -Ax By BB By C = C + C Ax Ax C = = y B B A C = + y x B B
+ = Example 50 15 x y Choose x-values Let x = 5 Substitute: Solve for y: Choose y-values Let y = 10 Substitute: Solve for x: + = 50 ) 5 ( 15 y + = 50 10 15 x + = 50 10 -10 -10 50 = x (-50) (-50) 5 = 15 x + = 50 ) 5 ( 250 +250 +250 15 15 y + = y 5 = 265 y 1 = x (5, 265) is a solution 50 10 1 10, 10) is a solution (
Workshop Must Do May Do Finish lesson 10&11 cw Exit ticket 10 & 11 Lesson 12 cw #1-5 HINTS: think about why #1-2 small numbers easier! #3 choose y, solve for x #4 choose x that are multiples of 5 Khan academy Inky puzzles
LESSON 13 Discussion/example, workshop
Example 5 1 1 4 2 2 3 -1 -2 7 8
Workshop Must Do May Do Finish lesson 12 cw HINTS: think about why #1-2 small numbers easier! #3 choose y, solve for x #4 choose x that are multiples of 5 Lesson 13 cw #1-3 Khan academy Folder organize Notes sheet
LESSON 14 Explore, discussion/notes, workshop (part2)
Explore Complete Lesson 14 classwork #1-3 and #7-9 SKIP #4-6 and #10-12 FOR NOW Prepare to discuss #3 and #9
Notes When in standard form Ax + By = C: An equation with A=1 and B=0 (in other words: 1x + 0y = C or x = C) will be a vertical line through (C, 0) An equation with A=0 and B=1 (in other words 0x + 1y = C or y = C) will be a horizontal line through (0, C)
Workshop Must Do May Do Finish lesson 12&13 cw Exit ticket 12 & 13 Lesson 14 cw #1-12 #4-6 #10-12 Khan academy Complete all cw & hw Folder organize Notes sheet