Understanding Linear Equations: Basics and Examples
This content covers the basics of linear equations, including their standard form Ax + By = C and how to identify them. It also discusses examples of linear and nonlinear equations, x and y-intercepts, and how to find them. The visual aids and explanations provided make it easier to grasp these fundamental concepts in mathematics.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
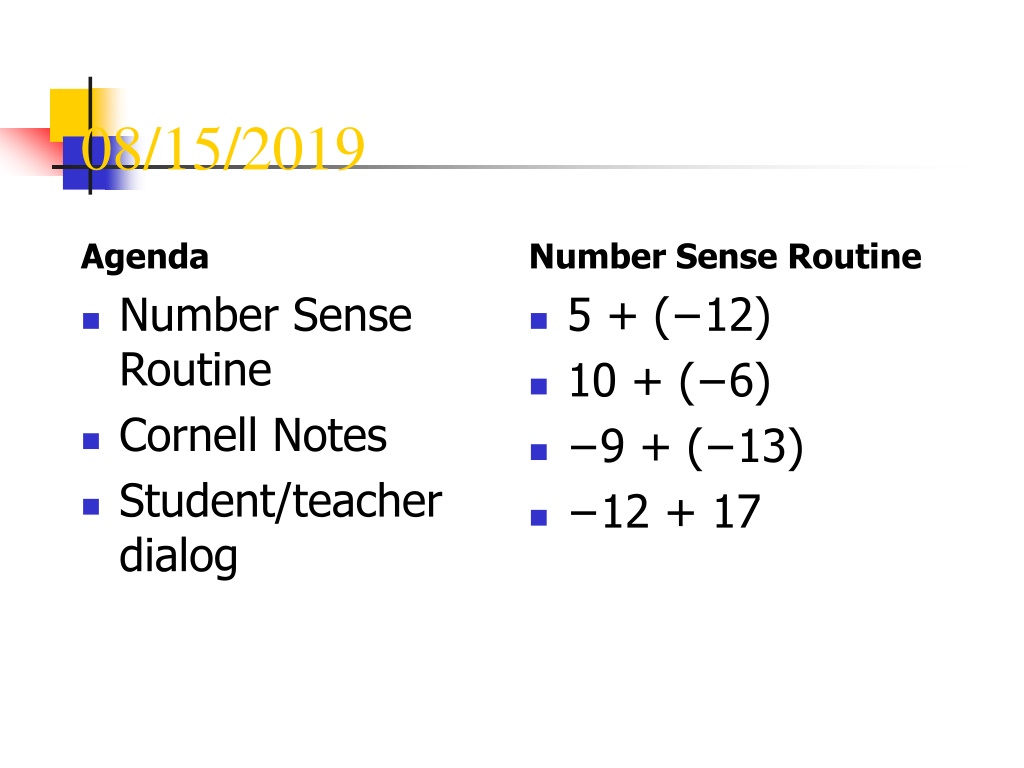
08/15/2019 Agenda Number Sense Routine Cornell Notes Student/teacher dialog Number Sense Routine 5 + ( 12) 10 + ( 6) 9 + ( 13) 12 + 17
Identifying a Linear Equation Ax + By = C The exponent of each variable is 1. The variables are added or subtracted. A or B can equal zero. A > 0 Besides x and y, other commonly used variables are m and n, a and b, and r and s. There are no radicals in the equation. Every linear equation graphs as a line.
Examples of linear equations 2x + 4y =8 6y = 3 x x = 1 Equation is in Ax + By =C form Rewrite with both variables on left side x + 6y =3 B =0 x + 0 y =1 -2a + b = 5 Multiply both sides of the equation by -1 2a b = -5 4 x y = = Multiply both sides of the equation by 3 4x y =-21 7 3
Examples of Nonlinear Equations The following equations are NOT in the standard form of Ax + By =C: The exponent is 2 4x2 + y = 5 xy + x = 5 s/r + r = 3 There is a radical in the equation x = = 4 Variables are multiplied Variables are divided
x and y -intercepts The x-intercept is the point where a line crosses the x-axis. The general form of the x-intercept is (x, 0). The y-coordinate will always be zero. The y-intercept is the point where a line crosses the y-axis. The general form of the y-intercept is (0, y). The x-coordinate will always be zero.
Finding the x-intercept For the equation 2x + y = 6, we know that y must equal 0. What must x equal? Plug in 0 for y and simplify. 2x + 0 = 6 2x = 6 x = 3 So (3, 0) is the x-intercept of the line.
Finding the y-intercept For the equation 2x + y = 6, we know that x must equal 0. What must y equal? Plug in 0 for x and simplify. 2(0) + y = 6 0 + y = 6 y = 6 So (0, 6) is the y-intercept of the line.
To summarize. To find the x-intercept, plug in 0 for y. To find the y-intercept, plug in 0 for x.
Find the x and y- intercepts of x = 4y 5 y-intercept: x-intercept: Plug in x = 0 x = 4y - 5 0 = 4y - 5 5 = 4y = y 4 Plug in y = 0 x = 4y - 5 x = 4(0) - 5 x = 0 - 5 x = -5 5 (-5, 0) is the x-intercept 5 4 (0, ) is the y-intercept
Find the x and y-intercepts of g(x) = -3x 1* y-intercept x-intercept Plug in x = 0 g(x) = -3(0) - 1 g(x)= 0 - 1 g(x) = -1 Plug in y = 0 g(x) = -3x - 1 0 = -3x - 1 1 = -3x = x 3 1 3 1 (0, -1) is the y-intercept ( , 0) is the x-intercept *g(x) is the same as y
Find the x and y-intercepts of 6x - 3y =-18 x-intercept y-intercept Plug in y = 0 6x - 3y = -18 6x -3(0) = -18 6x - 0 = -18 6x = -18 x = -3 Plug in x = 0 6x -3y = -18 6(0) -3y = -18 0 - 3y = -18 -3y = -18 y = 6 (-3, 0) is the x-intercept (0, 6) is the y-intercept
Find the x and y-intercepts of x = 3 x-intercept y-intercept Plug in y = 0. A vertical line never crosses the y-axis. There is no y. Why? There is no y-intercept. x = 3 is a vertical line so x always equals 3. (3, 0) is the x-intercept. x y
Find the x and y-intercepts of y = -2 y-intercept x-intercept y = -2 is a horizontal line Plug in y = 0. y cannot = 0 because y = -2. y = -2 is a horizontal line so it never crosses the x-axis. so y always equals -2. (0,-2) is the y-intercept. x There is no x-intercept. y
Graphing Equations Example: Graph the equation -5x + y = 2 Solve for y first. -5x + y = 2 y = 5x + 2 Add 5x to both sides The equation y = 5x + 2 is in slope-intercept form, y = mx+b. The y-intercept is 2 and the slope is 5. Graph the line on the coordinate plane.
Graphing Equations Graph y = 5x + 2 x y
Graphing Equations Graph 4x - 3y = 12 Solve for y first 4x - 3y =12 -3y = -4x + 12 Divide by -3 12 -3 4 3 Subtract 4x from both sides -4 y = x + -3 Simplify y = x 4 4 3 The equation y = x - 4 is in slope-intercept form, y=mx+b. The y -intercept is -4 and the slope is . Graph the line on the coordinate plane. 4 3
Graphing Equations 4 3 Graph y = x - 4 x y
Reference teachers.henrico.k12.va.us/math/hcpsal gebra2/Documents/2-2/2006_2_2.ppt