Exploring Real-Life Applications of Algebra Through Linear Equations
Dive into the practical application of algebra in everyday situations by understanding how to compare costs using linear equations. Discover the concept of systems of linear equations and how they can help you make informed decisions on the best value. Learn about consistent, inconsistent, and coincident systems and how to solve them using various methods like graphing, substitution, and elimination.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
1/18 School HOLIDAY 1/18 School HOLIDAY MLK, Jr. Day No school for staff or students.
1/19 1/19 Warm Warm Up Up- - Tuesday Math in everyday life. Think of a time you, a family member, or a friend had to compare the cost of products. Choose a thinking map and fill in descriptions, events, or sequences.
1/19 Objective 1/19 Objective I will recognize that math can be used in everyday situations. I will write an essay explaining how I can apply concepts from algebra to solve problems in real life.
1/19 Expository Essay 1/19 Expository Essay
1/19 Concept Builder 1/19 Concept Builder Five Step Writing Process Read prompt carefully, circling key words. Read and annotate the related text. Plan response using a thinking map. Write your response, incorporating your controlling idea (thesis). Read and edit your response for clarity. 1. 2. 3. 4. 5.
1/19 Supporting Details 1/19 Supporting Details S = SPORTS AND/OR STARS (Famous athlete/team or general supporting example from a specific sport; stars from music, TV, film, etc.) B = BOOKS and MOVIES (name the book/movie and describe the character and situation) H = HISTORICAL EXAMPLES (Apollo 11, September 11th, Civil War, etc.) P = PERSONAL EXPERIENCE (Something that has happened to you or someone you know) P = PERSONAL HYPOTHETICAL (Something that could have happened to you or someone you know) N = NEWS (Something you ve seen in the news/current events)
1/19 Article 1/19 Article Sometimes there are different charges for the same service or product at different places. For example, Bowl-a- Rama may charge $2.50 per game plus $2.00 for shoe rental whereas Bowling Pinz may charge $2.00 per game plus $4.00 for shoe rental. A system of linear equations can be used to compare these charges to find the best cost. A system of linear equations is a set of two or more linear equations containing two or more variables. A solution of a system of linear equations with two or more variables is an ordered pair that satisfies each equation in the system. So, if an ordered pair is a solution, it will make both equations true. We can also view the solution as where the equations intersect. We learned that when two lines intersect at a point, there is exactly one solution to the system. Systems with one solution are called consistent. There can also be inconsistent or coincident systems. An inconsistent system is a system that has no solution because the lines are parallel. A coincident system is a system that has an infinite number of solutions because the equations graph the same line. In our class, we learned you can solve systems of linear equations using the graph, substitution, elimination, or matrix methods. The best method to use depends on the forms of the given equations and how precise the solution should be. Another tool we have learned to use in our class is the graphing calculator. The graphing calculator can help us find the best cost through the graphing or matrix method.
1/19 Prompt 1/19 Prompt Write a one page essay (no more than 26 lines) that describes a time you, a family member, or a friend had to compare the cost of products. Then describe how you could have used the graphing calculator to find the best cost. Describe how to use both the graphing and matrix methods.
1/20 Warm Up 1/20 Warm Up- - Wednesday
1/20 Objective 1/20 Objective I will be able to simplify expressions involving zero and negative exponents.
1/20 Review Writing Literacy 1/20 Review Writing Literacy Introduction: Introduction: When shopping for cars, my family wanted to get the best car for lowest cost. We shopped at many places and compared features of the car to the price. To find the best price, we can use the graphing calculator.
1/20 Review Writing Literacy 1/20 Review Writing Literacy Supporting Paragraph 1: Supporting Paragraph 1: One way to use the graphing calculator is the matrix method. When using matrix, you have to line up same features to same features. In this case, I could align the cost of each car along with their interest rate. After that, I would input the information in the graphing calculator under second matrix. Then I have to get out of matrix using second quit. The calculator has to be told which math option to use, so I would go into second matrix again. This time I would go to the math options and move up to B:rref(. After that, I have to name the matrix the calculator needs to use, so I would go back to second matrix and hit [A], then enter, enter.
1/20 Review Writing Literacy 1/20 Review Writing Literacy Supporting Paragraph 2: Supporting Paragraph 2: Another way to use the graphing calculator is the graphing method. When using graphing, the calculator will tell you where the costs intersect. That is the point where your cost for each car would be equal. When graphing, you use the y= function on the calculator. You would put the equations into y1 and y2, then go to graph to see if the lines cross. If they do, you would punch in second, trace, 5, then enter, enter, enter. Graphing shows you a visual of how the costs intersect.
1/20 Review Writing Literacy 1/20 Review Writing Literacy Conclusion: Conclusion: In conclusion, comparing prices is important in getting the most for your money. The graphing calculator is a tool you can use to help you manage your money.
1/20 Concept Builder 1/20 Concept Builder Math Antics- Exponents Video
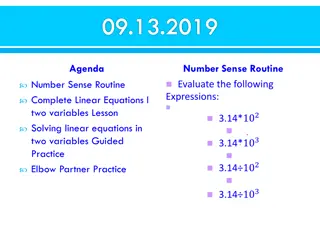
1/20 Concept Review 1/20 Concept Review Exponents are a shorthand way to show how many times a number, called the base, is multiplied times itself.
1/20 Reading Exponents 1/20 Reading Exponents
1/20 Practice 1/20 Practice Base and Exponent Worksheet Expanded and Exponent Form Worksheet
1/21: 1/21: Warm Warm Up Up- - Thursday
1/21 Objective 1/21 Objective I will be able to simplify expressions involving zero and negative exponents.
1/21 Exponent Rules 1/21 Exponent Rules
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Concept Builder 1/21 Concept Builder
1/21 Practice 1/21 Practice
1/21 Practice 1/21 Practice
1/22: 1/22: Warm Warm Up Up- - Friday Product Rule Quotient Rule Power Rule Zero Power Rule Negative Rule Power of 1 Rule
1/22 Practice 1/22 Practice Put into calculator exactly as written. Write answers on back of warm-up.
1/22 Objective 1/22 Objective I will be able to simplify expressions involving the product rule of exponents.
1/22 Exponent Rules 1/22 Exponent Rules
1/22 Concept Builder 1/22 Concept Builder
1/22 Concept Builder 1/22 Concept Builder
1/22 Concept Builder 1/22 Concept Builder
1/22 Concept Builder 1/22 Concept Builder
1/22 Concept Builder 1/22 Concept Builder
1/22 Concept Builder 1/22 Concept Builder
1/22 1/22 Practice (20 minutes) Practice (20 minutes)
1/22 Concept Builder 1/22 Concept Builder
1/22 Concept Builder 1/22 Concept Builder
1/22 Practice 1/22 Practice