Theory of Positive Displacement Pumps: Reciprocating Pumps

Theory Of Positive Displacement

Pumps

CHAPTER 5

1

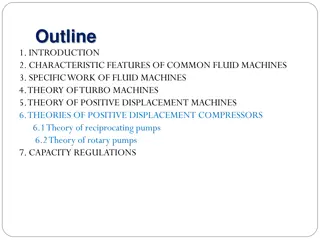
1. INTRODUCTION

2. CHARACTERISTIC FEATURES OF COMMON FLUID MACHINES

3. SPECIFIC WORK OF FLUID MACHINES

4. THEORY OF TURBO MACHINES

5. THEORY OF POSITIVE DISPLACEMENT MACHINES

     5.1 Theory of reciprocating pumps

     5.2 Theory of rotary pumps

6. THEORIES OF POSITIVE DISPLACEMENT COMPRESSORS

7. CAPACITY REGULATIONS

•

Reciprocating

 and 

rotary positive displacement 

pumps are

common in the chemical and process industries.

•

The 

characteristic features 

and 

operating principles 

of the most

common positive displacement pumps are discussed

previously.

•

In this chapter the relation between their 

geometry

 and

performance 

is discussed.

3

THEORY OF POSITIVE DISPLACEMENT

MACHINES

Positive Displacement

Reciprocating

Rotary

-Screw

-lobe

-vane

- Gear

*

-

Piston

-Plunger

-Diaphragm

Theory Of Positive Displacement

Pumps

5.1 Theory Of Reciprocating Pumps

•

Reciprocating pumps include:-



Piston pumps



Plunger pumps 

and



Diaphragm pumps

•

The operating principle of these pumps is the same. Therefore

the theory is discussed based on just one of them, i.e., a 

piston

pump

.

5

6

5.1.1 Capacity of Reciprocating Pumps

•

The capacity of a reciprocating pump is determined by:



 the 

size of the cylinder



the 

number of piston strokes 

or the speed of rotation of the

crank shaft



the 

number of cylinders 

and



the 

number of actions 

(single acting and double acting).

7

•

A single acting-reciprocating pump 

is a pump with only one

side of the piston acting on the liquid.

•

When the two sides act we call it 

double acting

.

8

a. Single acting pumps



The capacity of a single acting reciprocating pump is the

product of the 

displaced volume

, 

the number of double strokes

(rpm), and the 

volumetric efficiency 

as it is given

Where:-

n= number of piston double strokes

      D=Inner diameter of the cylinder (bore diameter)

      S= piston stroke

,



vol

= Volumetric efficiency

 Q= Capacity (Volume flow rate)

9



The 

volumetric efficiency 

takes into account 

leakage through

clearances and suction and discharge valves 

during the suction

stroke and vice versa. It is determined, in the course of pump

tests, by measuring the actual volume of liquid delivered by

the pump per unit time.

Q: Actual volume flow rate

Q’: Theoretical volume flow rate

10



For a reciprocating pump it is common to give the 

slip of the

pump 

instead of the 

volumetric efficiency

.



The slip of the pump is the measure of the total volumetric loss

of the pump given as a fraction of the theoretical capacity.



The slip is given by:-

Normally  



vol

 = 0.7 to 0.97

11

b. Double acting pumps



The capacity of a double acting pump is t

wice 

the capacity of

the single acting minus the reduction in capacity due to the

volume displaced by the connecting rod. Hence,

Where:-  d is the diameter of the rod

12

13

Figure:  multiplex pump



If a pump has 

several cylinders 

of same size with their

pistons 

actuated  by a common crankshaft 

(multiplex pump),

the pump capacity is calculated as the capacity developed

by one times the number of pistons.

c. Multiple Cylinders

Example 5.1

•

The stroke length and bore diameter of a

double acting single cylinder reciprocating

pump are 30cm, and 35cm respectively.  The

diameter of the rod is 22mm.The speed of the

crank is 60 rpm. Determine the capacity of the

pump if the slip of the pump is 5%.

14

Example 5.2

•

The flow rate of a reciprocating pump that

runs at 90 rpm is measured to be 38.2 m

3

/hr.

The stroke length and the internal diameter of

the cylinder are 24cm and 20cm respectively.

Calculate the slip of the pump.

15

5.1.2 Suction and Discharge Pulsations in

Reciprocating Pumps

•

Since liquid is an incompressible medium the velocity of

the liquid inside the cylinder of reciprocating pumps is

the same as the velocity of the piston head.

•

The velocity of the piston head, if it is actuated by crank,

varies with the crank angle.

•

Hence the velocity of the liquid inside the cylinder and

consequently the capacity vary with the crank angle.

Unless special steps are taken, the motion of the liquid in

the suction and discharge pipes will be also non-uniform.

It is this 

non-uniformity that we call pulsation

.

16

•

Pulsation causes several of problems in addition to the non-

uniform delivery.



It 

reduces the NPSHA 

significantly and to avoid cavitaions the

pump should run at a reduced speed. It causes 

mechanical

instability 

of the piping network.



It also causes 

increased power consumption 

due to the

acceleration head involved caused by variation in flow

velocity.

17

The Velocity and Acceleration of the Flow

Medium in Reciprocating Pumps

F

i

g

u

r

e

5

.

2

G

e

o

m

e

t

r

i

c

r

e

l

a

t

i

o

n

s

i

n

a

r

e

c

i

p

r

o

c

a

t

i

n

g

p

u

m

p

18



 The 

velocity of the piston head

19



The ratio L/R is commonly in the range of 4:1 to 6:1.



Lower L/R ratio 

causes high pulsation 

and larger L/R ratio

results in large 

uneconomical power frame

.

Since

When L/R >>1, the motion can be approximated by simple

harmonic motion



The 

Acceleration of the Piston Head 

(plunger)

For simple harmonic 

i.e.,

20

Example 5.2

•

The flow rate of a reciprocating pump that

runs at 90 rpm is measured to be 38.2 m

3

/hr.

The stroke length and the internal diameter of

the cylinder are 24cm and 20cm respectively.

Calculate the slip of the pump.

21

Example 5.3

•

For the pump in Example 5.2 determine the

flow rate and acceleration of the liquid in the

cylinder of the pump at the beginning and

middle of the suction stroke (1) assuming L>>R

and (2) without the assumption in (1) and

taking the length of the connecting rod to be

75cm.

22

The Acceleration Head of the Flow Medium in

the Suction and Discharge Pipes



Because of the acceleration of liquid in the cylinder the liquid

in the suction and discharge pipes also accelerate.



From the continuity equation,

23

Where:-

A

p

= Cross -sectional Area of the piston head

v

p

=velocity of the piston head

A

s

= Flow area of the suction pipe

v

s

= velocity of the liquid in the suction pipe



Therefore the acceleration of the liquid in the 

suction pipe 

is

given by

24



 Similarly for the liquid in the 

discharge pipe

A

d

= Flow area of the discharge pipe



For simple harmonic motion the 

accelerations of the liquid 

in

the 

suction

 and 

discharge pipes 

are given by:-



The 

specific work 

to accelerate the liquid through the 

suction

and 

discharge pipes 

are given by

25

26

F=Force to accelerate the liquid

Y

a,s

= The specific work to accelerate the liquid in the suction pipe

Y

a,d

= The specific work to accelerate the liquid in the discharge pipe

Ls= Portion of the suction pipe through which there is acceleration (pulsation)

Ld= Portion of the discharge pipe through which there is acceleration

m= Mass of liquid in consideration

Hence,



The

 acceleration head

, h

a

=Y

a 

/g is therefore

27



For simple harmonic motion

•

Example 5.4

The dimensions and speed of a single acting single

cylinder reciprocating pump and the dimensions

of the suction pipe are as given below.

S= 32cm, D=30cm, d

s

= 2" (Diameter of suction

pipe), n=50rpm, Ls= 5m

Assume L/R>>1 and

Determine the 

acceleration head 

of the liquid in the

suction pipe at the 

beginning

, 

middle

 and 

end

 of

the suction stroke.

28

5.1.3 The Minimum Pressure

for the Piston to Move in the Cylinder



The piston or plunger of a reciprocating pump should apply a

certain minimum pressure to move inside the cylinder.



This pressure depends on:-



 The 

pump design



Speed 

and the 

piping system

 and



The 

flow medium

29

Where

h

a

= acceleration head in the suction pipe

h

fs

= friction head in the suction pipe

30



The relationship between the total mechanical energy of the flow medium at

point 1 and 2 , is given by:-



Therefore the pressure inside the cylinder, 

P

2

 can be calculated

by:-

Since most commonly 

P

1

=P

atm

Similarly for the discharge stroke



Note that during the discharge stroke h

a

 < 0

since cos



 < 0 for  

>90

0

31

5.1.4 The Minimum Pressure to Open the Suction

Valve



The maximum acceleration head is much larger than the

maximum friction head.



Therefore the minimum pressure occurs when the

acceleration head is maximum, i.e. at 



=0

0

.



At this pressure the friction head is zero, since the velocity

of the flow medium is zero. Hence,

32

33



 For simple harmonic motion , with 



=0

0

.



 The minimum pressure to open the suction valve is:-

5.1.5 The Minimum Pressure to Open the Discharge

Valve



The minimum pressure during the discharge can be similarly

calculated.



The minimum pressure to open the discharge valve can be

calculated using:-

34

5.1.6 The Indicator Diagram



The indicator diagram of a reciprocating pump shows 

the

pressure variations in the cylinder

 and 

valve chest over the

length 

of two piston strokes.

•

The indicator diagram is used to calculate the 

work done 

by

the pump during 

one complete suction and discharge stroke

.

35

Theoretical Indicator Diagram



As the piston moves to the right the pressure inside the

cylinder is reduced and the suction valve is opened while the

discharge valve is closed. The enclosed space of the cylinder is

increased and is filled with liquid coming from the intake pipe

through suction valve.

36

37

S

u

c

t

i

o

n

o

p

e

n

v

d

i

s

c

h

a

r

g

e

c

l

o

s

e

d

S

u

c

t

i

o

n

c

l

o

s

e

d

v

d

i

s

c

h

a

r

g

e

o

p

e

n

S

u

c

t

i

o

n

D

i

s

c

h

a

r

g

e

•

At this point, the pressure in the valve chest is below atmospheric, which is due

to the hydraulic resistance of the suction line. The 

change in pressure 

over the

whole length of rightward stroke of the piston is given by suction line 4-1.

•

When the piston head assumes position 1, the piston reverses its direction of

motion and 

the suction valve is automatically closed

; the pressure in the valve

chest builds up abruptly to its level P

2

. This process is shown by the vertical

line 1-2.

•

At the instant the pressure grows as high as P

2

 the pressure difference across

the discharge valve overcomes the weight and tension of its spring, thus

opening the valve

.

•

As the piston moves steadily from point 2 leftwards, 

the liquid is discharged 

at

constant pressure P

2

.

•

In the extreme left position the piston again reverse its direction of motion. The

result is that the 

pressure in the valve chest drops 

abruptly along line 3-4,

discharge valve is closed and the suction valve is opened

.

•

The pressure–displacement diagram, referred to as 

indicator diagram

, is

completed.

38

  The Indicator Power



is the theoretical power of a reciprocating pump that can be

calculated from the theoretical indicator diagram.



Work done by the piston in any of the strokes can be given as



For the suction stroke



For the discharge stroke



 The total work done in one complete cycle

39



For one revolution of shaft the work done by a single acting

pump is:-

Since 

V=A

pist

 S,  if the indicator diagram is constructed with volume as the

horizontal axis the 

area of the indicator diagram ( the rectangle 1-2-3-4) is

equal to the work done in one revolution.



The indicator power can be calculated by multiplying 

the area of

the indicator curve

 by the 

speed

 of rotation.



It can also be noted that 

Q’=A

pist

 S n

, hence the 

indicator power 

is

equal to the product of the 

indicator pressure 

and the theoretical

volume flow rate

.

40

B

r

a

k

e

P

o

w

e

r

a

n

d

U

s

e

f

u

l

P

o

w

e

r



The break power that should be delivered from the motor to

the pump hence is,

Where:- 



m

 is the mechanical efficiency



m

 = 0.9 to 0.95

41

The useful power N



Where 



i is 

the internal efficiency which takes care of the

hydraulic loss and leakage losses



h

 = 0.8 to 0.94



vol

 = 0.7 to 0.97



The overall efficiency  is determine by



The coupling power

 is determined by the formula



The efficiency of a piston pump is determined by experiment

42

H

e

a

d

o

f

R

e

c

i

p

r

o

c

a

t

i

n

g

P

u

m

p

s



Therefore the head of a reciprocating pump can be

obtained from the indicator pressure using:-

43

The Actual Indicator Diagram



The main difference between the actual and theoretical

indicator diagrams lies in the 

pressure fluctuations 

in the

beginning of suction and discharge strokes, and the 

effect of

the acceleration head 

which varies with crank angle.



 These fluctuations are caused by the 

effect inertia 

of the valve

and the 

striking of the valves to their seats 

because of the

intimate meeting of ground-in surfaces.



Therefore when the discharge valve is being seated, the

pressure in the valve chest must be raised to a level high

enough to produce a force capable of taking the valve off its

seat and overcoming its inertia.

44



As soon as the valve opens, the pressure in the valve chest falls off

abruptly and 

the valve bobs rapidly up and down

 several times in

the liquid flow, thus 

throttling the flow 

and causing the pressure in

the valve chest to fluctuate, which accordingly affects the

discharge line of the indicator diagram. The actual indicator

diagrams are drawn using readings of indicators connected to

pumps.

45

Cavitations in Reciprocating Pumps



Calculation of the NPSHA should involve the 

acceleration

head

 incase of reciprocating pumps.

Where:-  

ha

 is the acceleration head



As it is already discussed the acceleration head is mostly

much larger than the friction head, hence the 

cavitation

condition 

is usually considered, 

for 



=0

, when the

acceleration head at the suction side is maximum, i.e.

h

as

=h

as,max. 

At this condition, h

fs

=0, and NPSHR=0, since the

velocity of the flow medium at that instant is zero.

46

Since NPSHR=0, no flow

for 



=0

N

o

t

e

:

L

s

i

s

t

h

e

l

e

n

g

t

h

o

f

t

h

e

s

u

c

t

i

o

n

p

i

p

e

t

h

r

o

u

g

h

w

h

i

c

h

t

h

e

r

e

i

s

p

u

l

s

a

t

i

o

n

.

47

Therefore to avoid cavitations,

Performance Characteristics of Reciprocating

Pumps



The performance characteristic of reciprocating pumps is 

quite

different 

from centrifugal pumps. As in the case of centrifugal

pumps, the performance characteristics is commonly described

as a graph. In such cases it is called characteristic curve

Theoretical Performance Characteristics



For a given 

reciprocating pump 

with a given geometry and

speed the 

head does not depend on the theoretical capacity 

and

vice versa. The theoretical capacity, for a single acting single

cylinder-reciprocating pump is given by :-

48

H-Q curves

Constant diameter (D) and stroke length (S), different speeds

•

This curve is especially important in 

flow rate regulation 

by

varying speed. The mean volume flow rate is directly

proportional to the speed.

•

Therefore for three speeds n

1

<n

2

<n

3

, the flow rate becomes:

49

 Constant Diameter (D) and speed (n) and various

strokes (S)



The theoretical performance characteristics for different stroke

lengths are derived in similar fashion and the curves are

similar to those in previous figure.

50

Actual Performance Characteristics



The difference between the actual and theoretical performance

characteristic curves is caused by the dependence of the slip on

head.



The slip of a reciprocating pump increases with the head

against which it operates.

51

5.2

Theory Of Rotary Pumps



Rotary pumps are positive displacement pumps in which

energy is transferred to the flow medium by 

direct application

of force on the boundary of the fluid

, which is defined by the

rotating and stationary elements of the pump.



Like reciprocating pumps the 

amount of fluid 

displaced by

each revolution is 

independent of speed

.



The inlet and outlet ports of rotary fluid machines are

separated by the action and position of the pumping elements

and the close running clearance of the fluid machine. Hence,

unlike reciprocating machines rotary machines 

do not need

suction and discharge 

valves.

52

5.2.1

Operating Principle of Rotary Pumps

•

There are 

three

 distinct parts in the any rotary pump that is in

operation.

•

These parts are defined by the rotating and stationary parts of

the pump and determine the amount of the displaced volume.



 The 

first part 

is defined by the part that is open to the inlet and

is sealed from the outlet.



The 

second 

is the part that is sealed from both the inlet and

outlet.



The 

third

 is the part that is sealed from the inlet but open to the

outlet.

53

•

The three parts are designated as 

OTI 

(Open to inlet

)

, 

CTIO

(Closed to inlet and outlet) 

and 

OTO (Open to outlet

).

•

For a good pumping action the open-to-inlet (OTI)volume

should grow smoothly and continuously with pump rotation

while the open-to-outlet volume (OTO)should reduce

smoothly and continuously. The closed–to-inlet and-outlet

volume should remain constant with pump rotation.

54

Displacement of Common Rotary Pumps

•

The displacement D

of a rotary pump is the total net volume

transferred from the OTI to the OTO volume during one

complete revolution of the driving rotor.

•

For any given pump, the displacement depends only upon the

physical dimensions of the pump 

elements and the pump

geometry 

and 

is independent of other 

operating conditions.

55

D

i

s

p

l

a

c

e

m

e

n

t

o

f

E

x

t

e

r

n

a

l

G

e

a

r

P

u

m

p

s

The displacement D of a gear pump is given by

Where --

D= Displacement,

A=cross-sectional area of tooth space

l=length of gear teeth,

z=number of teeth

56

identical gears

Displacement of Vane Pumps



It is used for the determination of the displacement of vane

pumps.

Figure Vane pump Minimum and Maximum Radii

l

=Total axial length of the rotor

R

1

=Minimum radial dimension of the rotor elements

R

2

=Maximum radial dimension of the rotor elements

57

1.

C

a

p

a

c

i

t

y

o

f

R

o

t

a

r

y

P

u

m

p

s



In general the capacity of any rotary pump is the product of its

displacement (D)

, 

speed of rotation

 of the drive (n) and the

volumetric efficiency 

(



v

).

It is also commonly given as

Where:-

 s = slip of the pump =1-



v

58

1.

P

r

e

s

s

u

r

e

(

H

e

a

d

)

o

f

R

o

t

a

r

y

P

u

m

p

s



Rotary pumps unlike centrifugal pumps 

can deliver

whatever head is required 

by the system.



The only limitations are 

the 

power of the drive 

and the

strength

 of the pump

.



If the drive can deliver sufficient power, yet if the strength of

the pump is low the pump will be damaged.



Hence all positive displacement pumps are commonly, fit

with 

relief valves 

that 

limits the maximum pressure

 inside the

pump.

59

60



Another limiting factor for the maximum pressure is the 

pump

slip.



The pump slip (leakage) in rotary pumps generally increases

with pressure hence running at very high pressure may result in

very low efficiency.



 Note that the total head of the pump is given by:-

Where P

t

 is the total pressure developed by the pump

1.

P

o

w

e

r

o

f

R

o

t

a

r

y

P

u

m

p

s



The useful and brake power of a rotary pump are calculated

from the 

total pressure 

to be transferred to the flow medium,

the 

volume flow rate 

and 

overall efficiency 

of the pump.

Useful Power

The useful power of a rotary pump is the product of the flow

rate and total pressure of the pump (Useful), and is given by,

Brake Power

The brake power is calculated from the useful power

and the overall efficiency using :-

The overall efficiency of rotary pumps is determined by

test

61

5.2.6 Performance Characteristic of Rotary Pumps



The performance characteristics of all positive displacement

pumps are similar and can be applied to rotary pumps also.



 For rotary it is also common to present the curves as

functions of the total pressure:-

62



Note that the capacity curve decreases with pressure.



This is due to the fact that the volumetric efficiency of rotary

pumps in general decreases with the pressure against which the

pump is working.



The limiting pressure P

lim

 represents the pressure above which

there will be 

rapid wear of the pump

.



The pump efficiency drops rapidly and hence the power

consumption ( brake power) of the pump also grows quickly.



 The value of the limiting pressure is adjusted by the setting

point 

(limiting pressure)

 of the 

relief valve

.

63

Methods of Reducing Pulsation

•

Reducing the suction and discharge pulsation is crucial in

installation and operation of reciprocating pumps.

•

By 

reducing the pulsation 

in the suction pipe we increase the

NPSHA available 

and consequently the rpm of the pump can

be increased significantly without fear of cavitation

.

•

In addition to this, 

significant reduction in the power

requirement of the pump and mechanical stability of the pipe

line can be achieved by reducing the pulsation.

64



In a process where uniform discharge is required, either

the discharge pulsation should be reduced or eliminated

somehow or other type of pumps should be used.



The other major problem related to discharge pulsation is

mechanical instability. Due to the non-uniformity of

velocity of the liquid in the cylinder and the discharge

pipe the liquid will decelerate.



This deceleration causes pressure pulsation, which in

some cases cause serious mechanical instability. The

following section discusses the methods for reducing

pulsation in reciprocating pumps.

65

a. Using multiplex pumps



Using multiplex pumps with the 

cylinder connected in parallel

and the 

piston actuated by common cra

nkshaft reduces

pulsation significantly.

Figure 5.6 A multiplex (triplex) reciprocating pump

66

67

Figure 5.7 Reduction of pulsations due to multiple cylinders

Table 5.2 Effect of number of piston/cylinder on variation in capacity

68

b. Air Chambers in the suction and Discharge

lines



Air vessels are closed cylindrical vessels for storing excess

flow. Towards the middle of the stroke, when the velocity of

the flow is greater than the average, the excess flow gets into

the air vessel and compresses the air in the cylinder, building

up a pressure higher than the atmospheric pressure.

69

•

Towards the end and the beginning of the next stroke, when

the velocity is low, the liquid under pressure in the air vessel is

pushed back to the delivery or suction line depending on

whether the stroke is delivery or suction stroke, thus increasing

the velocity there to the average value. The only liquid which

is accelerated is that between the air vessel and the cylinder.

•

When the volume of air in the chamber is large enough, the

flow velocity in the suction pipe is nearly constant. The

suction pulsation in the valve chest is offset by the variable

rate of liquid flow from the air chamber.

70

The Average Volume of Air in The Chamber

•

The amount of air in the air chamber is an important parameter

that determines the uniformity of the flow in the pipe line

section above which it is installed. While the pump is working

the air in chamber gets compressed and expanded and occupies

corresponding volumes, indicated as V

min

 and V

max

respectively (Figure 5.9). When the volume of the air is

minimum its pressure is maximum and vice versa. When the

pump is not working it takes the middle position as indicated

in Figure 5.9.

71

•

The calculation of the average volume of air is based on the excess

volume of liquid that should be handled by the air chamber and the,

and isothermal expansion and compression of the air in the chamber.

As can be seen from Figure 5.9 , the excess volume of liquid that

should be drawing into the chamber and delivered during each cycle

is the difference between V

max

 and V

min

. Therefore,

72

Determining the Excess Volume

•

It is already shown that the capacity of a reciprocating pump

depends on the crank angle. The relation between the crank

angle and the capacity for harmonic motion is equal to the

product of the area of the piston head and the velocity of the

piston head. The velocity of the piston head is given by

Equation 5.8b for harmonic motion.

73

•

The average capacity does not depend on the crank angle and

is given by Equation 5.1 for a single acting single cylinder

reciprocating pumps. Figure 5.10 is a typical representation of

the actual and average volume flow rates as function of time

for one complete rotation, i.e. 



  between 0

0

and 360

0

. The area

under each of the curves represents the total volume to be

delivered by the pump in one complete rotation of the crank

(

one suction and one discharge stroke)

. The two areas should

be equal, since whether the flow is uniform or not the same

amount of liquid is drawn into the pump and is discharged out

in every rotation.

74

•

The shaded area above the average volume flow rate

represents the volume of the liquid which has a flow rate in

excess of the average volume flow rate. The volume of liquid

represented by that area should be stored and delivered by the

air chamber.

75

•

Hence the volume of the liquid to be stored in the chamber can

be computed by drawing the actual and average volume flow

rates on the same scale for a time of one complete rotation, and

determining graphically the area above the average volume

flow rate line 

(shaded area).

76

Calculating the Average Volume of Air in the

Chamber

•

This calculation is based on the assumption that the

compression and expansion in the air chamber takes place at

isothermal condition. Hence,

•

When the air is compressed the pressure is maximum and the

volume is minimum and it is vice versa for expansion.

Applying the above equation,

•

The performance of the air chamber is characterized by the

degree of irregularity which is defined by

77

•

Where: P

av

 is the average air pressure in the chamber, given by

78

•

The average volume of air in the chamber can be

determined for a predetermined degree of irregularity.

Note it is earlier discussed how to determine the

excess volume graphically. This procedure is used

both for suction and discharge air chambers. The

commonly accepted degrees of irregularity are

•

For Suction Air Chambers



 0.02

•

For Discharge Air Chambers

0.04





 0.05

79