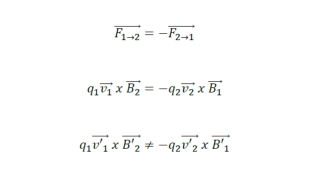Tailoring Strong Lensing Cosmography Observations
The exploration of cosmography involves mapping the general features of the universe to understand its large-scale geometry and kinematics. This study delves into measuring time delay distances, optimizing spectroscopic follow-up, and addressing systematics such as redshift distribution and model systematics in strong gravitational lensing. The time delay distance, a crucial aspect, is influenced by dark energy properties and complements traditional distance probes, making it an intriguing area for research and observations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Eric V. Linder (arXiv: 1502.01353v1) Tailoring Tailoring Strong Lensing Cosmographic Strong Lensing Cosmographic Observations Observations
Contents Introduction I. II. Measuring time delay distances III. Optimizing Spectroscopic followup IV. Influence of systematics A. Redshift distribution revisited B. Model systematics V. Conclusion
I. Introduction What is the Cosmography ? The science that maps the general features of the cosmos or universe, describing both heaven and Earth beginning to be used to describe attempts to determine the large-scale geometry and kinematics of the observable universe, independent of any specific cosmological theory or model
1. Introduction Cosmography Distance redshift relations Type Ia supernova luminosity distance - redshift relation The cosmic microwave background radiation anisotropies/ baryon acoustic oscillations in galaxy clustering Cosmic redshift drift The strong gravitational lensing time delay distance - redshift relation (1964, Refsdal)
1. Introduction Strong lensing distance Why interesting? unlike the standard distance-redshift relations the measured time delay is a dimensionful quantity the time delay distance is comprised of the ratio of three distances sensitive to the Hubble constant H0 the time delay distance has an unusual dependence on dark energy properties and has high complementarity with the usual distance probes On-going & future surveys: DES, LSST, Euclid, WFIRST
1. Introduction Two aspects of implementation of time delay distances into surveys 1. Optimization of spectroscopic resources 2. The role of systematics
II. Measuring time delay distances Time delay between two images of the source come from: The geometric path difference of the light propagation The differing gravitational potential experienced The time delay distance: t : the observed time delay : the potential difference modeled from the observations such as image position, fluxes, surface brightness
II. Measuring time delay distances The time delay distance: (surveys: DES, LSST, Euclid, WFIRST) t : the observed time delay By monitoring the image fluxes over several years : the potential difference modeled from the observations such as image position, fluxes, surface brightness constrained by the rich data of the images (HST, JWST) lens mass modeling : galaxy velocity dispersion by through spectroscopy Redshift of lens and sources : spectroscopy
II. Measuring time delay distances For t and , These essential followup must be sought in order to derive the strong lensing cosmological constraints from the wide field imaging survey (limitation on telescope time) The optimization of cosmological leverage given a finite followup resources Combine the strong lesing distances with CMB and supernovae distances to break degeneracies between parameters
II. Measuring time delay distances Etc. Combine the strong lesing distances with CMB and supernovae distances to break degeneracies between parameters Adopt a Planck quality constraint on the distance to last scattering (0.2%) and physical matter density (0.9%) For supernovae, use a sample of the quality expected from ground based surveys Perform a Fisher information analysis for ( m, w0, wa, h, ) with flat LCMD cosmology
III. Optimizing Spectroscopic Followup Spectroscopic time is restricted Optimization: maximize the cosmological leverage of the measured time delay distance given the constraint, fixed this (= limited source) by examining the impact of sculpting the redshift distribution of the lenses to be followed up especially, fix the spectroscopic time for the sample of lenses whose redshift or galaxy velocity dispersion are to be measured with fixed signal-to-noise
III. Optimizing Spectroscopic Followup Spectroscopic time for lenses is restricted fixed signal-to-noise gives Spectroscopic exposure time becomes increasingly expensive with redshift as roughly (1+z)^6 However, as exposure time gets smaller, other noise contributions enter as well as overheads (telescope slewing and detector readout time)
III. Optimizing Spectroscopic Followup Spectroscopic time for lenses is restricted Optimizing procesure Fix signal-to-noise to obtain constraint on the exposure time, t_exp Choose the quantity to optimize: dark energy figure of merit (FOM), the area of a confidence contour in the dark energy equation of state plane, marginalized over all other parameters. To optimize the redshift distribution, begin with a uniform distribution in lens redshift
III. Optimizing Spectroscopic Followup Spectroscopic time for lenses is restricted Optimizing procesure Fix signal-to-noise to obtain constraint on the exposure time, t_exp Choose the quantity to optimize: dark energy figure of merit (FOM) To optimize the redshift distribution, begin with a uniform distribution in lens redshift Take 25 time delay systems of 5% precision in each bin of redshift width dz = 0.1 over the range z = 0.1~0.7, for a total of 150 systems (fixed resource constraint, total spectroscopic time)
III. Optimizing Spectroscopic Followup Spectroscopic time for lenses is restricted Optimizing procesure Fixe signal-to-noise to obtain constraint on the exposure time, t_exp Choose the quantity to optimize: dark energy figure of merit (FOM) Begin with a uniform distribution in lens redshift Perturb the initial uniform distribution by one system in each bin, one at a time (conserve the resources) Calculate resulting FOM Iterate the last two processes Round the numbers in each bin to the nearest integer
III. Optimizing Spectroscopic Followup Optimization increases the FOM by almost 40%, keeping the spectroscopic time fixed. Optimized redshift dist. Heavily weighted toward low redshift (less time burden) The higher redshift bin is needed, but it does not seek to maximize the range by taking the highest bin (greatest time burden) FOM becomes improved Parameter estimation
IV. Influence of Systematics Dealing with systematic uncertainties Investigate two impact of systematics A. Redshift distribution revisited B. Model systematics
IV. Influence of Systematics A. Redshift distribution revisited The effect of various levels of systematic uncertainties on the optimization in III. On the optimized redshift distribution and the resulting cosmological parameter estimation. Implementation of the systematic as a floor, added in quadrature to the statistical uncertainty: ni: the number in redshift bin i
IV. Influence of Systematics A. Redshift distribution revisited Optimized lens redshift distribution, subject to resource constraint, for different levels of systematics
IV. Influence of Systematics A. Redshift distribution revisited Optimized lens redshift distribution, subject to resource constraint, for different levels of systematics
IV. Influence of Systematics A. Redshift distribution revisited Optimized lens redshift distribution, subject to resource constraint, for different levels of systematics reduced hubble constant, h
V. Conclusion The strong gravitational lensing time delay distance redshift relation : a geometric probe of cosmology Being dimensionful and hence sensitive to the Hubble constant, H0 Being a triple distance ratio and hence highly complementary to other distance probes
V. Conclusion The strong gravitational lensing time delay distance redshift relation : a geometric probe of cosmology Optimization of the lens system redshift distribution to give maximal cosmology leverage, given followup resources do improve the result (FOM, parameter estimation, etc)

 undefined
undefined