Special Channels in Information Theory
Explore different types of special channels in information theory, including lossless, deterministic, noiseless, and extremely noisy channels, each with unique characteristics affecting entropy and information transmission. See visual channel models for better understanding.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
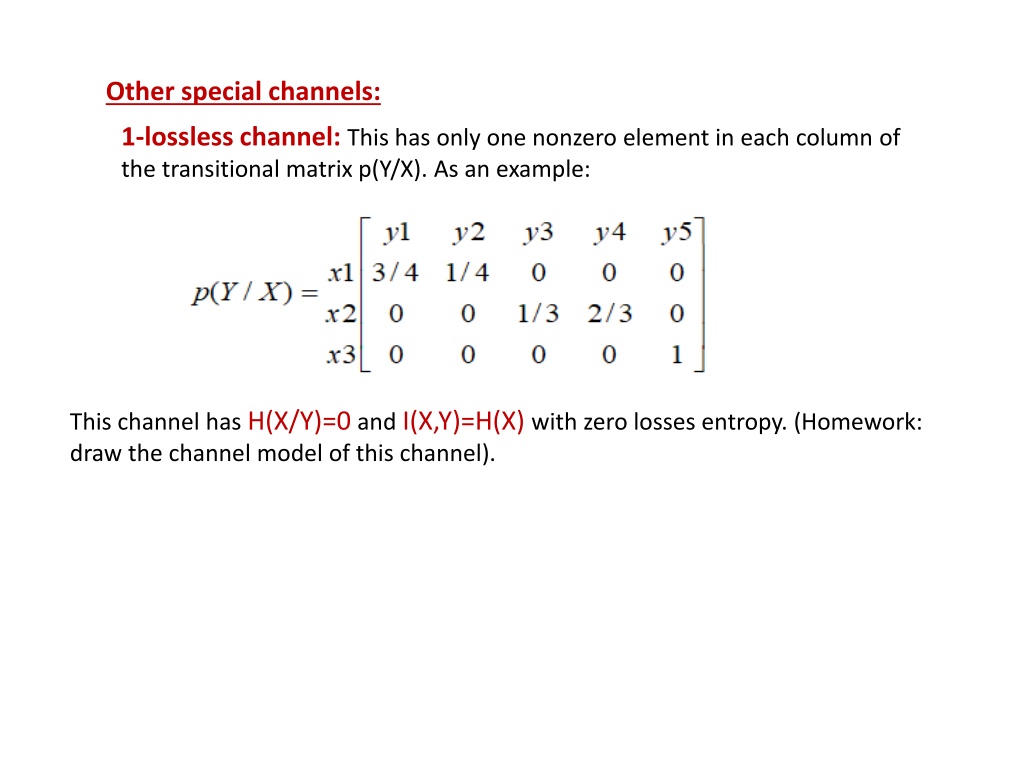
Other special channels: 1-lossless channel: This has only one nonzero element in each column of the transitional matrix p(Y/X). As an example: This channel has H(X/Y)=0 and I(X,Y)=H(X) with zero losses entropy. (Homework: draw the channel model of this channel).
2-Determinstic channel: This has only one nonzero element in each row of the transitional matrix p(Y/X). As an example: This has H(Y/X)=0 and I(X,Y)=H(Y) with zero noise entropy. (Homework: draw the channel model of this channel).
3-Noiseless channel: This has only one nonzero element in each row and column of the transitional matrix p(Y/X), i.e. it is an identity matrix. As an example: This has H(X/Y)=H(Y/X)=0, and I(X,Y)=H(X)=H(Y). (Homework: draw the channel model of this channel).
3- extremely noisy channel: xi and yj are statistically independent, so I(X,Y) =0