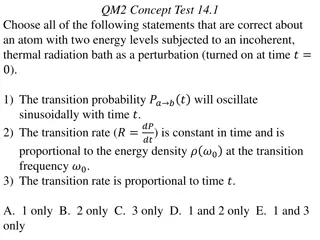
Evolution of Perturbations in Decaying Dark Matter Models
The study discusses the evolution of perturbations and cosmological constraints in decaying dark matter models, focusing on the impact on ClTT, large-scale structure, and small-scale discrepancies. Mechanisms to address these issues, such as decaying dark matter, are explored in detail. The model considered involves dark matter decay into two product particles, with parameters characterized to analyze density perturbations and other cosmological effects.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
KIAS workshop (2014) 3rdNovember, 2014 Evolution of perturbations and cosmological constraints in decaying dark matter models with arbitrary decay mass products Shohei Aoyama Nagoya University Collaborators: Toyokazu Sekiguchi, Kiyotomo Ichiki & Naoshi Sugiyama JCAP07(2014)021, arXiv: 1402.2972
CDM and its problem The CDM model can predict numerous cosmological observations on large scales about temperature anisotropy of the CMB ClTT and the large scale structure. http://cdn.arstechnica.net/wp- content/uploads/2013/03/Planck_cosmic_recipe.png However the nature of dark matter (eg. the mass or interactions ) is still unknown. In addition, observations on the small scale, the discrepancies between the observations and the theoretical predictions. (eg. missing satellite problem) Moore et al. (1999) [astro-ph/9907411]
Planck anomaly Planck collaborations pointed out that 8 which is estimated from ClTT is larger than that from the number counts of cluster by SZ effect on CMB by more than 2 CL. Planck Collaboration(2013,XX)[1303.5080]
A prescription of these problems Some mechanisms that suppress small scale structure formation may rescue these problems. (Ostriker & Steinhardt (2003) [astro-ph/0306402]). Decaying dark matter (DDM) is a candidate of these problems because product particles are generated with large momentum and smear out of the gravitational potential which was originated by progenitor particles. Moore+ (1999) [astro-ph/9907411] Planck Collaboration(XX)[1303.5080]
Our model of DDM We consider the DDM which decays into two product dark matter particles. In this case, the decaying process and the time evolutions of distribution functions of dark matter particles can be characterized by 4 parameters. In our calculation, by solving Boltzmann equations for these product particles directly, one can take the mass of one of daughter particles arbitrary and obtain the time evolutions of density perturbations, ClTTand 8.
The effect on ClTT peak shift integrated SW
Our model of DDM We consider the DDM which decays into two product dark matter particles. In this case, the decaying process and the time evolutions of distribution functions of dark matter particles can be characterized by 4 parameters. In this work, we set the constraint on the lifetime of DDM from 8 estimated from BOSS III data. 8=0.80 0.02 S nchez et al.(2012)[arXiv: 1203.6616])
The constraint on the lifetime of DDM This SDSS data excluded 8< 0.76 on 2 CL. 2 anomaly The lifetime of the DDM should be longer than 200Gyr in the case relativistic decay. This constraint is consistent with previous works (Ichiki et al. (2004), [astro-ph:astro-ph/0403164]).
Planck anomaly Favored region The DDM whose lifetime of DDM is around 200 Gyr and which decays into two relativistic particles can explain observational ClTTand the number of clusters.
The power spectrum of CMB lens potential Cl mD1/mM=0.3 mD1/mM=0.9 CDM The DDM which decays into two relativistic particles is not ruled out from the current CMB lensing observation.
Conclusion We consider the decaying dark matter which decays two particles and its effect on the cosmological observables. Even in the case that the finite mass of one of product particles, the lifetime should be larger than 200 Gyr. DDM may reconcile the tension between the observed 8estimated from ClTT and that from number counts of cluster from SZ effect.