Limits on Dark Energy Using Atom Interferometry - UC Berkeley Study
Atom-interferometry
limits on dark energy
Jun. 17. 2011
Geena Kim
P. Hamilton, D. Schlippe, and H. Mueller
University of California, Berkeley
Paul Hamilton
Müller group
University of California at
Berkeley
1.
Screened scalar fields as dark energy
2.
Atom interferometry search for dark energy
•
P. Hamilton, M. Jaffe, P. Haslinger, Q. Simmons, H. Müller, J.
Khoury arXiv:1502.03888
3.
Future reach with atom interferometry
•
Eliminate theories with coupling up to Planck mass
Outline
Evidence
ESA/Planck
SDSS
SCP
+
+
=
Known unknowns
D
a
r
k
e
n
e
r
g
y
d
e
n
s
i
t
y
~
1
h
y
d
r
o
g
e
n
a
t
o
m
/
m
3
o
r
a
n
e
n
e
r
g
y
s
c
a
l
e
o
f
2
.
4
m
e
V
ESA/Planck
Dark energy sources
•
Cosmological constant
•
New energy scale = new field?
•
Scalar fields advantages
•
Can explain why cosmic acceleration started now
•
Allow for equations of state with w ≠ -1
Scalar dark energy
Simple scalar models lead to equivalence principle
violations in conflict with solar system tests and
fifth force searches.
What if scalar field effects are somehow reduced in
normal matter?
Only two ingredients needed for screening
1)
Scalar field self-potential
2)
Coupling to local matter density
Chameleon fields
K
h
o
u
r
y
,
W
e
l
t
m
a
n
P
h
y
s
.
R
e
v
.
D
6
9
,
0
4
4
0
2
6
The “chameleon” as a model screened scalar field
Chameleon fields
K
h
o
u
r
y
,
W
e
l
t
m
a
n
P
h
y
s
.
R
e
v
.
D
6
9
,
0
4
4
0
2
6
Normal matter
In vacuum
Coupling to
local density
Self-potential
Chameleon screening
Unscreened
Screened
Only a thin shell
contributes in
macroscopic objects
1 cm
10 nm
Chameleon field acts as a potential for objects
Screened force
Public outreach
B
u
r
r
a
g
e
,
C
o
p
e
l
a
n
d
,
H
i
n
d
s
a
r
X
i
v
:
1
4
0
8
.
1
4
0
9
Realization:
Single atom’s small size makes it ideal
test mass which evades screening
Semi-famous internet meme
Screened force
Atom interferometry
Detection
•
Optically push one state to side before imaging
•
Fluorescence detection of two output states
•
Sphere moved in and out with translation stage
3 mm
Cavity interferometer
•
4 lasers, 2 optical cavities
•
7 frequency and phase locks
For more information:
Gravimetry fringes
Reversed interferometers
Alternate momentum kick directions to
reduce systematics
Results
Red = sphere near
Blue = sphere far
Difference between
sphere near/far
Systematics
Differential measurement
How else can the sphere affect our measurement?
•
Changes in interferometry laser
•
Check power dependence
•
Magnetic fields
•
Increase bias field x10
•
Electric fields
•
Negligible due to small polarizability
Constraints on parameters
Atom interferometry
Dark energy limits
Photon coupling comparison
Limits including experiments using an additional
coupling to the photon
CAST- arxiv:1503.04561
Atom
interferometry
Atom interferometry does not need photon coupling
The future
3-4 orders of magnitude improvement
reaches Planck Mass couplings
The future
Atom interferometry can help constrain many scalar dark energy theories
“Force-Free Gravitational Redshift: Proposed Gravitational Aharonov-Bohm Experiment”
M
.
H
o
h
e
n
s
e
e
,
B
.
E
s
t
e
y
,
P
.
H
a
m
i
l
t
o
n
,
A
.
Z
e
i
l
i
n
g
e
r
,
H
.
M
ü
l
l
e
r
,
P
R
L
1
0
8
,
2
3
0
4
0
4
(
2
0
1
2
)
x
[m]
Optical lattice
Field mass
Gravitational Aharonov-Bohm Effect
See poster by Matt Jaffe
D
a
r
k
e
n
e
r
g
y
/
o
p
t
i
c
a
l
c
a
v
i
t
y
i
n
t
e
r
f
e
r
o
m
e
t
e
r
Paul Hamilton
Philipp Haslinger
Matt Jaffe
Justin Khoury
Quinn Simmons
A
n
t
i
h
y
d
r
o
g
e
n
i
n
t
e
r
f
e
r
o
m
e
t
e
r
Paul Hamilton
Philipp Haslinger
ALPHA collaborators
Andrey Zhmoginov
Joel Fajans
Jonathan Wurtele
X
U
V
a
t
o
m
i
n
t
e
r
f
e
r
o
m
e
t
e
r
Paul Hamilton
Collaborators
Birgitta Whaley
Ali Belkacem
P
I
:
H
o
l
g
e
r
M
ü
l
l
e
r
L
i
t
h
i
u
m
a
t
o
m
i
n
t
e
r
f
e
r
o
m
e
t
e
r
Kayleigh Cassell
Eric Copenhaver
Paul Hamilton
T
a
l
k
t
o
m
o
r
r
o
w
P
o
s
t
e
r
O
t
h
e
r
p
r
o
j
e
c
t
s
:
Systematics - Magnetic
Systematics – AC Stark
Systematics - All
F=3 atoms
F=4 atoms
Recent Mach-Zehnder data
F=4 /
(F=3 + F=4)
Chameleon mass
Atom screening
Mass screening
Exclusion plot regions
Strong coupling
Lorentz invariance requires
•
Holds exactly in all of QM and QFT
Phase of a quantum state
[de Broglie, 1924, Ph.D thesis (!)]
An atom interferometer measures the phase difference:
(for Mach – Zehnder)
Compton frequency
Time dilation
Redshift
Light pulse atom interferometer
Beamsplitter
Interferometer
Demonstrated sensitivity
of atom interferometers
•
Accelerations: ~ppb !
•
Rotations: <nrad/s !
Cavity parameters: L = 40.76 cm,
waist~600
μ
m, finesse~200
Cavity interferometer
apparatus
Cavity lock
laser
Interferometry
laser
•
2D magneto-optical source
•
3D magneto-optical trap
•
Molasses cooling
•
Optical lattice load
•
Adiabatic release
•
Optical pumping
•
State and spatial selection
•
Velocity selection
•
Interferometer sequence
•
Fluorescence detection
Experimental sequence
Which requires stable operation of:
•
4 lasers, 2 optical cavities
•
7 frequency and phase locks
Research conducted by Paul Hamilton Müller's group at the University of California, Berkeley, focuses on using atom interferometry to explore dark energy. The study delves into screened scalar fields as dark energy, future reach with atom interferometry, known unknowns related to dark energy density, and sources of dark energy such as cosmological constants and scalar fields. It also discusses chameleon fields as a model for screened scalar fields and the concept of chameleon screening. The study raises questions about scalar dark energy and proposes theories to test and further understand dark energy in the universe.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Atom-interferometry limits on dark energy Paul Hamilton M ller group University of California at Berkeley Jun. 17. 2011 Geena Kim P. Hamilton, D. Schlippe, and H. Mueller University of California, Berkeley
Outline 1. Screened scalar fields as dark energy 2. Atom interferometry search for dark energy P. Hamilton, M. Jaffe, P. Haslinger, Q. Simmons, H. M ller, J. Khoury arXiv:1502.03888 3. Future reach with atom interferometry Eliminate theories with coupling up to Planck mass
Evidence + = SCP + SDSS ESA/Planck
Known unknowns ESA/Planck Dark energy density ~1 hydrogen atom / m3 or an energy scale of 2.4 meV
Dark energy sources Cosmological constant New energy scale = new field? Scalar fields advantages Can explain why cosmic acceleration started now Allow for equations of state with w -1
Scalar dark energy Simple scalar models lead to equivalence principle violations in conflict with solar system tests and fifth force searches. What if scalar field effects are somehow reduced in normal matter? Only two ingredients needed for screening 1) Scalar field self-potential 2) Coupling to local matter density
Chameleon fields The chameleon as a model screened scalar field Veff= 4+ 4+? ??+? ?? Self-potential Coupling to local density Low density ? vacuum High density ? normal matter Khoury, Weltman Phys. Rev. D 69, 044026
Chameleon fields Low mass Long range High mass Short range Coupling to local density Self-potential In vacuum Normal matter Khoury, Weltman Phys. Rev. D 69, 044026
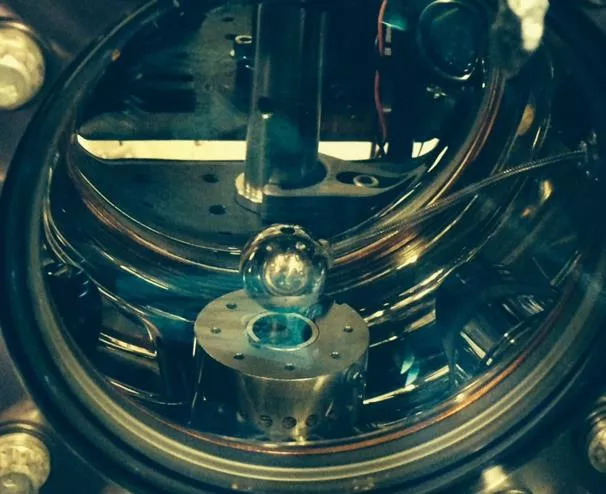
Chameleon screening Screened 10 nm Unscreened Only a thin shell contributes in macroscopic objects 1 cm For = 2.4 meV, M = 10-5 MPl Al sphere in 10-10 Torr vacuum Chameleon field acts as a potential for objects
Screened force 2 ?? ???????=????? ??? ? ? ?? ??? 1 + 2 ???? ?2 ? =? ??? ???? ???? ???? Can be extremely small ( 10-20) for macroscopic objects Unscreened force can be much stronger than gravity ? < ???
Public outreach Burrage, Copeland, Hinds arXiv:1408.1409 Realization: Semi-famous internet meme Single atom s small size makes it ideal test mass which evades screening
Screened force 2 ?? ???????=????? ??? ? 1 + 2 ???? ?2 ? =? ??? ???? ???? ???? ?????= 1 For most of parameter space Can be extremely small ( 10-20) for macroscopic objects Unscreened force can be much stronger than gravity ? < ???
Atom interferometry Height 0 T 2T Time Aluminum sphere source mass for scalar field Atoms act as test masses for force sensing Final state probability ? ? ?2
Detection 3 mm Optically push one state to side before imaging Fluorescence detection of two output states Sphere moved in and out with translation stage
Cavity interferometer Upper Mirror 3D MOT 2D MOT Lower Mirror 4 lasers, 2 optical cavities 7 frequency and phase locks For more information:
Gravimetry fringes Interferometer phase depends on acceleration ? and photon momentum ? Height Time Lower state probability Cos2? ? ?2
Reversed interferometers Alternate momentum kick directions to reduce systematics Kick up + ? Kick down ? Height Time Interferometer phase ? ? ?2+ ?????
Results Red = sphere near Blue = sphere far Difference between sphere near/far ? = 2.3 3.3 m/s2
Systematics Differential measurement How else can the sphere affect our measurement? Changes in interferometry laser Check power dependence Magnetic fields Increase bias field x10 Electric fields Negligible due to small polarizability Final result: 8.2 m/s2< ? < 6.7 m/s2 (95% confidence) For attractive force: ? < 5.5 m/s2 (95% confidence)
Constraints on parameters Atom interferometry Neutrons = Dark energy screened ? < 6.6 10 5??? unscreened n=1 n=5 Veff= 4+ 4+? ??+? ??
Dark energy limits Veff= 4+ 4+? Limits at = 2.4 meV versus power law exponent, n, of the chameleon potential ??+? ??
Photon coupling comparison Atom interferometry CAST- arxiv:1503.04561 Limits including experiments using an additional coupling to the photon Atom interferometry does not need photon coupling
The future Current status: Atomic shot noise limit is < 1 m/s2 / s Vibration noise is ~50x higher Improvements: Vibration isolation 10 Launch atoms 4 20 ? Bragg beamsplitters 10 Improve stability, integrate longer 10 Two cloud gradiometer 5 Lattice interferometry 3-4 orders of magnitude improvement reaches Planck Mass couplings
The future Model Description Chameleon Mass couples to matter density Symmetron Coupling depends on matter density f(R) gravity Equivalent to chameleon theories with coupling of 1 6??? Maps to chameleon theory Preferred scale Varying dilaton Time varying equivalence principle violation Topological DM Anomalous forces K-mouflage Pressuron Galileon Atom interferometry can help constrain many scalar dark energy theories
Gravitational Aharonov-Bohm Effect Field mass Optical lattice 0.35 0.30 U C 0.25 2 c 0.20 0.15 [rad/s] 0.10 0.05 0.02 0.01 0.00 x [m] 0.01 0.02 U 2 = = = UT / S dt m C c Force-Free Gravitational Redshift: Proposed Gravitational Aharonov-Bohm Experiment M. Hohensee, B. Estey, P. Hamilton, A. Zeilinger, H. M ller, PRL 108, 230404 (2012)
Other projects: The chameleonaires Lithium atom interferometer Kayleigh Cassell Eric Copenhaver Paul Hamilton Justin Khoury Matt JaffePSH XUV atom interferometer Paul Hamilton Collaborators Birgitta Whaley Ali Belkacem Philipp Haslinger Holger M ller Dark energy / optical cavity interferometer Antihydrogen interferometer Paul Hamilton Philipp Haslinger ALPHA collaborators Andrey Zhmoginov Joel Fajans Jonathan Wurtele Paul Hamilton Philipp Haslinger Matt Jaffe Justin Khoury Quinn Simmons Talk tomorrow Poster PI : Holger M ller
Recent Mach-Zehnder data F=4 / (F=3 + F=4) F=3 atoms F=4 atoms
Phase of a quantum state = = 2 E mc [de Broglie, 1924, Ph.D thesis (!)] C Lorentz invariance requires ( ) C i ~ exp Holds exactly in all of QM and QFT An atom interferometer measures the phase difference: Compton frequency Time dilation Redshift ) ( 2 v U = + 2 mc dt 2 2 laser 2 c c = 2 k g T (for Mach Zehnder)
Light pulse atom interferometer Beamsplitter Interferometer ? ? ? ? ? |?,? |?,? + 2 ? Height k k |?,? |?,? + 2 ? |?,? + 2 ? |?,? 0 T 2T Time Demonstrated sensitivity of atom interferometers = + L d laser Accelerations: ~ppb ! Rotations: <nrad/s ! = 2 k g T
Cavity interferometer apparatus Cavity mirror Upper Mirror 3D MOT p=2n k 2D MOT Magnetic Shields Cavity mirror Lower Mirror Cavity lock laser Interferometry laser Cavity parameters: L = 40.76 cm, waist~600 m, finesse~200
Experimental sequence 2D magneto-optical source 3D magneto-optical trap Molasses cooling Optical lattice load Adiabatic release Optical pumping State and spatial selection Velocity selection Interferometer sequence Which requires stable operation of: Fluorescence detection 4 lasers, 2 optical cavities 7 frequency and phase locks
