Introduction to Chemistry: Matter, Energy, and Measurement
Chemistry is the study of matter, its properties, and the changes it undergoes. This content covers the basics of chemistry, including the classification of matter into pure substances and mixtures, different states of matter, and the building blocks of matter such as atoms and molecules. Sample exercises and practice questions are provided to reinforce learning.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
AP Chapter 1- Introduction: Matter, Energy, and Measurement Jennie L. Borders
Section 1.1 - The Study of Chemistry Chemistry is the study of matter, its properties, and the changes that matter undergoes. Matter is anything that has mass and occupies space. Atoms are the building blocks of matter. Elements are made of one type of atom. Molecules are two or more atoms that are joined together by bonds.
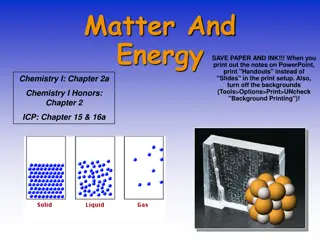
Section 1.2 Classifications of Matter States of Matter A gas has no fixed shape or volume and can easily by compressed. The particles are far apart. Ex: O2gas A vapor is the gaseous state of matter of a substance that is normally a solid or liquid at room temperature. Ex: H2Ovapor A liquid has an indefinite shape but a definite volume. The particles are close together but can flow. A solid has a definite shape and volume. The particles are close together and orderly since they vibrate in fixed locations.
Pure Substances A pure substance has a constant composition. Pure substances can be elements or compounds. An element is made of one type of atom. Elements can be composed of atoms or molecules. A compound is composed of two or more elements bonded together in a fixed proportion. Compounds can be broken down into elements by chemical processes.
Mixtures A mixture has a variable composition. Homogeneous mixtures are variable but APPEAR uniform throughout. They are also called solutions. An example is Windex. Heterogeneous mixtures have a variable composition in which the different parts are noticeable. An example is Italian salad dressing. Mixtures can be made of solids, liquids, or gases.
Sample Exercise 1.1 White gold contains gold and a white metal, such as palladium. Two samples of white gold differ in the relative amounts of gold and palladium they contain. Both samples are uniform in composition throughout. Use the chart to classify white gold.
Practice Exercise 1 Which of the following is the correct description of the inside of a grapefruit? a. It is a pure compound. b. It consists of a homogeneous mixture of compounds. c. It consists of a heterogeneous mixture of compounds. d. It consists of a heterogeneous mixture of elements and compounds. e. It consists of a singe compound in different states.
Practice Exercise 2 Aspirin is composed of 60.0% carbon, 4.5% hydrogen, and 35.5% oxygen by mass, regardless of its source. Use the chart to classify aspirin.
Section 1.3 Properties of Matter Physical properties can be observed without changing the composition of a substance. Ex. Melting point Chemical properties describe the way a substance may react or change composition. Ex. Flammability Intensive properties do not depend on the amount of substance only the type of substance. Ex. Density Extensive properties depend on the amount of a sample. Ex. mass
Physical and Chemical Changes A physical change occurs when properties of a substance change but the composition remains the same. Ex. Boiling water A chemical change occurs when the composition of a substance changes. Ex. Burning wood
Separating Mixtures Distillation separates mixtures based on their different boiling points. Filtration separates mixtures based on particle size. Chromatography separates mixtures based on the differing abilities of substances to adhere to a surface.
Section 1.4 The Nature of Energy Energy is the capacity to do work or transfer heat. Work is the energy transferred when a force exerted on an object causes the object to move in the same direction as the force. Force is a push or pull on an object. Heat is the energy used to cause the temperature of an object to increase.
Kinetic and Potential Energy Kinetic energy is the energy of motion. It depends on mass and velocity. Atoms and molecules have kinetic energy which increases as a substance is heated. Potential energy is stored energy due to a position of an object. Electrostatic potential energy arises from the interaction of charged particles. Opposite charges attract and like charges repel.
Section 1.5 Units of Measurement Quantitative properties are associated with numbers. SI units are the preferred units for science.
Unit Prefixes Make sure that you remember how to convert between units with different prefixes using this chart.
Sample Exercise 1.2 What is the name of the unit that equals a. 10-9gram b. 10-6 second c. 10-3meter
Practice Exercise 1 Which of the following weights would you expect to be suitable for weighing on an ordinary bathroom scale? a. 2.0 x 107mg b. 2500 mg c. 5 x 10-4kg d. 4 x 106cg e. 5.5 x 108dg
Practice Exercise 2 a. How many picometers are the in 1 m? b. Express 6.0 x 103m using a metric prefix to replace the power of ten. c. Use exponential notation to express 4.22 mg in grams. d. Use decimal notation to express 4.22 mg in grams.
Temperature Temperature is the measure of the average kinetic energy of the particles in a substance. Absolute zero (0K) is the temperature which the motion of particles stops. The size of a degree in Celsius is the same as the size of a degree in Kelvin. A degree in Fahrenheit is smaller. K = oC + 273 oC = 5(oF 32) 9 5 oF = 9(oC) + 32
Sample Exercise 1.3 A weather forecaster predicts the temperature will reach 31oC. What is this temperature a. in K? b. in oF?
Practice Exercise 1 Ethylene glycol, the major ingredient in antifreeze, freezes at -11.5 oC. What is the freezing point in a. K? b. oF?
Density and Energy Density is the amount of mass in a unit volume of a substance. D = m v The SI unit for energy is the Joule. (1 J = 1 kg.m2/s2) A calorie is the amount of energy required to raise 1 gram of water 1oC. 1 cal = 4.184 J 1 Cal = 1000 cal
Sample Exercise 1.4 a. Calculate the density of mercury if 1.00 x 102 g occupies a volume of 7.36 cm3. b. Calculate the volume of 65.0 g of liquid methanol if its density is 0.791 g/mL. c. What is the mass in grams of a cube of gold (density = 19.32 g/cm3) if the length of the cube is 2.00 cm?
Practice Exercise 1 a. Calculate the density of a 374.5 g sample of copper if it has a volume of 41.8 cm3. b. A student needs 15.0 g of ethanol for an experiment. If the density of ethanol is 0.789 g/mL, how many milliliters of ethanol are needed? c. What is the mass, if grams, of 25.0 mL of mercury (density = 13.6 g/mL)?
Sample Exercise 1.5 A standard propane (C3H8) tank used in an outdoor grill holds approximately 9.0 kg of propane. When the grill is operating, propane reacts with oxygen to form carbon dioxide and water. For every gram of propane that reacts with oxygen in this way, 46 kJ of energy is released as heat. a. How much energy is released if the entire contents of the propane tank react with oxygen? b. As the propane reacts, does the potential energy stored in chemical bonds increase or decrease?
Practice Exercise 1 A 12-oz vanilla milkshake at a fast food restaurant contains 547 Calories. What quantity of energy is this in joules?
Section 1.6 Uncertainty of Measurement Accuracy refers to how closely an individual measurement agrees with the correct value. Precision is how close multiple measurements are to each other.
Significant Figures All digits 1-9 are significant. Front zeros (to the left of the significant figures) are NOT significant. Middle zeros are significant. Back zeros (to the right of the significant figures) are only significant if you can see the decimal point. Numbers that are counted and conversion factors contain infinite significant figures.
Sample Exercise 1.7 How many significant figures are in each of the following numbers (assume that each number is a measured quantity)? a. 4.003 b. 6.023 x 1023 c. 5000
Practice Exercise 1 An object is determined to have a mass of 0.01080 g. How many significant figures are there in this measurement? a. 2 b. 3 c. 4 d. 5 e. 6
Practice Exercise 2 How many significant figures are in each of the following measurements? a. 3.549 g b. 2.3 x 104 cm c. 0.00134 m3
Significant Figures in Calculations For adding and subtracting, your answer must have the same amount of decimal places as the measurement with the least amount of decimal places. For multiplying and dividing, your answer must have the same amount of significant figures as the measurement with the least amount of significant figures.
Sample Exercise 1.8 The width, length, and height of a small box are 15.5, 27.3, and 5.4 cm, respectively. Calculate the volume of the box, using the correct number of significant figures in your answer.
Practice Exercise 1 Ellen recently purchased a new hybrid car and wants to check her gas mileage. At an odometer setting of 651.1 mi, she fills the tank. At 1314.4 mi, she requires 16.1 gal to refill the tank. Assuming that the tank is filled to the same level both times, how is the gas mileage best expressed? a. 40 mi/gal b. 41 mi/gal c. 41.2 mi/gal d. 41.20 mi/gal
Practice Exercise 2 It takes 10.5 s for a sprinter to run 100.00 m. Calculate her average speed in meters per second and express the result to the correct number of significant figures.
Sample Exercise 1.9 A vessel containing a gas at 25oC is weighed, emptied, and then reweighed as depicted in the figure. From the data provided, calculate the density of the gas at 25oC.
Practice Exercise 1 You are asked to determine the mass of a piece of copper using its reported density, 8.96 g/mL, and a 150-mL graduated cylinder. First, you add 105 mL of water to the graduated cylinder; then you place the piece of copper in the cylinder and record a volume of 137 mL. What is the mass of the copper reported with the correct number of significant figures? a. 287 g b. 3.5 x 10-3 g/mL c. 286.72 g/mL d. 3.48 x 10-3 g/mL e. 2.9 x 102 g/mL
Practice Exercise 2 If the mass of the container in the sample exercise were measured to three decimal places before and after pumping out the gas, could the density of the gas then be reported to four significant figures?
Section 1.7 Dimensional Analysis Dimensional analysis is using different units to calculate a quantity. A conversion factor is a fraction whose numerator and denominator are the same quantity expressed in different units. Follow the golden rule every time.
Sample Exercise 1.10 If a woman has a mass of 115 lbs, what is her mass in grams? (Use the relationship between units given on the back inside cover or the textbook or remember the conversion factor from Honors Chemistry, 1 kg = 2.2 lbs.)
Practice Exercise 1 At a particular instant in time, the Earth is judged to be 92,955,000 miles from the Sun. What is the distance in kilometers to four significant figures? (1 km = 0.62137 mi) a. 5763 x 104 km b. 1.496 x 108 km c. 1.49596 x 108 km d. 1.483 x 104 km e. 57,759,000 km
Sample Exercise 1.11 The average speed of a nitrogen molecule in air at 25oC is 515 m/s. Convert this speed to miles per hour. (1 km = 0.62137 mi)
Practice Exercise 1 Fabiola, who lives in Mexico City, fills her car with gas, paying 357 pesos for 40.0 L. What is her fuel cost in dollars per gallon, if 1 peso = 0.0759 dollars? (1 gal = 3.7854 L) a. $1.18/gal b. $3.03/gal c. $1.47/gal d. $9.68/gal e. $2.56/gal
Practice Exercise 2 A car travels 28 mi per gallon of gasoline. What is the mileage in kilometers per liter? (1 km = 0.62137 mi, 1 gal = 3.7854 L)
Sample Exercise 1.12 Earth s oceans contain approximately 1.36 x 109 km3 of water. Calculate the volume in liters.
Practice Exercise 1 A barrel of oil as measured on the oil market is equal to 1.333 U.S. barrels. A U.S. barrel is equal to 31.5 gal. If oil is on the market at $94.0 per barrel, what is the price in dollars per gallon?
Practice Exercise 2 The surface area of Earth is 510 x 106 km2, and 71% of this is ocean. Using the data from the sample exercise, calculate the average depth of the world s ocean in feet.
Sample Exercise 1.13 What is the mass in grams of 1.00 gal of water? The density of water is 1.00 g/mL.
Practice Exercise 1 Composite decking is a manufactured substitute for wood compounded from post-consumer plastic and wood. It is frequently used in outdoor decks. The density of a particular composite decking is reported as 60.0 lb/ft3. What is the density in kg/L? (1 kg = 2.2 lbs, 1 mL = 1 cm3) a. 138 kg/L b. 0.961 kg/L c. 259 kg/L d. 15.8 kg/L e. 11.5 kg/L
