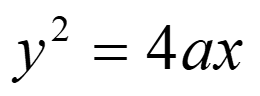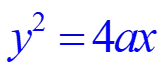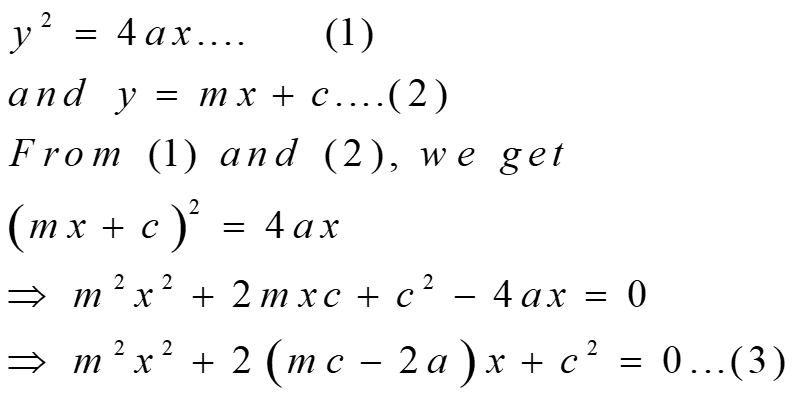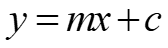Condition for Tangent and Normal to Parabola

In this lecture, we explore the conditions for a line to touch a parabola, find the equation of the tangent at any point, and determine the equation of the normal at any point on the parabola. Understanding these conditions and equations is crucial for solving problems related to parabolas in coordinate geometry. Theoretical concepts are explained with examples to aid comprehension.

• Parabola
• Coordinate Geometry
• Tangent
• Normal
• Mathematics

schnitzer_a Follow

Uploaded on Feb 23, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. 2 D Co-ordinate Geometry Lecture-11 Parabola Dated:-05.05.2020 PPT-02 UG (B.Sc., Part-1) Dr. Md. Ataur Rahman Guest Faculty Department of Mathematics M.L. Arya, College, Kasba PURNEA UNIVERSITY, PURNIA

2. Condition for tangent to the Parabola Theorem(1):-Find the condition that the Line may touch the parabola Sol:-The equations of the parabola and the st.line are given by 4 .... (1) ....(2) (1) (2), From and we get + = + + = + + = Y=mx+c = + = 2 y mx c 4 y ax P (x,y) = 2 y and y ax = + mx c ( ) 2 4 mx c ax 2 2 2 = 2 2 4 0 2 m x m x mxc mc c ax 4 y ax ( ) 2 2 2 2 0...(3) a x c

3. Continue If the given line (2) touches the parabola (1), the the two values of x given by (3) must equal. i.e the condition for which 4 0 b ac = 2 ( ( a ) 2 = 2 2 2 2 4 0 mc a m c ) + = 2 2 2 2 2 4 4 4 4 m c acm a m c = 2 4 4 ac m a m = tan c which is the required condition for gen c y

4. Continue Remarks:- (i) Putting in the eq. we get is the eq. of tangent to the Parabola (ii) Point of Contact: From (1) and (2), We get ( ( mx m a x m a y m = + a y mx c = c m a = + ....(4) y mx m 4 = 2 0. y ax for all values of m ) ( ) 2 2 a a + = + = 4 4 0 mx ax mx ax m m ) 2 a a = = 0 0 mx m a = = 2 (2) 4 From y a 2 2 m 2 = ) ( Hence Point of Contact is 2 a am , 2 m

5. Condition for tangent to the Parabola Theorem (2):-Find the equation of the tangent at any point of the parabola Solution:- The eq. of the parabola is Let P and Q be two neighbouring points on the parabola (1). ( ) = 2 , x y 4 y ax 1 1 = 2 4 .....(1) ax y ( , ) Q x y 2 2 = 2 4 ....(2) 4 ax and a x a y + y ax = 1 1 ( , ) P x y 2 ....(3) (3), and y From y y x 1 1 2 (2) y y x 2 we get x = 2 2 4 ( 4 ) 2 1 2 1 = .....(4) 2 1 y 2 1 2 1

6. Continue The eq. of the chord PQ passing through the points is 1 1 2 2 ( , ) ( , ) P x y and Q x y y x y x ( ) = y y 2 1 x x 1 1 2 1 4 a + ( ) = (4) y y x x from 1 1 y y 2 1 ( ) + = 4 0.....(5) y y y ax y y 2 1 1 2 When and PQ becomes the tangent at P Hence the eq. of the tangent at is ( ) 1 1 2 yy a x = Q P x x and y y 2 1 2 1 ( , P x y ) 1 1 = + 4 0 y y y ax + y y 1 1 ( ) x 1 1

7. Equation of normal to the Parabola Theorem (3): Find the equation of the normal at any point to the parabola Sol:-The eq. of the tangent at is Slope of the tangent So slope of the normal Hence the eq. of the normal at is = ( , P x y ) 2 4 y ax 1 1 Tangent at P ( , ) P x y ( ) = + 2 .....(2) yy a x x 1 1 1 1 ( , P x y ) 2a y 1 1 = 1 = y 1 a Normal at P 2 = 2 4 y ax y ( ) = ( , ) P x y y y 1 a x x 1 1 1 1 2