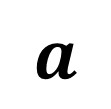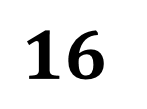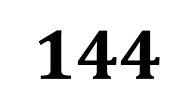Solving for the Radius of the Smallest Circle in Three Circles and a Tangent
Three circles with a common tangent are depicted, and the radii of the larger circles are provided in the diagram. By using geometric properties and calculations, the value of the radius of the smallest circle can be determined accurately.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
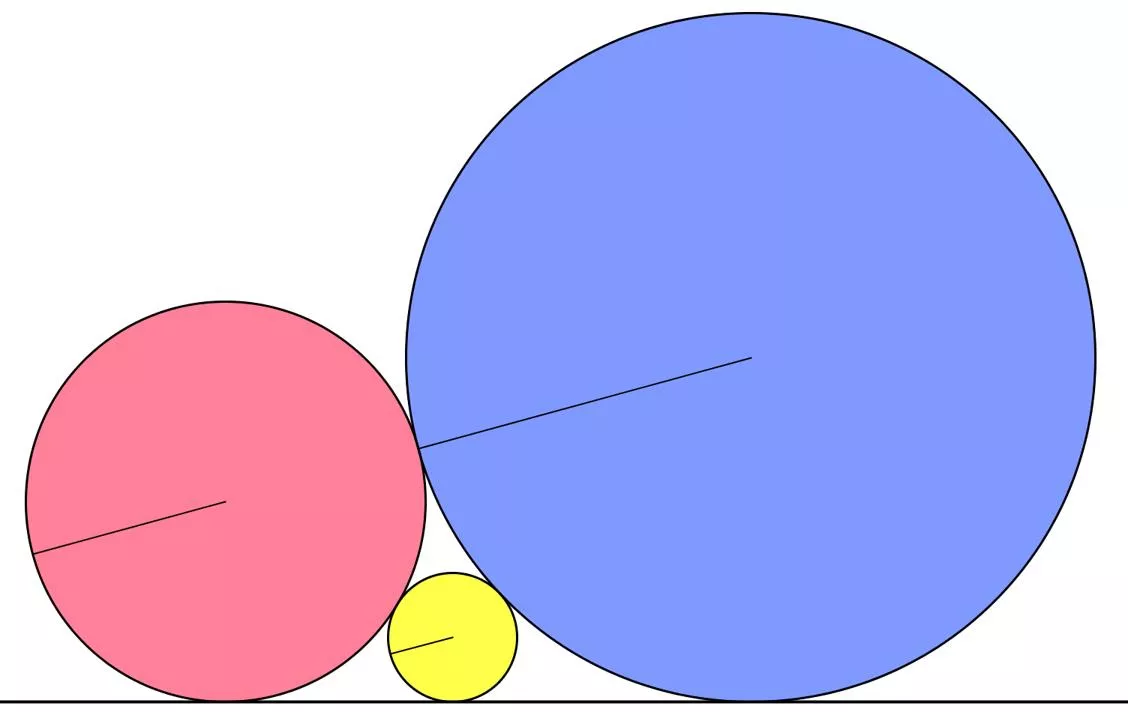
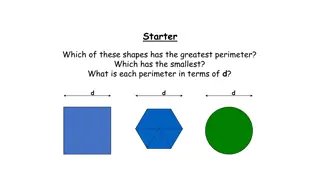
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??? ?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
Three Circles and a Tangent ? ? + ? ? ? ? ?
Three Circles and a Tangent ? ? ? + ? ? ?
Three Circles and a Tangent ? + ? ? ? ? ? ?
Three Circles and a Tangent ? + ? ? ? ? ? ? + ? ? + ? ? ?
Three Circles and a Tangent ? + ? ? ? ? + ?? ? ??= ? ?? ? ?? = ? ?? + ? ?? ? ? ? + ? ? + ? ? ? ? ?? ? ??
Three Circles and a Tangent ? ?? = ? ?? + ? ??
Three Circles and a Tangent ? ?? = ? ?? + ? ?? ?? = ?? + ?? ??? both sides 1 1 1 ?= ?+ ? Returning to our problem: 1 1 144+ 1 16 ?= 1 1 12+1 4=1 ?= 3 ? = 9
Three Circles and a Tangent 1 1 1 ?= ?+ ? ? ? ?
??? ?? ?
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??? ?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ???? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ???.?? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ???.???? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ???.?? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ???.?? ??.???? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??.?? ?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??.?? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ?? ??.???? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ?? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ?? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??? ??.???? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ??? ??.?? (Not to scale) ? What is the value of the radius of the smallest circle, ??
SIC_48 Three Circles and a Tangent The three circles are touching and share a common tangent. The radii of the two larger circles are as stated in the diagram - all units are cm. ???? ??.???? (Not to scale) ? What is the value of the radius of the smallest circle, ??