Comprehensive Overview of Two-Phase Flow Equations and Gas Pipeline Hydraulic Analysis
Explore the general flow equations for two-phase flow in pipes and gas pipeline hydraulics. Understand concepts such as internal energy, kinetic energy, potential energy, entropy, and friction factors. Learn about pressure gradients, Reynolds numbers, and friction factors in single-phase gas flow. Gain insights into the calculations and analysis involved in fluid dynamics and pipeline systems.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Ref.1: Brill & Beggs, Two Phase Flow in Pipes, 6thEdition, 1991. Chapter 1. Ref.2: Menon, Gas Pipeline Hydraulic, Taylor & Francis, 2005, Chapter 2.
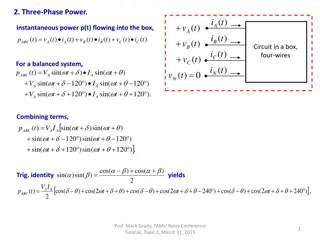
General Flow Equation U U : Internal Energy 2 1 P V Expansion : Compressio or Energy n P V 2 2 1 1 2 2 m 2 v 2 1 m v Kinetic : Energy g 2 m g c c m g Z g Z 2 Potential : Energy 1 g g c c Energy balance at steady state: 2 1 2 2 mv 2 mgZ mv 2 mgZ + + + + + = + + + 1 2 U P V 1 q W U P V 2 1 1 2 2 s g g g g c c c c = Work = Where Heat added to the fluid and done on the fluid q W s
General Flow Equation Dividing by m and writing in differential form: d g d g P v v g Z + + + + + = d d d d 0 U q W s c c By using the enthalpy and entropy definition: d P P = + = + d d d , d d h U h T S d d g d g P v v g Z + + + + + = d d d 0 T S q W s c c
General Flow Equation = ( d + For irreversible process therefore: d S T d ) q losses No Work d d g d g P v v g Z + + + + = d( ) d 0 losses W s c c For an inclined pipe, therefore: d Z = d sin L d d P L friction d d sin ( d ) P v v g losses d = + + d d L g L g L c c For Up Flow : 0 For Down Flow : 0
General Flow Equation = w v f Fanning friction factor ( f ): 2 2 g c Wall shear stress: P P+dP 2 4 d 2 P v f 2 d 4 = = w + = ( d ) ( ) d P P P d L w d L d g d f c Darcy or Moody friction factor (fm): 2 d f 2 v P = = 4 m f f m d L g d f c
General Flow Equation Pressure gradient in pipe: 2 d d sin f 2 v P v v g = + + m d d L g L g g d c c c d d d d P P P P = + + d d d d L L L L total accelerati on elevation friction Usually negligible Zero for horizontal pipe
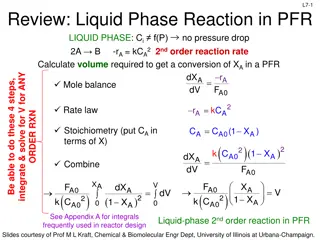
Single Phase Gas Flow Reynolds Number Reynolds Number in Gas Pipeline: 3 ( ft ) ( ft/sec ) ( lb /ft ) d v = 1488 m N Re ( cp ) q g A g = = = Mass flow rate v A q v sc sc g g g sc sc g 4 0764 . 0 d q g g d sc g 2 20 14 . ( Mscfd d ) q g g g = = 1488 N sc Re ( cp ) in ( )
Single Phase Gas Flow Friction Factor 64 N fm= Laminar Flow (NRe < 2100): Re Turbulent Flow (NRe > 2100): Moody Diagram Smooth Wall Pipe: . 0 = 5 . 0 + 32 3 6 0056 . 0 3 10 3 10 fm N for N Re Re Rough Wall Pipe: d 1 fm 21 N 25 . 9 . 0 Re . 1 = + = 14 2 log , : 0006 . 0 in Commonly 10
Single Phase Gas Flow General Equation g L g d 4 q 2 d d sin f 2 v P v v g g = + + = , m v sc 2 sc d L g g d d c c c g 2 P M sc g 4 q g PM RT sc g f sc PM PM m z RT g g 2 sin g d g z RT z RT d P g g = + d 2 L g g d c c + 2 2 8 P M q z T f sin PM g d P sc g g 2 g m g = sc 2 5 d L g z RT g T d P R c g c sc
Single Phase Gas Flow General Equation If T and zg are constant (T=Tav and zg=zav): C2 2 2 g 2 av 2 8 P q z T f sin RT M g d P sc av m g = + 2 P P sc 2 2 5 d sin L g z gT d c av av sc sin T av M g L d + P P S P g = = 1 2 2 2 P C g z R P 2 c av ( ) 1 + 2 2 P C = + 2 2 2 S S = P e P C e ln 1 S 1 2 + 2 2 P C 2
Single Phase Gas Flow General Equation 2 2 g T 16 P q z T f L M ( ) 1 0375 . 0 z ( ft ) Z sc av d av g m g = + g 2 2 S S = P e P e Where S sc 1 2 o 2 2 5 ( R ) T S R av av sc c 5 o 2 . 2 527 10 ( R) ( Mscfd) z f T in) ( q S 1 e g av m 5 av g = + 2 2 S (ft) P e P L sc 1 2 d S Le ( ) ( ) 5 . 0 5 . 0 2 2 5 2 2 5 s s T P e P d P e P d = = 198 94 . 6354 . 5 1 2 f 1 2 f sc q g z T L P z T L sc g av av m e sc g av av m e S 1 e = = : lim 1 For Horizontal Pipe L L e S 0 S
Single Phase Gas Flow Average Pressure 2 2 g T 16 P q z T f L M ( ) 1 sc av d av g m g = + 2 2 S S P e P e sc 1 2 2 2 5 S R sc c = = 2 2 2 2 1 ( L ) 0 1 Px P K x P P K L x Where x 2 1 x 2 2 2 2 P P P P 5 . 0 = = 2 2 2 1 2 ( ) x x P P x P P 1 1 2 x 1 x x 2 3 3 2 2 P P P 1 = = + = d 2 1 2 P P x P P 1 av x av + 2 2 3 3 P P P P 0 1 2 1 2
Single Phase Gas Flow Erosional Velocity Higher velocities will cause erosion of the pipe interior over a long period of time. The upper limit of the gas velocity is usually calculated approximately from the following equation: 100 = ( ft/s) v max 3 ( lbm/ft ) g Usually, an acceptable operational velocity is 50% of the above.
Single Phase Gas Flow Pipeline Efficiency In Practice, even for single-phase gas flow, some water or condensate may be present. Some solids may be also present. Therefore the gas flow rate must be multiply by an efficiency factor (E). A pipeline with E greater than 0.9 is usually considered clean .
Single Phase Gas Flow Non-Iterative Equations Several equations for gas flow have been derived from General Equation. These equations differ only in friction factor relation assumed: Gas Transmission Pipline 1. AGA equation 2. Weymouth equation 3. Panhandle A equation 4. Panhandle B equation Gas Distribution Pipeline 1. IGT equation 2. Spitzglass equation 3. Mueller equation 4. Fritzsche equation
Single Phase Gas Flow AGA Equation 2 = F The transmission factor is defined as: m f First, F is calculated for the fully turbulent zone. Next, F is calculated based on the smooth pipe law. Finally, the smaller of the two values of the transmission factor is used. d = 4 log 7 . 3 F Fully Turbulent 10 Min N N = = Re Re 4 log , 4 log 6 . 0 F F Smooth Pipe 10 10 t 4125 . 1 F F t t
Single Phase Gas Flow Weymouth Equation The Weymouth equation is used for high pressure, high flow rate, and large diameter gas gathering systems. The Weymouth friction factor is: . 0 032 fm= 3 / 1 d
Single Phase Gas Flow Panhandle A Equation The Panhandle A Equation was developed for use in large diameter natural gas pipelines, incorporating an efficiency factor for Reynolds numbers in the range of 5 to 11 million. In this equation, the pipe roughness is not used. The Panhandle A friction factor is: 0768 . 0 N fm= 1461 . 0 Re
Single Phase Gas Flow Panhandle B Equation The Panhandle B Equation is most applicable to large diameter, high pressure transmission lines. In fully turbulent flow, it is found to be accurate for values of Reynolds number in the range of 4 to 40 million. The Panhandle B friction factor is: 00359 . 0 N fm= 03922 . 0 Re
Single Phase Gas Flow Gas Transmission Equations A. Comparison of the calculated Output Pressure by AGA, Colebrook, Weymouth and Panhandle equations: Figure 2.5 B. Comparison of the calculated Flow rateby AGA, Colebrook, Weymouth and Panhandle equations: Figure 2.6 We therefore conclude that the most conservative flow equation that predicts the highest pressure drop is the Weymouth equation and the least conservative flow equation is Panhandle A.
Single Phase Gas Flow IGT Equation The IGT equation proposed by the Institute of Gas Technology is also known as the IGT distribution equation: . 0 555 2 2 s T P e P = = . 2 667 35 861 . , cp 1 2 sc q d gsc 8 . 0 g 2 . 0 P T L sc av e
Single Phase Gas Flow Spitzglass Equation The Spitzglass equation originally was used in fuel gas piping calculations. This equation has two version A. Low pressure (less than 1 psig): 5 . 0 T P P = 5 . 2 278 956 . 1 2 sc q d gsc 6 . 3 d P + + 1 ( e . 0 03 ) T L d sc g av B. High pressure (more than 1 psig): 5 . 0 2 2 S T P e P = 5 . 2 53 016 . 1 2 sc q d gsc 6 . 3 d P + + 1 ( e . 0 03 ) T z L d sc g av av
Single Phase Gas Flow Mueller and Fritzsche Equation The Mueller equation is: . 0 575 2 2 s T P e P = = . 2 725 4509 . 35 , cp 1 2 sc q d gsc 7391 . 0 g 2609 . 0 P T L sc av e The Fritzsche formula, developed in Germany in 1908, has found extensive use in compressed air and gas piping: . 0 538 2 2 s T P e P = . 2 69 41 28 . 1 2 L sc q d gsc 8587 . 0 g P T sc av e
16 in., 100 MMSCFD, 80F roughness of 700 in. for AGA and Colebrook, pipeline efficiency of 0.95 in Panhandle and Weymouth
30 in., 100 miles, 80F, output pressure of 800 psig roughness of 700 in. for AGA and Colebrook, pipeline efficiency of 0.95 in Panhandle and Weymouth