Understanding Three-Phase Power Systems and Instantaneous Power Flow

This detailed content delves into three-phase power systems, analyzing instantaneous power flow, balanced circuits, and trigonometric calculations. It explores the concept of constant three-phase power and provides insights into the analogy of a piston engine with infinite cylinders. The data includes phase voltages, currents, powers, and torque values, shedding light on single-phase and three-phase circuits' instantaneous power.

• Three-phase power
• Instantaneous power
• Balanced circuits
• Trigonometric calculations
• Electrical engineering

eithne Follow

Uploaded on Jul 17, 2024 | 2 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

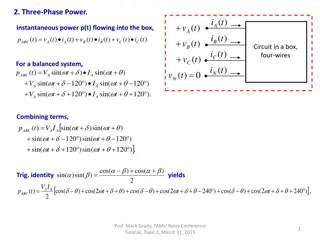
1. 2. Three-Phase Power. (t ) iA iB + (t ) vA vB vC ) t Instantaneous power p(t) flowing into the box, + = (t ) + ( ) ( ) ( ) ( ) ( ) ( ) ( ) p t v t i t v t i t v t i t + + (t ) ABC A A B B C C Circuit in a box, four-wires (t ) iC iN (t ) For a balanced system, (t ) = + + t ( V ) sin( + t ) sin( I A ) p t V t I t ( = 0 vN ABC A A + + sin( 120 ) sin( 120 ) t A + + + + + sin( 120 ) sin( 120 ). V I t A A Combining terms, = + + ( ) sin( ) sin( t ) p t V I t t ABC A A + + + sin( 120 ) sin( 120 + ) t . ) + + + + sin( 120 ) sin ( 120 t t + + cos( ) cos( ) Trig. identity yields ) sin( ) sin( = 2 cos( V I , ) = + + + + + + + + + + + + ( ) cos( ) cos( 2 ) ) cos( 2 240 ) cos( ) cos( 2 240 A A p t t t t ABC 2 Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 1

2. 2. Three-Phase Power, cont. + + + + + + + + + cos( 2 ) cos( 2 240 ) cos( 2 240 ) V I t t t = + ( ) 3 cos( ) . A A p t ABC 3 2 2 Letting x = (2 t+ + ) and expanding the time varying term, + + + = + + + cos( ) cos( 240 ) cos( 240 ) cos( ) cos( 120 ) cos( 120 ), x x x x x x + + + cos( ) cos( 120 ) cos( 120 ) x x x then expanding yields cancel each other + + + cos( ) cos( ) cos( 120 ) sin( ) sin( 120 ) cos( ) cos( 120 ) sin( ) sin( 120 ), x x x x x + + cos( ) cos( ) cos( 120 ) cos( ) cos( 120 ), x x x 1 1 + + = = cos( ) 1 cos( 120 ) cos( 120 ) cos( ) 1 , 0 x x 2 2 Instantaneous three-phase power is constant thus, smooth running machines! V I = = ( ) 3 cos( ) 3 cos( ) A A p t V I ABC Arms Arms 2 2 Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 2

3. 2. Three-Phase Power, cont. Analogous to a piston engine with an infinite number of cylinders Vmag = Vang = Imag = Iang = 1 0 Strong 120 Hz torque 0.50 -30 50 150 Phase A I(t) 1 0.433013 0.433013 0.216506 2 0.999391 0.441474 0.441205 0.216506 4 0.997564 0.449397 0.448302 0.216506 6 0.994522 0.456773 8 0.990268 0.463592 10 0.984808 0.469846 0.462708 0.216506 12 0.978148 0.475528 0.465137 0.216506 14 0.970296 0.480631 0.466354 0.216506 16 0.961262 0.485148 0.466354 0.216506 18 0.951057 0.489074 0.465137 0.216506 20 0.939693 0.492404 0.462708 0.216506 22 0.927184 0.495134 24 0.913545 0.497261 26 0.898794 0.498782 0.448302 0.216506 28 0.882948 0.499695 0.441205 0.216506 30 0.866025 0.5 0.433013 0.216506 32 0.848048 0.499695 0.423766 0.216506 34 0.829038 0.498782 0.413509 0.216506 Phase A v(t) Instantaneous Power in Single-Phase Circuit Phase A p(t) P Q Phase B v(t) Phase B I(t) -0.43301 0.216506 -0.42402 0.199067 -0.41452 0.181713 -0.40451 0.164528 -0.39401 0.147597 -0.38302 0.131001 -0.37157 0.114822 -0.35967 0.099138 -0.34733 0.084027 -0.33457 -0.32139 0.055809 -0.30783 0.042842 -0.29389 -0.2796 0.019504 -0.26496 0.009247 -0.25 -0.23474 -0.21919 Phase B p(t) Phase C v(t) Phase C I(t) 3.06E-17 -0.01745 0.009247 0.649519 -0.03488 0.019504 0.649519 -0.05226 0.03072 0.649519 -0.06959 0.042842 0.649519 -0.08682 0.055809 0.649519 -0.10396 0.06956 0.649519 -0.12096 0.084027 0.649519 -0.13782 0.099138 0.649519 -0.15451 0.114822 0.649519 -0.17101 0.131001 0.649519 -0.1873 0.147597 0.649519 -0.20337 0.164528 0.649519 -0.21919 0.181713 0.649519 -0.23474 0.199067 0.649519 -0.25 0.216506 0.649519 -0.26496 0.233945 0.649519 -0.2796 0.2513 0.649519 Phase C p(t) -1.5E-17 0.649519 A+B+C p(t) Q 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 0.375 wt 0.216506 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.125 0.034899 0.125 0.069756 0 -0.5 -0.5 Instantaneous Power in Three-Phase Circuit -0.46947 -0.43837 -0.40674 -0.37461 -0.34202 -0.30902 -0.27564 -0.24192 -0.20791 -0.17365 -0.13917 -0.10453 -0.06976 -0.0349 6.13E-17 720 -0.52992 -0.55919 -0.58779 -0.61566 -0.64279 -0.66913 -0.69466 -0.71934 -0.74314 -0.76604 -0.78801 -0.80902 -0.82904 -0.84805 -0.86603 -0.88295 -0.89879 1.5 1.5 0.45427 0.216506 0.45908 0.216506 va ia va vb ia ib 0 0 vc pa 0.06956 ic Q 0.45908 0.216506 0.45427 0.216506 pa+pb+pc 0.03072 Q -1.5 -1.5 -1.5E-17 -0.00819 -0.01529 0 90 180 270 360 450 540 630 0 90 180 270 360 450 540 630 720 Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 3

4. 2. Three-Phase Power, cont. Instantaneous Power to a Small Fan (medium power level) 200 150 100 Watts 50 0 -50 0.0000 0.0167 0.0333 0.0500 Seconds Instantaneous Power to a Compact Fluorescent Lamp 200 150 100 Watts 50 0 -50 0.0000 0.0167 0.0333 0.0500 Seconds Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 4

5. Balanced Three-Phase System 2. Three-Phase Power, cont. Imaginary Vcn Vab = Van Vbn Vca = Vcn Van 30 Real Van 120 Phasors rotate counter-clockwise. Magnitude of line-to-line voltage is ? times that of line- to-neutral voltage. Vbn Vbc = Vbn Vcn Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 5

6. Balanced Three-Phase System 2. Three-Phase Power, cont. Imaginary Vcn Vab = Van Vbn Vca = Vcn Van Ic Ica 30 Iab Real Van Ia Line currents Ia, Ib, and Ic Delta currents Iab, Ibc, and Ica Ibc Ib Ic c Ic Vbn Ica Because line-to-line voltage magnitudes are ? larger than line-to-neutral voltages, conservation of power requires that magnitudes of delta currents Iab, Ica, and Ibc are ? ?smaller than Ia, Ib, Ic. Ibc Iab Ib b a Vbc = Vab + Vbn Vcn Ia Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 6

7. c 2. Three-Phase Power, cont. c Ic Ic Z 3Z 3Z n Z Z a b a b Vab + Ib Ib 3Z Vab + Ia Ia Balanced three-phase systems, no matter if they are delta connected, wye connected, or a mix of wye and delta, are easier to solve if you follow these steps: Convert the entire circuit to an equivalent wye with a grounded neutral. Draw the one-line diagram for phase a, recognizing that phase a has one third of the P and Q. Solve the one-line diagram for line-to-neutral voltages and line currents. If needed, compute line-to-neutral voltages and line currents for phases b and c using the 120 relationships. If needed, compute line-to-line voltages and delta currents using the and 30 relationships. Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 2, March 31, 2015 7

8. 4.17A on the 240V side corresponds to 0.14A on the 7.2kV side Single-Phase Residence. 1kVA to the customer requires 4.17A in each of the two legs 30:1 single-phase transformer 7.2kV 8

9. Most commercial buildings, like ENS, are served by a 12.47kV (line-to- line) to 480V (line-to-line) three-phase transformer, delta-connected on the 12.47kV side, and grounded-wye connected on the 480V side 1kVA to the customer corresponds to 1.20A in each of the three phases Most lighting in commercial buildings is 277V fluorescent that s the voltage behind the switchplate cover. 120V outlets are served by 480:120V step down transformers on each floor of the building, often located in utility closets in two diagonally- opposite corners of each floor. 9