Modeling and Generation of Realistic Network Activity Using Non-Negative Matrix Factorization
The GHOST project focuses on the challenges of modeling, analyzing, and generating patterns of network activity. By utilizing Non-Negative Matrix Factorization (NMF), realistic network activity patterns can be created and injected into live wireless networks. Understanding and predicting user behavi
6 views • 28 slides
The Importance of Completing a Trainer Matrix
Completing a Trainer Matrix is essential for Registered Training Organizations (RTOs) to demonstrate compliance with Standards for RTOs 2015, specifically Clauses 1.13 to 1.16. This matrix outlines requirements for trainers, including holding relevant qualifications, industry skills, and maintaining
2 views • 38 slides
Math Quiz and Prime Factorization Concepts
Test your math knowledge with a quiz ranging from square numbers to factorization and explore prime factorization concepts through examples and practice questions involving finding prime factors, highest common factor (HCF), and lowest common multiple (LCM).
1 views • 26 slides
Cubes and Cube Roots: Interesting Patterns and Prime Factors
Explore the fascinating world of cubes and cube roots in mathematics with insights on patterns, prime factorization, and more. Discover the relationships between consecutive odd numbers, learn how to express numbers as the sum of odd numbers, and delve into the prime factorization of cubes. Unravel
0 views • 21 slides
Singular Value Decomposition
The Singular Value Decomposition (SVD) is a powerful factorization method for matrices, extending the concept of eigenvectors and eigenvalues to non-symmetric matrices. This decomposition allows any matrix to be expressed as the product of three matrices: two orthogonal matrices and a diagonal matri
0 views • 35 slides
Singular Value Decomposition and the Conjugate Gradient Method
Singular Value Decomposition (SVD) is a powerful method that decomposes a matrix into orthogonal matrices and diagonal matrices. It helps in understanding the range, rank, nullity, and goal of matrix transformations. The method involves decomposing a matrix into basis vectors that span its range, id
1 views • 21 slides
Comprehensive Guide to Factorization in Mathematics
Explore the concept of factorization in algebraic expressions, including the definition of factors, common factors, methods of factorization, and illustrated examples. Learn about finding factors, common factors, and various factorization techniques such as common factors method and regrouping of te
1 views • 13 slides
Mastering Quadratic Equation Factorization Techniques
Learn various factorization techniques for quadratic equations including grouping 'Two and Two', factorization of a difference of two squares, factorization of quadratic trinomials, cross-multiplication method, and use of common factors. Improve your factorization skills and solve quadratic equation
0 views • 24 slides
Diagonalization in Linear Algebra
Discover the concept of diagonalization in linear algebra through eigenvectors, eigenvalues, and diagonal matrices. Learn the conditions for a matrix to be diagonalizable, the importance of eigenvectors in forming an invertible matrix, and the step-by-step process to diagonalize a matrix by finding
1 views • 26 slides
SCET: Effective Theory of QCD
SCET, a soft collinear effective theory, describes interactions between low energy, soft partonic fields, and collinear fields in QCD. It helps prove factorization theorems and identifies relevant scales. The SCET Lagrangian is formed by gauge invariant building blocks, enabling gauge transformation
1 views • 38 slides
Matrix Factorization for Latent Factor Recovery
Explore the concept of matrix factorization for recovering latent factors in a matrix, specifically focusing on user ratings of movies. This technique involves decomposing a matrix into multiple matrices to extract hidden patterns and relationships. The process is crucial for tasks like image denois
0 views • 50 slides
Linear Equations and Matrix Operations
Explore the concepts of linear equations, matrix forms, determinants, and finding solutions for variables like x1, x2, x3. Learn about Cramer's Rules, Adjoint Matrix, and calculating the inverse of a matrix through examples and formulas.
1 views • 24 slides
Factorization Methods and Factor Tables in Mathematics
Explore the techniques of factorization through Tic-Tac-Toe method and factor tables. Learn how to find pairs of numbers that multiply to a given result, factor polynomials correctly, and understand the importance of factoring in mathematics concepts. Engage with visual representations and step-by-s
0 views • 12 slides
Communication Costs in Distributed Sparse Tensor Factorization on Multi-GPU Systems
This research paper presented an evaluation of communication costs for distributed sparse tensor factorization on multi-GPU systems. It discussed the background of tensors, tensor factorization methods like CP-ALS, and communication requirements in RefacTo. The motivation highlighted the dominance o
1 views • 34 slides
Revolutionizing Hotel Communication with Matrix Hospitality Solution
Simplify hotel operations and enhance guest experiences with Matrix Hospitality Solution. From enhancing staff efficiency to boosting revenue generation opportunities, Matrix offers a comprehensive suite of features to meet the diverse needs of hotels. Its modular configuration, scalable platform, a
1 views • 36 slides
Composite Matrix Materials in Engineering
Composite materials are made of reinforcing fibers and matrix materials, with the matrix serving to protect and enhance the properties of the composite. There are three main types of composite matrix materials: metal matrix composites (MMC), ceramic matrix composites (CMC), and polymer matrix compos
0 views • 22 slides
Non-Negative Tensor Factorization with RESCAL
This article discusses non-negative tensor factorization with RESCAL, covering topics such as Non-Negative Matrix Factorization, Multiplicative Updates, RESCAL for Relational Learning, and Non-Negative Constraint for RESCAL. It explores how factorizing matrices/tensors into non-negative factors can
2 views • 11 slides
Structural Identification in Vector Autoregressions
Explore the algebra of identification problems in VARs, including Cholesky factorization, timing restrictions, long-run impact restrictions, sign restrictions, and identification through heteroskedasticity. Discover why structural identification is crucial for policy design, economic modeling, and u
1 views • 63 slides
Essential Math Concepts: Prime Factorization, Powers, Exponents, and Multiplication Strategies
Learn fundamental math concepts such as prime factorization, powers, exponents, multiplication patterns, and problem-solving strategies with examples and visuals. Explore topics like the distributive property, estimating products, and multiplying by one-digit numbers in an engaging manner.
0 views • 9 slides
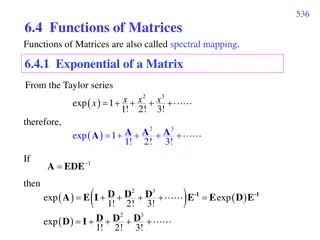
Matrix Functions and Taylor Series in Mathematics
A detailed exploration of functions of matrices, including exponential of a matrix, eigenvector sets, eigenvalues, Jordan-Canonical form, and applications of Taylor series to compute matrix functions like cosine. The content provides a deep dive into spectral mapping, eigenvalues, eigenvectors, and
0 views • 53 slides
Parallel Computation for Matrix Multiplication
Matrix multiplication is a fundamental operation with diverse applications across scientific research. Parallel computation for matrix multiplication involves distributing the computational workload over multiple processors, improving efficiency. Different algorithms have been developed for multiply
1 views • 36 slides
Elastic Net Regularized Matrix Factorization for Recommender Systems
This research paper presents an elastic net regularized matrix factorization technique for recommender systems, focusing on reducing the dimensionality of the problem and utilizing features to describe item characteristics and user preferences. The approach combines existing algorithms and applies e
0 views • 27 slides
Prime Factorization for Prime and Composite Numbers
Learn about prime and composite numbers, prime factorization, and how to identify them using examples. Discover the concept of factors, prime numbers, composite numbers, and prime factors through this educational presentation. Explore how every composite number can be expressed as a product of prime
2 views • 8 slides
Solving Quadratic Equations and Factorization
Various methods for solving quadratic equations, including factorization and the quadratic formula. Understand the concept of factorized forms and their significance in solving equations efficiently.
0 views • 37 slides
Nonsymmetric Gaussian elimination
Intricacies of nonsymmetric Gaussian elimination, LU factorization, partial pivoting, left-looking column LU factorization, symbolic sparse Gaussian elimination, column preordering for sparsity, and more in numerical linear algebra algorithms.
0 views • 28 slides
Context-aware Recommender Systems: Overview and Concepts
In this content, the Overview of COT (Contextual Operating Tensor) is presented, focusing on context-aware recommender systems. The images and related works delve into the tensor factorization and latent vectors of entities, illustrating computational procedures under different context combinations.
0 views • 22 slides
Closed Factorization
This document delves into the concept of closed factorization and closed strings, exploring the definitions, contributions, and algorithms associated with them. It introduces the Longest Closed Factor Array and its computation, along with explanations of closed strings and their unique properties. T
0 views • 33 slides
Matrices Multiplication: Understanding Matrix Dimensions and Operations
In matrix multiplication, understanding the dimensions of matrices is crucial for determining the feasibility of the operation. This involves multiplying corresponding elements of rows and columns to obtain the final matrix with specific dimensions. The order of multiplication matters, as changing i
1 views • 39 slides
Large Matrix-Matrix Multiply on PS3 Clusters - September 2010 Study
Matrix-matrix multiplication of large matrices over PS3 clusters, achieving high computational efficiency and GFLOPS performance. Challenges, approach, and results of the study are discussed in detail.
0 views • 5 slides
Python Implementation of Recommendation Algorithms for Rating Prediction and Item Recommendation
This Python library, CaseRecommender, provides implementations of various recommendation algorithms supporting rating prediction and item recommendation scenarios. It includes algorithms like ItemKNN, Matrix Factorization with BPR, UserKNN for item recommendation and Matrix Factorization, SVD, Item
0 views • 8 slides
Understanding Probabilistic Matrix Factorization for Recommender Systems
Learn about Probabilistic Matrix Factorization (PMF), a powerful technique in recommender systems, that uses user and item feature vectors to predict ratings. Discover how PMF maximizes log-posterior over user and item features and minimizes objective functions for accurate recommendations.
0 views • 8 slides
Communication Costs for Distributed Sparse Tensor Factorization on Multi-GPU Systems
Evaluate communication costs for distributed sparse tensor factorization on multi-GPU systems in the context of Supercomputing 2017. The research delves into background, motivation, experiments, results, discussions, conclusions, and future work, emphasizing factors like tensors, CP-ALS, MTTKRP, and
0 views • 34 slides
Probing Beta Decay Matrix Elements through Heavy Ion Charge Exchange Reactions
Explore research on probing beta decay matrix elements through heavy ion charge exchange reactions, investigating Dirac vs. Majorana neutrino masses and DCEX cross section factorization. Learn about nuclear transition matrix elements using reaction kernels and distortion factors in nuclear physics.
0 views • 25 slides
Methods for High-dimensional Tensor Factorization
Learn about distributed methods for high-dimensional and large-scale tensor factorization, a technique used to decompose tensors into core tensors and factor matrices. Discover the significance of tensor data in various applications such as context-aware recommendation and social network analysis.
0 views • 28 slides
Math Lessons: Prime Factorization, Powers, Multiplication Patterns, and Problem-Solving Strategies
Explore math lessons covering prime factorization, powers and exponents, multiplication patterns, problem-solving strategies, the distributive property, estimating products, and multiplying by one-digit numbers.
0 views • 9 slides
Matrix Factorization Techniques and Applications
Explore the concept of matrix factorization in data analysis, understanding the common factors between otakus and characters, and optimizing recommendations through gradient descent. Learn about latent factors, minimizing errors, and topic analysis using matrix factorization models like LSA and PLSA
0 views • 8 slides
Incremental Smoothing and Mapping in iSAM Matrix Factorization
Learn about iSAM, a system for fast incremental matrix updates, avoiding unnecessary calculations. Explore related works, SLAM problems, Gaussian measurement models, and matrix factorization techniques with Givens rotations. Discover how iSAM performs online data association efficiently. Created By:
0 views • 17 slides
Sparse Ax=b Solvers and Cholesky Factorization
Discover the landscape of sparse Ax=b solvers, from direct solvers like LU to iterative methods such as GMRES and QMR. Explore Cholesky factorization techniques, including both general and sparse column approaches. Learn about sparse Gaussian elimination, Chordal completion, and the Cholesky graph g
0 views • 21 slides
Mastering Matrix Math: Arrays, Addition, Multiplication & More
Learn how to define and name arrays, perform standard matrix operations like multiplying matrices by scalar values, matrix addition, multiplication, transposing matrices, inverting a matrix, finding determinants, and solving linear equations. Understand the process of naming cell ranges, conducting
0 views • 23 slides
Matrix Algebra and Multivariate Analysis Fundamentals
Learn about matrix algebra, identity matrix, diagonal matrix, triangular matrix, null matrix, and operations like addition and multiplication of matrices. Dive into the basics of multivariate analysis with Dr. Asmaa Ghalib Jabir.
0 views • 7 slides