Introduction to Polar Coordinates: A Different Coordinate System
Exploring the concept of polar coordinates, a coordinate system introduced in the mid-seventeenth century by notable mathematicians independently. Learn about measuring angles in radians, converting between degrees and radians, and drawing graphs in polar coordinates by considering length and angle values.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Polar Coordinates Stephen Earles ste9@aber.ac.uk s.n.f.earles@swansea.ac.uk
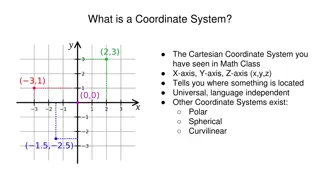
So far when plotting points on a graph we have used cartesian coordinates (named after ??? ? ????????? (1596 1650) the French philosopher and mathematician) in the ? ??? ? direction.
? ? ? ?
From the FM 4 course (which is an A2 course) you will look at a different coordinate system known as ????? ???????????
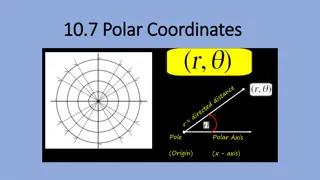
These concepts were introduced in the mid-seventeenth century by ?? ?????? ?? ????? ??????? ??? ??????????? ????????? independently. With this system of coordinates, you work with a distance from a reference point (usually the origin) called the ????. You also need to measure the angle from a reference direction (usually the positive x-axis) called the ??????? ????.
These angles can be measured in terms of ??????? or in terms of ??????? . We can convert between degrees and radians as follows, 360 = 2? ???? 180 = ? ???? 90 = 30 = ?2 ?6
Convert the following angles from degrees into radians. ?) 45 ?) 60 ?) 120 ?) 135 ?) 150 ?) 210 ?) 225 ) 240 ?) 270 ?) 300 ?) 315 ?) 330
Radians are normally used to measure angles in the A level course. The graph paper is very different for Polar coordinates.
To draw a graph in Polar coordinates, we need 2 values (for some graphs we will need 3 values), the length of the line ? and the angle of turn ? . The value of ? will be important in the diagrams that we draw.
These graphs are also called Roses and as such they have petals. We need to look at the relationship between ? ??? ? and the picture that we obtain.
Draw the following graphs ? ? = 4cos 3? ?? ? = 4cos 5? ??? ? = 4cos 7? ?? ? = 4cos ?? ? ? = 4cos(2?) ?? ? = 4cos(4?) ??? ? = 4cos(6?) ???? ? = 4cos ??
Draw the following graphs ? ? = 4sin 3? ?? ? = 4sin 5? ??? ? = 4sin 7? ?? ? = 4sin ?? ? ? = 4sin(2?) ?? ? = 4sin(4?) ??? ? = 4sin(6?) ???? ? = 4sin ??
With polar coordinates we can draw different types of graphs. ???? ??? (?????????) ???????????
??????? (?????????) We need to consider the following graphs ? = ? ?cos? ? = ? ?sin? We need to carefully look at the values of a and b .
Draw the following graphs ? ? = 1 + 2???? ?? ? = 1 2???? ??? ? = 4 + 4???? ?? ? = 5 + 3???? ? ? = 5 3????
For the graphs on the previous slide, change "cos" ??? "???"
??????????? With these curves, the value of the angle is always"2 " Draw the graph of ?2= ?2cos2?
?????? These are of the form ? 10 ? = ?
???????? Consider the equation 4? ? = 1 + ?cos?
With this equation we have 4 cases that we need to look at, ? ? = 0 ?? 0 < ? < 1 ??? ? = 1 ?? ? > 1
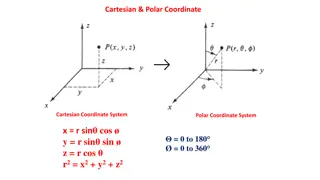
Converting between polar and Cartesian coordinates
To convert between polar and Cartesian coordinates we use the information from slide 3, which is ? = ?cos? ? = ?sin?
Find the cartesian equation of each of these curves ? = 4 ?cos? = 3 ?sin? = 7
Find the polar equation of each of these curves ?2+ ?2= 9 ?2 9+?2 16= 1 ?2+ ?2= 6?
Area enclosed by a polar curve. To do this we need to use the concept of differentiation that we first encounter in unit 1 of the A level Mathematics course.