Understanding Curvilinear Motion with Cylindrical Coordinates in Physics
Cylindrical coordinates, specifically the r- coordinate system, are useful in describing curvilinear motion. This system helps explain motion in relation to a fixed origin, making it ideal for scenarios involving rotation or changes in angle. By using radial and transverse unit vectors, positions, velocities, accelerations, and even magnitudes can be analyzed effectively. Polar and Cylindrical coordinate systems provide unique perspectives depending on the observer’s frame of reference, offering insights into complex motion scenarios.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Cylindrical Coordinate System (r-) Yay! Another coordinate system! You ve played with this one in calculus classes already. Now we are going to use this system to describe curvilinear motion. At first glance the r- coordinate system (polar) may seem similar to the n-t system. This is somewhat true, in that the unit vectors *do* change orientation. However, in this coordinate system the origin is fixed. Rather than describe the particle location using x and y we are going to describe it using r and . In 2D we call r- coordinate system Polar Coordinates . In 3D we call r- -z coordinate system Cylindrical Coordinates . Pop Quiz?: Are Polar and Cylindrical coordinate systems Eulerian viewpoints Lagrangian viewpoints Observations from a fixed coordinate system Observations from a moving coordinate system
Cylindrical Coordinate System (r-) So, when should you choose to use polar coordinates? If you want to describe motion from a fixed position, x-y and r- are both fine choices. If the motion you are describing is rotating around a fixed point, then polar is a great choice. If the motion is described in relation to a rotation (angle change) then polar is a necessary choice. A vehicle going around a curve makes for an interesting argument You know almost all of 12.7 practice problems are Lagrangian vehicle problems. But if you were standing at the radius of curvature you would probably choose polar coordinates to describe the motion, velocity, and acceleration. How about a vehicle racing down a drag strip? Lagrangian n-t analysis is pretty simple (v and a in just the tangential direction) Driver Perspective Eulerian x-y analysis is pretty simple (v and a in just one direction) Pit Crew observer on track behind vehicle Eularian r- analysis is less simple (v and a change in both r and ) Spectator in the stands
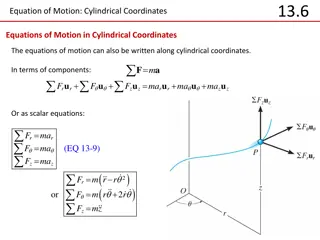
12.8 Curvilinear Motion: Cylindrical Components u (radial unit vector) (transverse unit vector) u r Polar Coordinates: = r u r Position: r Velocity: = = v r + u u r r r r = = v r + = u u u u r r r r = = v r + u u (EQ 12-24) v v r r = = r v v r r (EQ 12-25) velocity magnitude: = + r ( ) r ( ) v 2 2
12.8 Curvilinear Motion: Cylindrical Components u (radial unit vector) (transverse unit vector) u ( r r r dt r r r = = + + a v u u r Polar Coordinates: d ) = = a v + u u Acceleration: + + u u u r r r r = = a v r r + + = = u u u u u u ( ) ( 2 ) & r r 2 r r r = + a u u (EQ 12-28) a a r r = = + r a a r r r 2 (EQ 1 2-29) 2 r Acceleration magnitude: = r r + + ( 2 2 ) ( 2 ) a r r 2
12.8 Curvilinear Motion: Cylindrical Components u u u (radial unit vector) (transverse unit vector) (rectangular unit vector) r Cylindrical Coordinates : z = + r u u r z Position: r z = + + v u u u (EQ 12 -31) r r z Velocity: r z Acceleration: = r r + + + a u u u ( ) ( 2 ) (EQ 12-32) r r z 2 r z
In-Class Practice Problem 1 What do you notice about this problem? Key words? What information do you know? What are you trying to find? Why use polar coordinates for this problem? What equation(s) will you use? Is the car accelerating in the tangential direction? If so, how to you know?
In-Class Practice Problem 1 (checking answer) Let s check part of our answer to the previous problem using n-t coordinates Assume constant velocity of 20 m/s (what direction?) Calculate normal acceleration (what direction)? = v (EQ 12-19) a v ?? ? = 0,??= 0 t 2 = (EQ 12- 20) a ?2 ??]2 200 [?]= n 400 [ ?2] ??=20[ ? ?2 = 2 200 [?] In polar coordinates we got -2 m/s2. Why is this version positive?
In-Class Practice Problem 2 No picture? You want *me* to draw the picture? What do you notice about this problem? Key words? What information do you know? What are you trying to find? Why use polar coordinates for this problem? What equation(s) will you use?
In-Class Practice Problem 2 Solution doesn t even include the plot Typical Graduate students responsible for most solution manuals Ain t nobody got time fo dat!
In-Class Practice Problem 2 Can do this via substitution ? = 2? ? = 2cos 2? 2 When ? = 0, r = _____ When ? =? 4, r = _____ When ? =? 2, r = _____ 0 -2
In-Class Practice Problem 3 Have you seen mechanisms like this? Key words? What information do you know? What are you trying to find? Why use polar coordinates for this problem? What equation(s) will you use?
In-Class Practice Problem 4 What do you notice about this problem? Key words? What information do you know? What are you trying to find? Why use polar coordinates for this problem? What equation(s) will you use?
In-Class Practice Problem 4 Let s check part of our answer to the previous problem using n-t coordinates Constant velocity of 20 m/s (what direction?) Calculate normal acceleration (what direction)? = v (EQ 12-19) a v ? = 0,??= 0 t 2 = (EQ 12- 20) a ?2 ??]2 60 [?] n 400 [ ?2] ??=20[ ? ?2 = = 6.67 60 [?] In polar coordinates we got ar = -6.67 m/s2. Why is this version positive?