Understanding Quantum Mechanics Postulates and Operators
Explore the foundational principles of quantum mechanics, including postulates describing quantum systems, wavefunctions, probabilistic nature, Hermitian operators, eigenvalues, and their significance in measuring physical observables.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
EE 315/ECE 451 NANOELECTRONICS I
OUTLINE 2 3.1 General Postulates of QM 3.2 Time-Independent Schr dinger Equation 3.3 Analogies Between Quantum Mechanics and Electromagnetics 3.4 Probabilistic Current Density 3.5 Multiple Particle Systems 3.6 Spin and Angular Momentum 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
WHERETO BEGIN? 3 ( x , t ) Ae i ( kx t ) = Start with a plane wave i ( x , t ) 1 ( x , t ) 2 , k 2 = = ( x , t ) t ( x , t ) x 2 1 p 2 2 E mv 2 V + V + 2 = = m Classically ( ) 2 i ( x , t ) k 2 E V + , = = = ( x , t ) t , m Quantize energy with DeBroglie 1 ( x 2 t ) 2 2 V + = 2 m ( x , t ) x ( x , t ) ( x , t ) 2 2 Voila! Schr dinger's Equation i V + ( x , t ) = t 2 m x 2 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
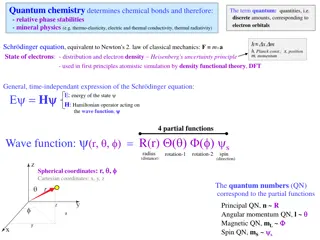
3.1 GENERAL POSTULATES OF QUANTUM MECHANICS 4 POSTULATE 1 - To every quantum system there is a state function, (r,t), that contains everything that can be known about the system The state function, or wavefunction, is probabilistic in nature. Probability density of finding the particle at a particular point in space, r, at time t is: t 2 ) = = 2 ( r , t ) r , ( , ) = P ( r t d r ( r , t ) ( r , t ) d r 3 * 3 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
POSTULATE 1 5 2 ( r = , t ) r , ( t 2 ) , ) = P ( r t d r ( r , t ) ( r , t ) d r 3 * 3 = Normalization Factor allspace ( r , t ) ( r , t ) d 3 r 1 * = 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
POSTULATE 2 6 A) Every physical observable O O (position, momentum, energy, etc.) is associated with a linear Hermitian operator B) Associated with the operator is the eigenvalue problem, n= n n such that the result of a measurement of an observable is one of the eigenvalues n of the operator c) If a system is in the initial state , measurement of O O will yield one of the eigenvalues n of with probability ) 2 P ( ) ( r , t ( r ) d 3 r = n n And the system will change from (an unknown state) to n. 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
3.1.1 OPERATORS 7 An operator maps one quantity to another For example 3x2 matrices map 2x1 onto 3x1 matrices The derivative operator maps sine onto cosine The operator in that case would be d/dx 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
3.1.2 EIGENVALUESAND EIGENFUNCTIONS 8 An eigenfunction of an operator is a function such that when the operator acts on it we obtain a multiple of the eigenfunction back n= n n n are the eigenvalues of the operator 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
3.1.3 HERMITIAN OPERATORS 9 A special class of operators. They have real eigenvalues Their eigenfunctions form an orthogonal, complete set of functions 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
3.1.4 OPERATORSFOR QM 10 x p i i = Momentum operator - E i = Energy - t x = x Position ] [ , ( ) = Commutator ] [ , 0 = 2 operators commute if which allows measurement to arbitrary precision 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
3.1.5 MEASUREMENT PROBABILITY 11 If a system is already in an eigenstate of the operator we are interested in, we are guaranteed 100% to measure it in that state However, if we do not know the state, we can only find the probability of finding it in that state Once measured however, we are locked in to that state, and subsequent measurements will return the same value 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
COLLAPSEOFTHE STATEFUNCTION -THE MEASUREMENT PROBLEM 12 Postulate 2 says that any observable measurement is associated with a Linear Hermitian operator, and the result of every measurement will be an eigenvalue of the operator After measurement (observation) the system will be in an eigenstate until perturbed 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
POSTULATE 3 13 The mean value of an observable is the expectation value of the corresponding operator f f ( x ) ( x ) dx = ) ( x dx 1 = 2 ( r , t ) ( r , t ) ( r , t ) ( r , t ) * = = For a QM system O ( r , t ) o ( r , t ) d r * 3 = x ( r , t ) x ( ) i ( r , t ) ) d r * 3 = Position p ( r , t i ( r , t ) d r * 3 = t Momentum E ( r , t ) ( r , t ) d r * 3 = Energy 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
POSTULATE 4 14 The state function (r,t) obeys the Schr dinger equation ) t ( r , t i H ( r , t ) = Where H is the Hamiltonian (total energy operator), kinetic + potential energy (plus field terms if necessary) 2 H V ( r , t ) 2 = + 2 m 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
3.2 TIME-INDEPENDENT SCHR DINGER'S EQUATION 15 If the potential energy does not depend on time we can simplify considerably to the time- independent Schr dinger Equation 2 V + ( r ) ( r ) E ( r ) 2 = 2 m 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315
QUANTUM CORRAL 16 An example of standing electron waves 8/11/2015 J. N. DENENBERG- FAIRFIELD UNIV. - EE315