Understanding Wave Diffraction and its Implications
Explore the phenomenon of wave diffraction, which involves the spreading and bending of waves as they encounter openings or obstacles. Learn how diffraction influences the behavior of light waves and how it is essential for creating interference patterns. Discover the relationship between gap size, wavelength, and diffraction effects in various scenarios.
Uploaded on | 1 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
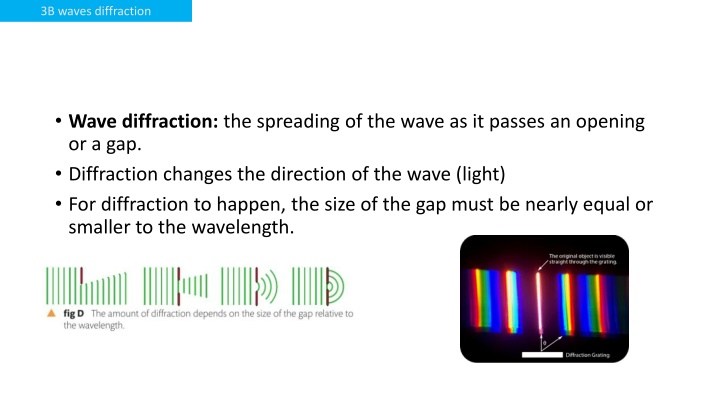
3B waves diffraction Wave diffraction: the spreading of the wave as it passes an opening or a gap. Diffraction changes the direction of the wave (light) For diffraction to happen, the size of the gap must be nearly equal or smaller to the wavelength.
3B waves diffraction Wave diffraction: the spreading of the wave as it passes an opening or a gap. Diffraction changes the direction of the wave (light) For best diffraction to happen, the size of the gap must be nearly equal or smaller to the wavelength. Much larger gaps than wavelengths do not produce diffraction as the wave can move through it easily
3B waves diffraction Wave diffraction: the spreading of the wave as it passes an opening or a gap. Diffraction occurs when a wave encounters an obstacle or a non-uniformity in its medium. For light waves, this could include passing through a small opening, around corners, or over an edge. When multiple waves overlap, they may interfere constructively or destructively, resulting in patterns of bright and dark regions. A path difference of half a wavelength leads to destructive interference, while a full wavelength results in constructive interference
3B waves diffraction Spacing between the slits (in meter) Law of diffraction Law of diffraction Order of diffraction (unitless 1st, 2nd, #) n = d sin sin of the angle of diffraction wavelength (in meter) 2nd 1st d 0th 1st 2nd
3B waves diffraction Spacing between the slits (in meter) Law of diffraction Law of diffraction Order of diffraction (unitless 1st, 2nd, #) n = d sin sin of the angle of diffraction wavelength (in meter) 2nd 1st d 0th 1st 2nd
3B waves diffraction Spacing between the slits (in meter) Law of diffraction Law of diffraction Order of diffraction (unitless 1st, 2nd, #) n = d sin sin of the angle of diffraction wavelength (in meter) 2nd 1st d 0th 1st 2nd
3B waves diffraction Color of light is a property of its wavelength Different wavelengths of light diffract at different angles Shorter wavelengths diffract less and have shorter gaps between them
3B waves diffraction Example Example A grating with 1000 lines per millimeter is illuminated by monochromatic light of wavelength 500 nanometers. What is the angle of the first-order diffraction? n = d sin = 10 1000 00 x x 10 3 ???? 10 3 1 x 500 x 10 1 x 500 x 10- -9 9 = Sin Sin = ??? ? ?? ? 1 1 x x ?? ? = 0.5 sin sin = = 0.5 arcsin arcsin 0.5 = 30 0.5 = 30
3B waves diffraction Example Example A grating with 1000 lines per millimeter is illuminated by monochromatic light of wavelength 500 nanometers. What is the angle of the first-order diffraction? n = d sin 00 x x ?? ? ???? 9 = = 10 1000 1 x 500 x 10 1 x 500 x 10- -9 Sin Sin = ??? ? ?? ? 1 1 x x ?? ? = 0.5 sin sin = = 0.5 arcsin arcsin 0.5 = 30 0.5 = 30
3B waves diffraction Example Example A grating with 1000 lines per millimeter is illuminated by monochromatic light of wavelength 500 nanometers. What is the angle of the second-order diffraction? n n = = d d sin sin
3B waves diffraction Example Example A grating with 1000 lines per millimeter is illuminated by monochromatic light of wavelength 500 nanometers. What is the angle of the second-order diffraction? n n = = d d sin sin 1000 00 x x ?? ? ???? = 10 2 x 500 x 10 2 x 500 x 10- -9 9 = Sin Sin = ???? ? ?? ? 1 1 x x ?? ? = 1 sin sin = = 1 arcsin arcsin 1 = 90 1 = 90
3B waves diffraction Example Example A grating with 1000 lines per millimeter is illuminated by monochromatic light of wavelength 500 nanometers. What is the angle of the second-order diffraction? n n = = d d sin sin 1000 00 x x ?? ? ???? = 10 2 x 500 x 10 2 x 500 x 10- -9 9 = Sin Sin = ???? ? ?? ? 1 1 x x ?? ? = 1 sin sin = = 1 arcsin arcsin 1 = 90 1 = 90
3B waves diffraction Example Example A grating with 900 lines per millimeter what is the spacing (in meters) between the slits ?
3B waves diffraction Example Example
3B waves diffraction Example Example