Understanding Vertical and Horizontal Asymptotes in Rational Functions
Vertical and horizontal asymptotes play a crucial role in understanding the behavior of rational functions. Vertical asymptotes occur when the denominator of a rational function equals zero, leading to excluded values. On the other hand, horizontal asymptotes are determined by comparing the degrees of the numerator and denominator polynomial functions. This guide explains how to find and interpret both types of asymptotes in rational functions with illustrative examples.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
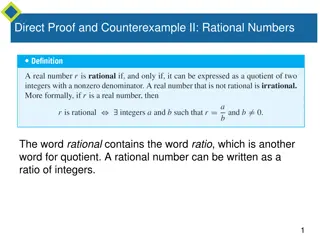
Vertical and Horizontal Asymptotes of Rational Functions
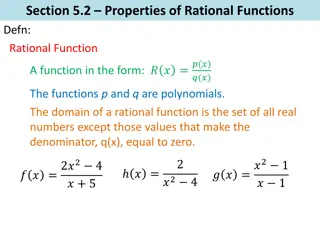
Rational Functions A rational function f(x) is a function that can be written as ) ( q where p(x) and q(x) are polynomial functions and q(x) 0 . ( ) p x = f x ( ) x A rational function can have more than one vertical asymptote, but it can have at most one horizontal asymptote.
Vertical Asymptotes If p(x) and q(x) have no common factors, then f(x) has vertical asymptote(s) when q(x) = 0. Thus the graph has vertical asymptotes at the excluded values.
Vertical Asymptotes V.A. is x = a, where a represents real zeros of q(x). Example: 2 2 x = Find the vertical asymptote of ( ) . f x 1 x 2 = = ) 1 + Since the zeros are 1 and -1. Thus the vertical asymptotes are x = 1 and x = -1. ( ) 1 ( 1 )( q x x x x
Horizontal Asymptotes A rational function f(x) is a function that can be written as ) ( q where p(x) and q(x) are polynomial functions and q(x) 0 . ( ) p x = f x ( ) x The horizontal asymptote is determined by looking at the degrees of p(x) and q(x).
Horizontal Asymptotes p x f = ( ) x ( ) ( ) q x a. If the degree of p(x) is less than the degree of q(x), then the horizontal asymptote is y = 0. b. If the degree of p(x) is equal to the degree of q(x), then the horizontal asymptote is coefficien leading y = t of ( ) p x . leading coefficien t of ( ) q x c. If the degree of p(x) is greater than the degree of q(x), then there is no horizontal asymptote.
Horizontal Asymptotes deg of p(x) = deg of q(x), then H.A. is leading y = deg of p(x) < deg of q(x), then H.A. is y = 0 ( ) p x = ( ) f x ( ) q x coefficien t of ( ) p x . leading coefficien t of ( ) q x deg of p(x) > deg of q(x), then no H.A. Example: Find the horizontal asymptote: Degree of numerator = 1 Degree of denominator = 2 3 2 x = ( ) . f x 1 x Since the degree of the numerator is less than the degree of the denominator, horizontal asymptote isy = 0.
Horizontal Asymptotes deg of p(x) = deg of q(x), then H.A. is leading y = deg of p(x) > deg of q(x), then no H.A. deg of p(x) < deg of q(x), then H.A. is y = 0 ( ) p x = ( ) f x ( ) q x coefficien t of ( ) p x leading coefficien t of ( ) q x Example: Find the horizontal asymptote: Degree of numerator = 1 Degree of denominator = 1 Since the degree of the numerator is equal to the degree of the denominator, horizontal asymptote is . + 3 1 x = ( ) . f x 2 1 x 3 y = 2
Horizontal Asymptotes deg of p(x) = deg of q(x), then H.A. is coefficien leading y = deg of p(x) > deg of q(x), then no H.A. deg of p(x) < deg of q(x), then H.A. is y = 0 ( ) p x = ( ) f x ( ) q x t of ( ) p x leading coefficien t of ( ) q x Example: Find the horizontal asymptote: Degree of numerator = 2 Degree of denominator = 1 + x 3 1 2 = f x ( ) . x 2 1 Since the degree of the numerator is greater than the degree of the denominator, there is nohorizontal asymptote.
Vertical & Horizontal Asymptotes H.A. : V.A. : x = a, where a represents real zeros of q(x). deg of p(x) < deg of q(x), then H.A. is y = 0 deg of p(x) = deg of q(x), then H.A. is coefficien leading y = deg of p(x) > deg of q(x), then no H.A. t of ( ) p x leading coefficien t of ( ) q x Practice: Find the vertical and horizontal asymptotes: 2 + 3 1 x = ( ) f x 2 1 x Answer Now
Vertical & Horizontal Asymptotes H.A. : V.A. : x = a, where a represents real zeros of q(x). deg of p(x) < deg of q(x), then H.A. is y = 0 deg of p(x) = deg of q(x), then H.A. is coefficien leading y = t of ( ) p x leading coefficien t of ( ) q x deg of p(x) > deg of q(x), then no H.A. Practice: Find the vertical and horizontal asymptotes: 1 3 ) ( x V.A. : x = + x 2 = f x . 1 2 1 2 H.A.: none
Vertical & Horizontal Asymptotes H.A. : V.A. : x = a, where a represents real zeros of q(x). deg of p(x) < deg of q(x), then H.A. is y = 0 deg of p(x) = deg of q(x), then H.A. is coefficien leading y = t of ( ) p x leading coefficien t of ( ) q x deg of p(x) > deg of q(x), then no H.A. Practice: Find the vertical and horizontal asymptotes: 2 ) ( 2+ x x = f x 1 Answer Now
Vertical & Horizontal Asymptotes H.A. : V.A. : x = a, where a represents real zeros of q(x). deg of p(x) < deg of q(x), then H.A. is y = 0 deg of p(x) = deg of q(x), then H.A. is coefficien leading y = t of ( ) p x leading coefficien t of ( ) q x deg of p(x) > deg of q(x), then no H.A. Practice: Find the vertical and horizontal asymptotes: 2 2+ x = ( ) f x V.A. : none H.A.: y = 0 1 x 2+ 1 x is not factorable and thus has no real roots.