Understanding Thermodynamic Energy Balances in Solids with Jacobian Algebra
Explore the intricacies of energy balances in solids through the lens of Jacobian algebra, covering topics such as mechanics of stress and strain, Gibbs-like functions for physical properties, and third-order derivatives for thermodynamic identities. Unravel the application of Jacobians for transformations and the interrelations among properties in thermodynamics. Delve into thermoelasticity and the manipulation of variables in solid systems. Discover the significance of thermodynamics in various disciplines and the key role it plays in predicting self-consistent phenomena.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Thermodynamic Energy Balances in Solids Special Lecture on using Jacobian Algebra by S. J. Burns High Energy Density Science Materials Science Program Mechanical Engineering Department Introduction Energy balances in fluids Jacobians for transformations to other variables Solids: mechanics of stress and strain Energy balances in solids Physical properties and elastic properties found using Gibbs like functions Third order derivatives for thermodynamic identities Example of a sheared solid with only three variables Finding constant volume lines in solids 9/11/2024 Thermoelasticity with Jacobian Algebra 1
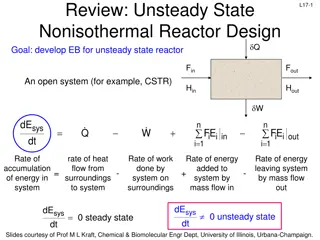
Introduction Why study thermodynamics? Energy balances are universal but very different for each discipline: Physics, Chemistry, Biology, Engineering, Materials, etc. All have their own Disciplines in Thermodynamics. Most don t understand what the others do!! Thermodynamics is always useful for finding self-consistent phenomena. Especially useful in complex chemical systems. Thermodynamics by itself predicts nothing. Assumptions about a system are often included in models of the phenomona! Energy balances in the First Law has been around for so many years it is indisputable. Entropy is very difficult to understand. Key problem is to define the system: Closed or Open 9/11/2024 Thermoelasticity with Jacobian Algebra 2
= internal energy = absolute temperature entropy = pressure = specific volume u T s Energy balances in fluids: = du g dg dT = Td u T s pdv s + s = p v pv vdp + - Construct a table that contains all the physical properties Use Jacobian Algebra Heat Capacity Thermal Expansion Isothermal compressibility 3rd order derivatives of Gibbs function (unique) Easy to find all interrelations among properties 9/11/2024 Thermoelasticity with Jacobian Algebra 3
3rd Order Jacobian Table for a Fluid 9/11/2024 Thermoelasticity with Jacobian Algebra 4
You use this table to change variables in the system Here is how it works: dy y x y x y x y x y x y x = + 1 1 dx dx 1 1 2 = = = ( , ) y y x x 1 2 1 1 1 x x x x 2 = + 2 2 dy dx dx y y y ( , y ( , ) ) 2 1 2 2 2 1 2 1 2 = + 3 3 1 2 3 3 dy dx dx 3 1 2 1 2 I want to let and vary while holding constant. So y y y y dy dx dx x x y y dy dx dx x x y dx dx x y 1 2 3 = + + 1 1 0 1 1 2 1 2 = + + 2 2 0 2 1 2 1 y x 2 = + 3 3 0 0 + 1 2 1 2 9/11/2024 Thermoelasticity with Jacobian Algebra 5
The determinate of the matrix must be zero for a solution. y x y x y x y x y x y x y x y x y x y x y x y x 1 1 dy 1 1 dy 1 1 1 2 = 0 0 0 1 dx dx 1 2 2 2 dy = 2 2 0 det dy 2 1 2 1 2 1 1 2 1 2 3 3 0 3 3 0 1 2 1 2 Expand matrix Cramer s rule y x y x y x y x y x y x y x y x 1 1 ( , ( J y y ) ) J y y y y 1 2 det = 1 3 1 3 3 y y 1 2 = 1 , 2 2 2 y 2 2 3 3 y 3 1 2 det 3 3 1 2 9/11/2024 Thermoelasticity with Jacobian Algebra 6
0 1 c T p v c ( , ) ( , ) J T p T J p s s p = = = 1 0 c T vT s p v The Jacobian method works for stress and strain in thermo-elasticity These systems have 7 independent variables. 9/11/2024 Thermoelasticity with Jacobian Algebra 7
Phase Diagram Pressure versus Temperature for Quartz 9/11/2024 Thermoelasticity with Jacobian Algebra 8
Mechanics of Stresses 9/11/2024 Thermoelasticity with Jacobian Algebra 9
Hookes Law Applied to a Plane Wave No strain in x and y, so stresses are equal when material is isotropic = x y 1 = The z stress is from Poisson s effect = Poisson's ratio z x + (1 2 )(1 (1 ) The z strain is from Hooke s law but we need adiabatic moduli and volume changes since stresses are huge. = z z ) E + (1 2 )(1 (1 ) = = z z ) v E Shear strains are through-the- thickness as shown not in-plane. Need strain volumes in energy balances. Volume change is seen above. 9/11/2024 Thermoelasticity with Jacobian Algebra 10
Fluids: First law of thermodynamics for a fluid like material The system is per unit mass has 2 independent variables du Td pdv = s- Solids: First law for a solid material is more complex. The system is per unit mass and has 7 independent variables du Td v d ij ij = + s = d vd Strain volume is used in all my work: For a solid 1st law: ij ij du Td = + d s ij ij d du Td = + s In vector representation: i i i = 1, 2 and 3 dilatational while 4, 5 and 6 deviatoric 9/11/2024 Thermoelasticity with Jacobian Algebra 11
Gibbs like free energy function with independent stresses: g u T = s i j So = dg dT d s i i The independent state variables (7 variables) are T and i while the equation is per unit mass. 1st derivatives of g gives the state variables 2nd derivatives of g gives the elastic and thermal properties 3rd derivatives of g gives interactions among properties These third order quantities are new but mostly of academic interest There are 196 3rd order terms and 77 new expressions as follows: 9/11/2024 Thermoelasticity with Jacobian Algebra 12
Jacobian table for a thermo-elastic solid with 6 independent stresses 9/11/2024 Thermoelasticity with Jacobian Algebra 13
Solid with Applied Pressure and Applied Shear Stress = du Td -pdv+v d d vd du Td -pdv+ d g u T +pv- dg=- dT+vdp- d s s = s s Use only a single shear (there are 3 independent) All entries in the Jacobian table to the right are definitions. c, and are heat capacity, volume thermal expansion and shear thermal expansion respectively. is compressibility. S is shear compliance. 9/11/2024 Thermoelasticity with Jacobian Algebra 14
= S dS dT = = T , p ( , , ) ( , , ) J s J p p s = Want to find: or v , , p p s s dS dT s = = v v 0 / - 0 1 0 0 0 1 / - 0 1 0 v c T v vS v , p T dS dT 2 2 v T = d v d s = = vS c v dS dT c T v v 2 = s 2 ' 2 2( ) '' S = [1 ] S , S S p s 9/11/2024 Thermoelasticity with Jacobian Algebra 15
Hookes Law for isotropic materials for normal dilatational strains with thermal strains Hooke s Law in isotropic materials; shear strains have no thermal strains at zero stress. 9/11/2024 Thermoelasticity with Jacobian Algebra 16
Engineering shear stress and strain Adiabatic lines must not cross as shown to the left If the adiabatic lines cross 2nd law of thermo is violated in the Kelvin - Planck statement 9/11/2024 Thermoelasticity with Jacobian Algebra 17
No corrections from isothermal to adiabatic for shear waves reason: thermal expansion coefficient is zero but only at zero stress! In shear, thermal expansion coefficient is only zero at zero stress/strain. That is only at one point on the shear stress vs shear strain system. Points in thermo systems: triple point, critical point, spinodal point, etc. 9/11/2024 Thermoelasticity with Jacobian Algebra 18
Here is a curve for entropy in shear in copper All the entropy lines are repulsed away from the point of zero stress - zero strain. All the isotherms must go through that point. 9/11/2024 Thermoelasticity with Jacobian Algebra 19
Derivation of entropy lines from shear with isothermal linear stress vs strain = + s du g dg Td u = d s = T dT d s 1 = S(T ) (T ) T s = = = s T = T or dS dT dS dT dS dT S T = = T = d d s 2 = s 2 9/11/2024 Thermoelasticity with Jacobian Algebra 20
Modulus vs Temperature Matched low and high temperature Data sets at T = 300K At the Repulsion Point we have: All values of T and all values of P 9/11/2024 Thermoelasticity with Jacobian Algebra 21
Solve the differential equation at the Repulsion Point for the slope of an adiabatic line 2 T = S c s ( ) S S 2 'S = 1 2 S '' s + 1 m = S 1 m s = m constant v T 9/11/2024 Thermoelasticity with Jacobian Algebra 22
Experimentally Measure Temperature and Pressure In a plot of pressure versus temperature for a given material I d like to find the line that keeps the volume constant: = ( , ) v p T = v v T v p + dv dT dp Thermal Expansion Compressibility dp dT p v T = = constant = v T v p 0 + dT dp Measure v p T v T v p v T v p = + v T p know and know p T p T 9/11/2024 Thermoelasticity with Jacobian Algebra 23
9/11/2024 Thermoelasticity with Jacobian Algebra 24
Stability: Entropy vs Temperature where does it change sign? When the Curvature Changes Sign: Use Modulus when the Strain is Constant Use the Compliance when Stress is Constant 9/11/2024 Thermoelasticity with Jacobian Algebra 25
Conclusions Shear modulus depends only on the specific volume The volume depends on the temperature and pressure Shear changes the shape of a solid but not the volume The value of m describes entropy slopes in shear and also atomic compression Isothermal lines are isochoric lines in shear systems Bulk modulus obeys Repulsion Point thermoelasticity Solids really are very, very different from gases! Two of three phonons are in shear. Constant stress free energies are about 85% from shear energies This universal shear law should have been found quite some time ago!! Most solids are liquids in literature. 9/11/2024 Thermoelasticity with Jacobian Algebra 26