Understanding Proportions and Confidence Intervals in Statistics
Explore the concept of proportions in statistics, where the parameter of interest is the percentage of a population with a specific characteristic. Learn how confidence intervals help us assess the reliability of our estimates and how to calculate them using sample statistics. Discover the importance of assumptions in checking the accuracy of these inferences and how to apply the formulas for proportions effectively.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Proportions: Confidence Intervals Chapter 13
Proportions Parameter of interest (p): percentage of the population with a certain characteristic. Examples: percentage of smokers in US, fraction of people who donate in a national organization Statistic (phat): Percentage of a sample with the characteristic used to estimate the parameter of interest to make inference. The statistic gives us a single number estimate .What if we want to tell someone how good our estimate is?
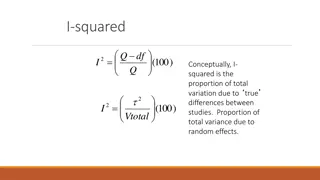
Confidence Intervals Example: We are 95% confident that between 7% and 21% of babies born in 1998 were premature. Interval includes TWO numbers, giving a range rather than one number Formula for proportions:
Why: The Sampling Distribution of Proportions Under certain assumptions, phat, the estimated proportion, follows a Normal Distribution True mean = p, the population proportion (typically unknown) Standard Deviation = sqrt(p*q/n) q = 1-p n is sample size We don t know p? Use phat instead of p in formulas Standard Deviation becomes known is standard error with this substitution
Assumptions to Check Independent Samples Randomization was used 10% Condition Success/Failure Condition
Using the Confidence Interval Formula Phat: From information given, find n = sample size # of interesting outcome Phat = #/n S.E. : after calculating phat S.E.(phat)= sqrt((phat)*(1-phat)/n) Critical Value: AKA zstar From z tables .but just memorize!! For 99% confidence -> zstar = 2.576 *****For 95% confidence -> zstar= 1.96**** For 90% confidence -> zstar = 1.645
In Class Problem Page 435, #5 Find phat. Calculate S.E. from phat. Can you find a 95% Confidence interval now? Standard error is the best estimate of the standard deviation of the sampling distribution of the proportion. This measures from sample to sample what kind Of variation we expect.
In Class Problem Page 435, #6
In Class Problem Page 438, #36 Find n. Calculate phat. Calculate standard error. Choose correct critical value from dictated confidence level. Use formula to get the lower and upper end of the interval. Check to see if 2% is within this interval.
Margin of Errorthe width of the Confidence Interval Margin of error (ME) = zstar*SE(phat). It s the part you add and subtract to phat to build your confidence interval You will be asked on your test tricky questions about manipulating parts of the ME and how that affects the other parts.
In Class Problem Page 437, #27 Hint: First, write down the formula for ME=zstar*sqrt((phat)*(1-phat)/n). For each question, write more down to walk you through the scenario.
WHAT TO STUDY FOR EXAM Chapter 9: Multiple Regression How to interpret b0, b1, and b2 in multiple regression How to interpret R^2 in multiple regression How to plug in values Chapter 10: Sample Surveys and Bias Every type of sampling method -> name and process -> know how to identify one from an explained scenario Every type of bias -> name and how it s possible -> know how to identify
WHAT TO STUDY CONT Chapter 11: Experiments and Observational Studies Identify if experiment or observational study Within Experiment: Identify experimental units Identify response Identify number of factors Identify number of treatments Identify blind and double blind Identify blocking Identify control group Identify placebo effect
WHAT TO STUDY CONT Chapter 11 cont. Within Observational Study: Identify Retrospective vs prospective Chapter 12: Probability Rules to have DOWN: Complement Rule General Addition Rule General Probability Rule Venn Diagram Drawing Tree Diagram Drawing
WHAT TO STUDY CONT Chapter 12 Cont Problems to practice: 5 houses problem: Remember you need to find the individual probability first. Then write down the overall problem they want. You will most likely have to use the complement rule on a problem like this. DRAW PICS. Tree diagram problem: You won t be forced to use Baye s rule .make sure you know how to use the diagram to calculate the reversed conditional probability instead. Drawing a Venn Diagram and then using the General Addition Rule. Identifying when wording is implying a CONDITIONAL probability and not the INTERSECTION probability.
WHAT TO STUDY CONT Chapter 13: Confidence Intervals for Proportions Practice the problems from this slideshow Make sure you are comfortable with the one that manipulates the Margin of Error.