Understanding Ratios and Proportions in Geometry
Explore the concept of ratios and proportions in geometry, focusing on how they are used to solve problems and prove relationships in geometric figures. Learn about properties of proportions, similarity criteria for triangles, and the importance of identifying and applying similar polygons. Discover how scale factors, AA Postulate, SAS, and SSS similarity theorems play a key role in geometric measurements and calculations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
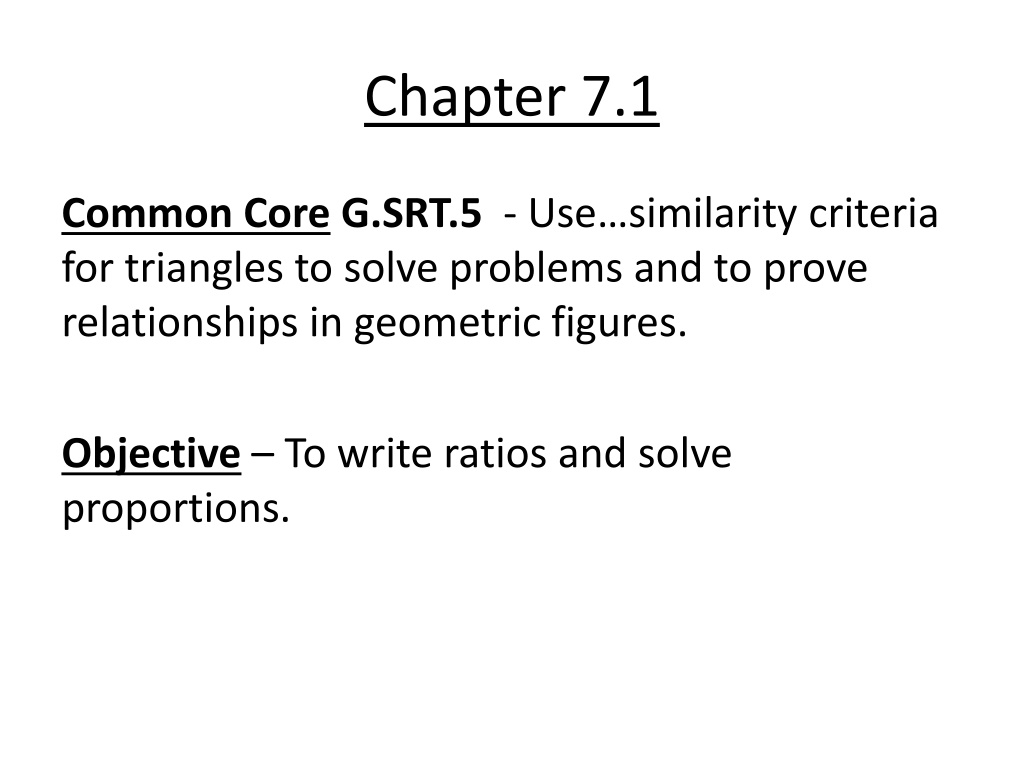
Chapter 7.1 Common Core G.SRT.5 - Use similarity criteria for triangles to solve problems and to prove relationships in geometric figures. Objective To write ratios and solve proportions.
Chapter 7.1 Notes Ratio if a and b are 2 quantities that are measured in the same units, then the ratio of a to b is a/b. (i.e. a ratio is a fraction) Proportion is an equation that equates 2 ratios Means a = c b d Extremes
Properties of Proportions If a = c , then ad = bc (Cross Product Prop.) b d If a = c , then b = d (Reciprocal Prop.) b d a c
Properties of Proportions If a = c , then a = b b d c d (Rotation) If a = c , then a+ b = c + d b d b d (Add the denominator to the numerator)
Chapter 7.2 Common Core G.SRT.5 - Use similarity criteria for triangles to solve problems and to prove relationships in geometric figures. Objective To identify and apply similar polygons.
Chapter 7.2 Notes Similar Polygons when you have 2 polygons that have all corresponding s are and all corresponding sides are in the same proportion then they are similar (~) A X Y Z B C
Thm if 2 polygons are ~, then the ratio of their perimeters is equal to the ratios of their corresponding side lengths Scale Factor if 2 polygons are ~, then the ratio of the lengths of 2 corresponding side is called the scale factor. We usually write scale factors like this a:b
Chapter 7.3 Common Core G.SRT.5 & G.GPE.5 Use similarity criteria for triangles to solve problems and to prove relationships in geometric figures. Prove the slope criteria for parallel and perpendicular lines and use them to solve geometric problems. Objectives To use the AA Postulate, SAS, & SSS similarity Theorems. To use similarity to find indirect measurements.
Chapter 7.3 Notes Similar Triangles 1) AA (angle-angle similarity postulate) If 2 s of one triangle are to 2 s of another triangle, then the 2 s are ~
Similar Triangles 2) SSS (side-side-side similarity postulate) 3) SAS (side-angle-side similarity postulate)
Chapter 7.4 Common Core G.SRT.5 & G.GPE.5 Use similarity criteria for triangles to solve problems and to prove relationships in geometric figures. Prove the slope criteria for parallel and perpendicular lines and use them to solve geometric problems. Objective To find and use relationships in similar right triangles.
Chapter 7.4 Notes Thm if the altitude is drawn to the hypotenuse of a right triangle, then the two triangles formed are similar to the original triangle and to each other. 1 ~ 2 ~ the big 1 2
Chapter 7.5 Common Core G.SRT.4 Prove theorems about triangles a line parallel to one side of a triangle divides the other two proportionally. Objective To use the Side-Splitter Theorem and the Triangle-Angle-Bisector Theorem.
Chapter 7.5 Notes Side Splitter Thm If then
Thm If then Thm If then