Understanding Fractions: Introduction and Types with Examples
Fractional numbers represent parts of a whole unit. Learn about introductory concepts of fractions, types such as equivalent, improper, proper, like, unlike, unit fractions, and mixed numbers. Explore examples and solve practice questions on equivalent fractions to enhance your understanding.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
CLASS: V SUBJECT: MATHEMATICS TOPIC: FRACTIONAL NUMBER
Contents Introduction of fractional numbers Types of fractional numbers Fraction in the lowest terms Simplification of fraction by HCF Comparing fractions Arrangement of Fractions in ascending and descending order Addition, subtraction, multiplication fractional numbers Reciprocal fraction and division of
Introduction of fractional numbers Definition: These are the numbers that represent one or more parts of a unit that has been divided in equal parts. Fraction consists of two numbers Fraction Numerator Denominator
Types of fractions 1. Equivalent Fractions 2.Improper Fractions 3. Proper Fractions 4. Like Fractions 5.Unlike Fractions 6.Unit Fractions 7. Mixed Number
1. Equivalent Fractions: Fractions which express the value of the same part of a whole are called equivalent fractions. Example:
2. Improper Fractions: Fractions whose numerators are greater than or equal to the denominators are called proper fractions. 3. Proper Fractions: Fractions whose numerators are smaller than denominators are called improper fractions.
4. Like Fractions: Fractions having same denominators are called like fractions. 5. Unlike Fractions: Fractions having different denominators are called unlike fractions.
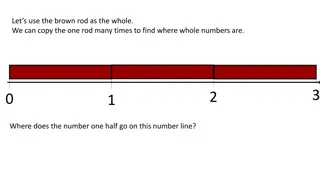
6.Unit Fractions: Fractions whose numerators are equal to one are called unit fractions. Example: 7. Mixed Number: Improper Fraction written as combination of a whole and a proper fraction is called mixed number. Example:
Solve these questions 1.Write next three equivalent fractions. (a) 3/6, 6/12, 9/18, .. , .. , .. (b) 1/9, 2/18, 3/27, .. , .. , .. (c) 15/20, 30/40, 45/60, .. , .. , .. 2. Convert the following into improper fractions. (a) 3 1/7 = (b) 5 1/9 = (c) 33 1/3 = .. (d) 2 2/11 = 3. Convert the following into a mixed number. (a) 45/6 = . (b) 21/6 = . (c) 18/4= . (d) 38/5=
Using this activity we can explain type of fractions (like equivalent, like, unlike, proper, improper etc) to the students. Activity
Fraction in the lowest term Fraction in the lowest term When we divide the numerator and denominator of a fraction by a common factor other than 1 , we get lower terms of the fraction. When we confirm that there is no common factor between numerator and denominator other than 1 then the fraction is the lowest term. Example : Reduce 18 24 in its lowest term. Solution : 18 24 = 18 2 2 = 9 12 3 3= 3 24 4 3 4is the lowest term of 18 24 because 3 and 4 are have no common factor other than
SIMPLIFICATION OF FRACTION BY HCF SIMPLIFICATION OF FRACTION BY HCF Example : Reduce20 80 to the lowest term. Solution: HCF of 20 and 80 is 20 20 80 20 20 (numerator and denominator both divided by the HCF 20) 1 4 is the lowest form. =
Class Work Class Work - - 1 1 Reduce the following into its lowest form. (a) 60 100 (b) 35 70 (c) 26 91 (d) 30 80 (e) 25 65
HOME ASSIGNMENT HOME ASSIGNMENT 1. which of the following is in the lowest form. (a) 30 210 (b) 5 3 2. Tick ( ) those fraction which are in the lowest term. (a) 6 70 , 60 15 , 2 140 , 3 6 , 4 7 , 90 12 , 1 10 _______ (b) 3 3. Reduce the following in its lowest term. (a) 9 8 _______ (c) 14 23 __________ 12 (b) 28 56 (c) 48 54 (d) 22 55
Comparing fractions Method 1
Compare of unlike fraction by taking LCM Compare of unlike fraction by taking LCM 16 and 15 3 Example : Compare of unlike fractions , 28 LCM of 16 and 28 is 112 3 16 = 3 7 15 28= 15 4 112 21 112 16 7 = 28 4 = 60 21 112 < 3 16 <15 60 112 28
#Compare the fractions by cross multiplication method. (a) 11 6 (b) 4 18 (c) 3 4 (d) 9 5 (e) 1 4 18 and 1 9and 5 5 and 3 10and 2 2 and 1
Fraction Subtraction Example
Word Problems Example 1: Rahul ate 3 4 kg of toffees. He gives 2 3 of it to his sister. How much toffee was given to his sister? Answer: Quantity of toffee Rahul was = 3 4 kg 3 of 3 Quantity of toffees given to his sister = 2 4 kg 3 4 Kg = 2 3 x = 1 2 Kg Rahul s sister gets 1 2 Kg toffees. Example 2: In a class of 20 students, 3 4 are girls. Find the number of girls present in the class? Answer: Total students = 20 Number of girls = 3 4 of 20 = 3 4 x 20 = 15 There are 15 girls in the class.
Reciprocal Fraction The reciprocal of a fraction is obtained by changing its numerator and denominator. The reciprocal of a number is also called its multiplicative inverse. The product of a number and its reciprocal is equal to 1. Examples
Division of Fractions Example
Properties of division of fractional numbers # Property 1: A fraction divided by one is the fraction itself. Example: 11 16 1 = 11 16 x 1 = 11 16 # Property 2: Zero divided by any fraction is zero. Example: 0 11 16 = 0 16 = 0 x 11 # Property 3: A fraction divided by itself is one. Example: 11 16 11 16 = 11 16 x 16 11 = 1
Concept Map Types of fractional numbers Fraction in the lowest terms Simplification of fraction by HCF Comparing fractions Reciprocal fraction Operation on fractions