Understanding Compound Interest Formulas and Examples
Compound interest is a powerful concept in finance that calculates the growth of an investment over time. This summary explains the formula for compound interest, how to calculate account balances and interest earned, and examples for various scenarios. You'll also learn how to solve for the principal and interest rate in compound interest calculations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
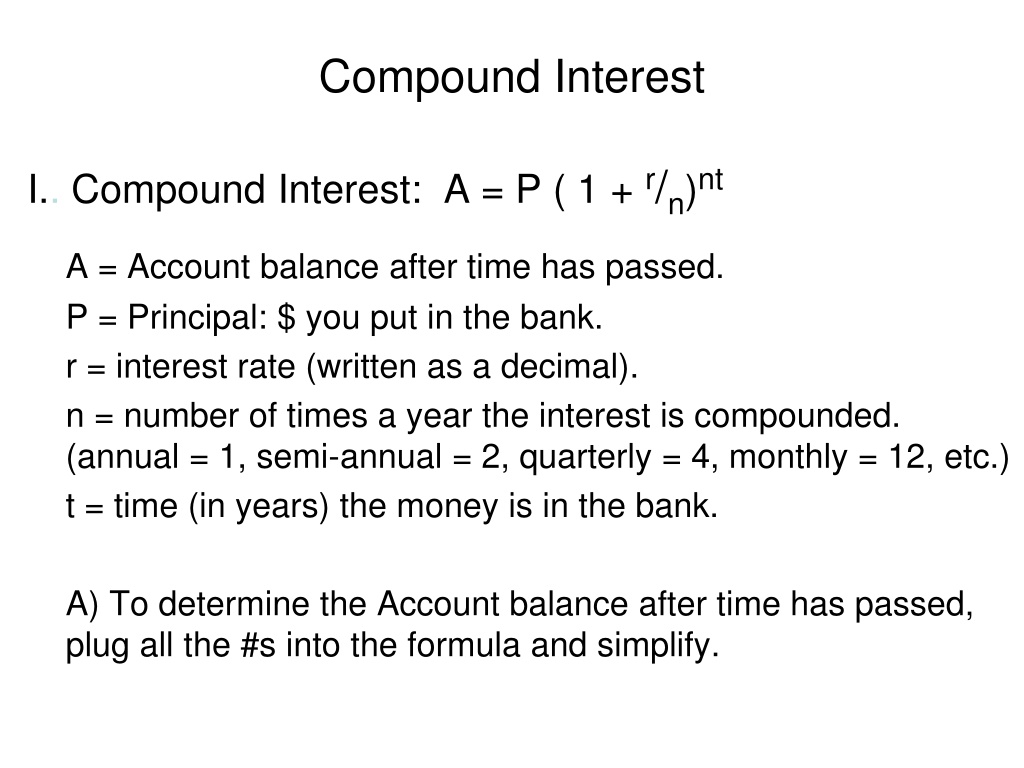
Compound Interest I.. Compound Interest: A = P ( 1 + r/n)nt A = Account balance after time has passed. P = Principal: $ you put in the bank. r = interest rate (written as a decimal). n = number of times a year the interest is compounded. (annual = 1, semi-annual = 2, quarterly = 4, monthly = 12, etc.) t = time (in years) the money is in the bank. A) To determine the Account balance after time has passed, plug all the #s into the formula and simplify.
Compound Interest Examples: 1) If you deposit $4000 in an account that pays 2.92% interest semi-annually, what is the balance after 5 years? How much did the account earn in interest? A = P ( 1 + r/n)nt A = 4000 ( 1 + .0292/2)2 5 A = 4000 ( 1 + .0146 )10 A = 4000 (1.0146)10 A = $ 4623.90 So the account gained $623.90 dollars in the 5 years.
Compound Interest Examples: 2) If you deposit $12,500 in an account that pays 4.5% interest quarterly, what is the balance after 8 years? How much did the account earn in interest? A = P ( 1 + r/n)nt A = 12500 ( 1 + .045/4)4 8 A = 12500 ( 1 + .01125 )32 A = 12500 (1.01125)32 A = $ 17,880.64 So the account gained $5380.64 dollars in the 8 years.
Compound Interest II.. Solving for P in Compound Interest: A = P (1 + r/n)nt A) Plug all the #s into the formula. B) Simplify the ( )ntpart. C) Divide both sides by the ( )ntpart. nt + 1 r A n = P nt nt + 1 + 1 r r n n
Compound Interest Examples: 3) How much would you have to deposit in a savings CD paying 4.9% annually so that you will have $60,000 in your account after 12 years? A = P ( 1 + r/n)nt 60,000 = P ( 1 + .049/1)1 12 60,000 = P ( 1 + .049 )12 60,000 = P (1.049)12 (1.049)12 (1.049)12 P = $ 33,795.20
Compound Interest III.. Solving for r in Compound Interest: A = P (1 + r/n)nt A) Plug all the #s into the formula. B) Divide by the P part to get (1 + r/n)ntby itself. C) Get rid of the exponent with a radical. 1) Use a reciprocal fractional exponent. D) Evaluate the A/P^(1/nt) term with a calculator. E) Solve for r. 1) Move the decimal 2 places to get a %.
Compound Interest Examples: 4) Your Great Grandpa bought his bride to be an engagement ring valued at $200.00 back in 1928. It was appraised in 2008 as being worth $12,500.00. What was the rate of increase per year? A = P ( 1 + r/n)nt 12500 = 200 ( 1 + r/1 )1 80 12500 = 200 ( 1 + r )80 200 200 62.5 = (1 + r )80 62.5^(1/80) = ( 1 + r )80 (1/80) 1.053 = 1 + r (subtract the 1) .053 = r so r = 5.3%