The Fascinating Euler Paths and the Seven Bridges of Konigsberg
Explore the intriguing world of Euler paths through the historical context of the Seven Bridges of Konigsberg. Learn about Leonhard Euler, the concept of Euler paths, and how to determine if a shape has a valid Euler path. Dive into the reasoning behind Euler paths and why they work with shapes of 0 or 2 odd degrees, as illustrated by the impossibility of traversing all seven bridges of Konigsberg in one go.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
7 BRIDGES OF KONIGSBERG By Ava and Rachel
BACKGROUND OF THE 7 BRIDGES OF KONIGSBURG - 7 bridges of Konigsberg was based in modern day Kaliningrad, Russia. - The 7 bridges were built over a river that ran through the city. - For centuries mathematicians have pondered Euler's discovery of the relationships between vertices, edges, and degrees, in the 7 bridges of Konigsberg. It is impossible to cross all the seven bridges using a Euler path.
HISTORY OF LEONHARD EULER - Born on April 15, 1707 in Basel, Switzerland. - Was a physicist scholar-Worked in the fields of geometry, Trigonometry, and calculus - Worked in St. Petersburg Academy, where he eventually lost his sight. - He died on September 18, 1783 in Russia.
WHAT IS A EULER PATH - A Euler path is a path that covers every edge of the shape only once. - For example, a Euler path is possible for a square with a single line through it
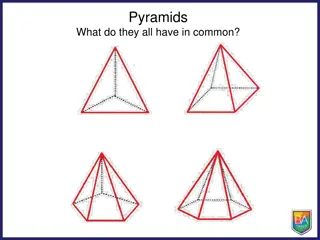
GIVE IT A TRY Now use the piece of paper given to you to find which of the following can or cannot have a Euler path. A good way to do this is to trace along the edge without removing your pencil from the paper. Start at one of the vertices or the corners.
SOLUTIONS TO THE PROBLEMS Shape A does not have a Euler path. There is no way to trace along each of the edges without retracing at least one edge. Shapes B and C both have a Euler path. It is difficult.
WHY DOES THIS WORK? A Euler path works for shapes that have 0 or 2 odd degrees ONLY This leads back to the Bridges of Konigsberg. The map looks like this Here is another way the bridges can be drawn
THE IMPOSSIBLE SHOWN Therefore, there is no possible way to cross each bridge once because there are 4 odd degrees in the diagram.