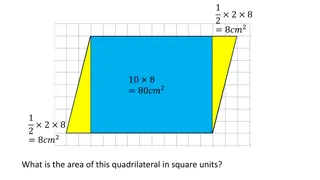
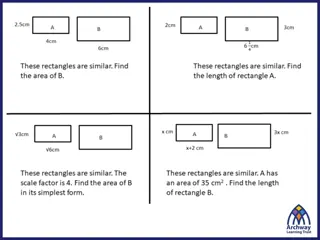
Solving a Geometric Progression Problem with Given Differences
The problem involves determining the two possible values of the first term and the associated common ratio in a geometric progression, given the differences between terms. By following the steps outlined in the solution, the first term is calculated to be 1.2 or 3.2, and the common ratio is determined as 2. The calculations are illustrated step by step to aid understanding.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
A Geometric Progression Problem Source: Aidan Burrows (don t know where he got it from)
Note to Teacher Hand out the worksheets and if they struggle take them through the specific problem that follows. You can also set the related problem as an extension task (slide 9).
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 20. The fourth term is greater than the first by 35. Find the two possible values of the first term and associated common ratio.
? 1 2 3 4 ??2 ??3 ?? ? ?? = ? + 20 = ? + 35 ??3= ? + 35 ?? = ? + 20 ? =? + 20 ?3=? + 35 ? ? ?+203 ?3 ?+35 ? = ? + 203= ?3+ 35?2 ?3+ 60?2+ 1200? + 8000 = ?3+ 35?2 25?2+ 1200? + 8000 = 0 ?2+ 48? + 320 = 0 ? + 40 ? + 8 = 0 ? = 40 , 8 ? =1 2, 3 2
? 1 2 3 4 ??2 ??3 ?? ? ?? = ? + 20 = ? + 35 ??3= ? + 35 ?? = ? + 20 ? =? + 20 ?3=? + 35 ? ? ?+203 ?3 ?+35 ? = ? + 203= ?3+ 35?2 ?3+ 60?2+ 1200? + 8000 = ?3+ 35?2 25?2+ 1200? + 8000 = 0 ?2+ 48? + 320 = 0 ? + 40 ? + 8 = 0 ? = 40 , 8 ? =1 2, 3 2
? 1 2 3 4 ??2 ??3 ?? ? ?? = ? + 20 = ? + 35 ??3= ? + 35 ?? = ? + 20 Alternatively: 20 35 (? 1) a = a = ?3 1 ? 1 20 ? 1= 35 ?3 1 20 ?3 1 = 35 ? 1 20 ?2+ ? + 1 ? 1 = 35 ? 1 20r2+ 20? + 20 = 35 4?2+ 4? 3 = 0 2r + 3 2? 1 = 0 ? =1 2, 3 2 ? = 40 , 8
? 1 2 3 4 ??2 ??3 ?? ? ?? = ? + 20 = ? + 35 ??3= ? + 35 ?? = ? + 20 Alternatively: 20 35 (? 1) a = a = ?3 1 ? 1 20 ? 1= 35 ?3 1 20 ?3 1 = 35 ? 1 20 ?2+ ? + 1 ? 1 = 35 ? 1 20r2+ 20? + 20 = 35 4?2+ 4? 3 = 0 2r + 3 2? 1 = 0 ? =1 2 , 3 2 ? = 40 , 8
? = 40 ,8 Answer: ? =1 2 , 3 , respectively 2 Check: ? 1 2 3 4 ??2 ??3 ?? ? ?? 40 20 10 5 8 12 18 27
A Related Geometric Progression Problem The second term of a geometric progression is greater than the first term by 20. By what amount should the fourth term be greater than the first to yield a unique solution? Find the first term and common ratio.
? 1 2 3 4 ??2 ??3 ?? ? ?? = ? + 20 = ? + ? ??3= ? + ? ?? = ? + 20 20 Q ? = ? = ?3 1 ? 1 20 ?3 1 = Q ? 1 20 ?2+ ? + 1 ? 1 = Q ? 1 20?2+ 20? + 20 = Q 20?2+ 20? + 20 ? = 0 ? = 20 400 4 20 20 ? 40 ? = 20 80? 1200 40 For a unique solution 80? 1200 = 0
? 1 2 3 4 ??2 ??3 ?? ? ?? = ? + 20 = ? + ? ??3= ? + ? ?? = ? + 20 20 Q ? = ? = ?3 1 ? 1 20 ?3 1 = Q ? 1 20 ?2+ ? + 1 ? 1 = Q ? 1 20?2+ 20? + 20 = Q 20?2+ 20? + 20 ? = 0 ? = 20 400 4 20 20 ? 40 ? = 20 80? 1200 40 For a unique solution 80? 1200 = 0
Q =1200 = 15 80 ? = 20 = 1 40 20 2 = 40 ? = 1 3 2 1 ? 1 2 3 4 ??2 10 ??3 ?? ? ?? 40 20 3 5 3 3 3 If we used ?in stead of 20 the general solution for ? would be: ?2 4? ? ? 2? 4?? 3?2 2? ? = ? = ? ? ?=3 4yields a single solution with ? = 2 3?and? = 1 Showing that 2 .
Q =1200 = 15 80 ? = 20 = 1 40 20 2 = 40 ? = 1 3 2 1 ? 1 2 3 4 ??2 10 ??3 ?? ? ?? 40 20 3 5 3 3 3 If we used ?in stead of 20 the general solution for ? would be: ?2 4? ? ? 2? 4?? 3?2 2? ? = ? = ? ? ?=3 4yields a single solution with ? = 2 3?and? = 1 Showing that 2 .
4?? 3?2 2? ? = ? 7? 4 3?2 ? 4? If ? ?=7 4then we get: ? = 2? ? = ? 7?2 3?2 2? ? = ? 2? 2? ? =1 2or? = 3 2 and ? = 2? or? = 2? 5, respectively. Do your answers to the original problem accord with the above? They should!
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 4. The fourth term is greater than the first by 7. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 8. The fourth term is greater than the first by 14. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 12. The fourth term is greater than the first by 21. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 16. The fourth term is greater than the first by 28. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 20. The fourth term is greater than the first by 35. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 24. The fourth term is greater than the first by 42. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 28. The fourth term is greater than the first by 49. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 32. The fourth term is greater than the first by 56. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 36. The fourth term is greater than the first by 63. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 40. The fourth term is greater than the first by 70. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 44. The fourth term is greater than the first by 77. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 48. The fourth term is greater than the first by 84. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 52. The fourth term is greater than the first by 91. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 56. The fourth term is greater than the first by 98. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 60. The fourth term is greater than the first by 105. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 64. The fourth term is greater than the first by 112. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 68. The fourth term is greater than the first by 119. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 72. The fourth term is greater than the first by 126. Find the two possible values of the first term and associated common ratio. SIC_13
A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 76. The fourth term is greater than the first by 133. Find the two possible values of the first term and associated common ratio. SIC_13 A Geometric Progression Problem The second term of a geometric progression is greater than the first term by 80. The fourth term is greater than the first by 140. Find the two possible values of the first term and associated common ratio. SIC_13