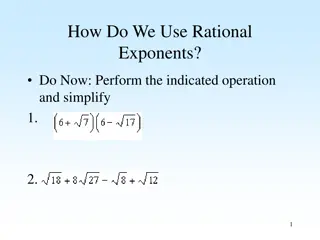
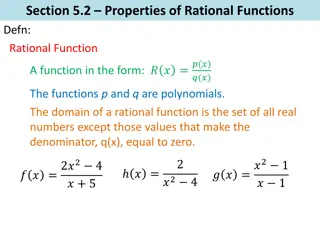
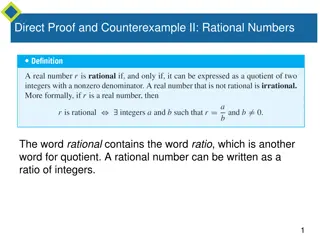
Rational Numbers: Subtracting Tips and Examples

Learn how to subtract rational numbers, including integers, by following key rules such as changing the subtraction sign to addition and adding the opposite of the second number. Visual explanations and practice problems help reinforce the concept.

• Rational numbers
• Subtracting
• Integers
• Rules
• Practice

azai_338 Follow

Uploaded on Sep 21, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. RATIONAL NUMBERS ~ SUBTRACTING RATIONAL NUMBERS

2. Rational Numbers

3. SUBTRACTING RATIONAL NUMBERS Subtracting Integers To subtract an integer, add its additive inverse.

4. SUBTRACTING RATIONAL NUMBERS Subtracting a smaller number from a larger number is the same as finding how far apart the two numbers are on a number line. Subtracting an integer is the same as adding its opposite. How do I subtract Integers? Words Numbers Change the subtraction sign to an addition sign and change the sign of the second number. Algebra 2 3 = 2 + ( 3) 4 ( 5) = 4 + 5 a b = a + ( b) a ( b) = a + b

5. SUBTRACTING RATIONAL NUMBERS Rules: 1.Change the SUBTRACTION sign to an ADDITION sign. 2.Change the sign of the SECOND addend to its OPPOSITE sign. 3.This is now an addition problem! Follow the rules for adding integers!

6. SUBTRACTING RATIONAL NUMBERS Subtract. A. 7 4 7 4 = 7 + ( 4) Add the opposite of 4. = 11 Same sign; use the sign of the integers. B. 8 ( 5) 8 ( 5) = 8 + 5 Add the opposite of 5. = 13 Same sign; use the sign of the integers. C. 6 ( 3) 6 ( 3) = 6 + 3 Add the opposite of 3. = 3 6 > 3; use the sign of 6.

7. SUBTRACTING RATIONAL NUMBERS Your Turn! Subtract. A. 3 ( 6) 3 ( 6) = 3 + 6 Add the opposite of 6. Same signs; use the sign of the integers. = 9 B. 4 1 4 1 = 4 + ( 1) Add the opposite of 1. = 5 Same sign; use the sign of the integers. C. 7 ( 8) 7 ( 8) = 7 + 8 Add the opposite of 8. = 1 8 > 7; use the sign of 8.

8. SUBTRACTING RATIONAL NUMBERS Evaluate the expression for the given value of the variable. A. 8 j for j = 6 8 j 8 ( 6) Substitute 6 for j. = 8 + 6 Add the opposite of 6. = 14 Same sign; use the sign of the integers.

9. SUBTRACTING RATIONAL NUMBERS Evaluate the expression for the given value of the variable. B. 9 y for y = 4 9 y 9 ( 4) Substitute 4 for y. = 9 + 4 Add the opposite of 4. = 5 9 > 4; use the sign of 9.

10. SUBTRACTING RATIONAL NUMBERS Your Turn! Evaluate the expression for the given value of the variable. A. 11 m for m = 3 11 m 11 ( 3) Substitute 3 for m. = 11 + 3 Add the opposite of 3. = 14 Same sign; use the sign of the integers.

11. SUBTRACTING RATIONAL NUMBERS Your Turn! Evaluate the expression for the given value of the variable. B. 5 r for r = 2 5 r 5 ( 2) Substitute 2 for r. = 5 + 2 Add the opposite of 2. = 3 5 > 2; use the sign of 5.

12. SUBTRACTING RATIONAL NUMBERS Architecture Application The top of the Sears Tower in Chicago, is 1454 feet above street level, while the lowest level is 43 feet below street level. How far is it from the lowest level to the top? Subtract the lowest level from the height. 1454 ( 43) Add the opposite of ( 43). 1454 + 43 Same sign; use the sign of the integers. = 1497 It is 1497 feet from the lowest level to the top.

13. SUBTRACTING RATIONAL NUMBERS Your Turn! The distance from the high dive to the swimming pool is 25 feet. The pool is 14 feet deep. What is the total distance from the high dive to the bottom of the pool? Subtract the depth of the pool from the height of the high dive. 25 ( 14) Add the opposite of ( 14). 25 + 14 = 39 Same sign; use the sign of the integers. It is 39 feet from the diving board to the bottom of the pool.

14. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. a. n = 6 (-4)

15. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. a. n = 6 (-4) n = 6 (-4) To subtract 4, add 4

16. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. a. n = 6 (-4) n = 6 (-4) n = 6 + 4 To subtract 4, add 4

17. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. a. n = 6 (-4) n = 6 (-4) n = 6 + 4 n = 10 To subtract 4, add 4

18. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. b. (-3) (-7) = y

19. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. b. (-3) (-7) = y (-3) (-7) = y To subtract 7, add 7

20. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. b. (-3) (-7) = y (-3) (-7) = y (-3) + 7 = y To subtract 7, add 7

21. SUBTRACTING RATIONAL NUMBERS Example Solve each equation. b. (-3) (-7) = y (-3) (-7) = y (-3) + 7 = y 4 = y To subtract 7, add 7

22. STUDENT ACTIVITY You will now receive a worksheet. Turn the worksheet in when completed.

23. Do Not Disturb Work In Progress