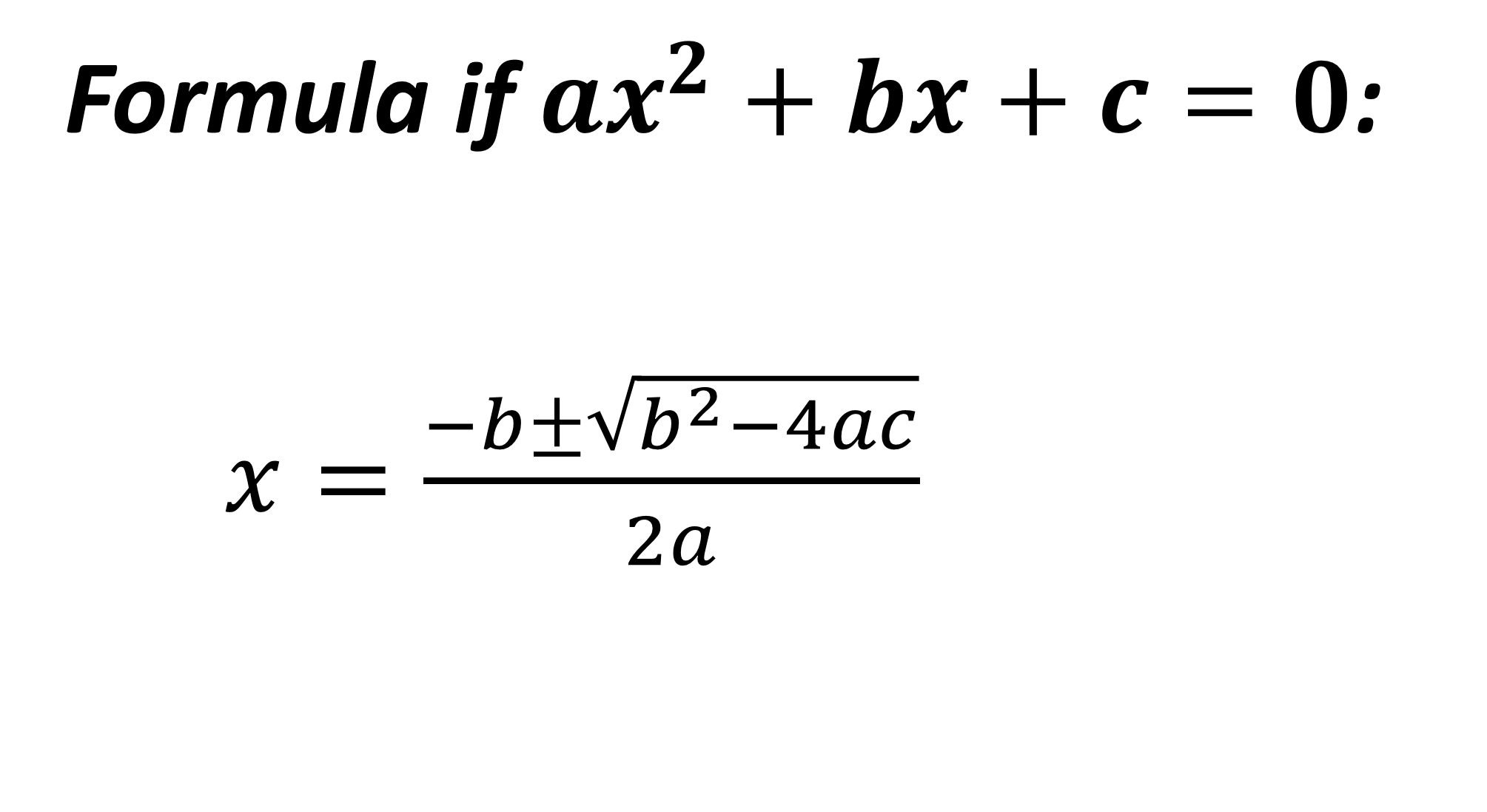Quadratic Formula and Discriminant in Mathematics
9-6
The Quadratic
Formula and the
Discriminant
The Quadratic Formula
Problem 1: Using the Quadratic Formula
Using the Discriminant
This part right
here
Problem 4: Using the Discriminant
Quadratic formula and discriminant in algebraic equations. Understand how to find solutions using the quadratic formula and analyze the number of real solutions using the discriminant. Discover the role of discriminant in determining the nature of roots in quadratic equations.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
9-6 The Quadratic Formula and the Discriminant
The Quadratic Formula Formula if ???+ ?? + ? = ?: ? = ? ?2 4?? 2?
Problem 1: Using the Quadratic Formula What are the solutions of ?2 2? 8 = 0? Use the Quadratic Formula.
What are the solutions of ?2 4? 21 = 0? Use the Quadratic Formula.
Using the Discriminant The discriminant is the expression under the radical sign in the quadratic formula ? = ? ?? ??? This part right here 2?
Problem 4: Using the Discriminant How many real number solutions does this equation have? 2?2 3? + 5 = 0
How many real number solutions does this equation have? 6?2 5? 7 = 0
