Probability Practice Questions: Independent and Dependent Events
Explore a series of practice questions to distinguish between independent and dependent events in probability scenarios. Topics covered include random selections of candies, cards, coins, children's genders, and flowers. Additionally, calculations for specific probabilities involving dice rolls and mug selections are illustrated.
Uploaded on Sep 13, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
CHAPTER 19 Probability II Solutions: Practice Questions 19.2
19 Practice Questions 19.2 1. Decide whether these events are independent or dependent. You have a jar with 24 chocolates and 14 jellies. You take one sweet at random from the jar, eat it, and then take a second sweet at random from the jar. (i) Dependent: The sweet is not replaced so there are fewer sweets to choose from on the next pick. Derek has a blue, a red, and a green tie. He also has a blue and a green shirt. He chooses a random tie and shirt for work today. (ii) Independent: The choice of tie does not affect the choice of shirts.
19 Practice Questions 19.2 1. Decide whether these events are independent or dependent. Amy plays card games. She picks a card at random. Then without putting the first card back, she picks a second card at random. (iii) Dependent: The card is not replaced so there are fewer cards to choose from on the next pick. Ian has 14 coins. He takes three of them at random, then he puts these back, and then picks two more coins at random. (iv) Independent: The coins are replaced so there are the same numbers of coins to pick from each time.
19 Practice Questions 19.2 1. Decide whether these events are independent or dependent. Jeff has three children. His first two children are boys. His last child is a girl. (v) Independent: Each child s gender is not affected by the gender of the others. A plant has four red flowers and two blue flowers. Brenda picks one flower from the plant. After some time, her sister picks a flower from the same plant. (vi) Dependent: The flowers aren t replaced, so there are fewer flowers to choose from the next pick.
19 Practice Questions 19.2 2. You roll a fair six-sided die twice. What is the probability the first roll shows a five and the second roll shows a six? P(5 on 1st Roll and 6 on 2nd Roll) P(5 and 6) and means multiply = P(5) P(6) =1 6 1 6 =1 36
19 Practice Questions 19.2 3. There are nine mugs in Tom s cupboard, four blue and five green. He randomly selects one to use on Monday, washes it and replaces it. He randomly selects another on Tuesday. What is the probability that: he uses a blue mug both days? (i) There are 4 blue mugs and 5 green mugs giving a total of 9 mugs. P(Blue on Monday and Blue on Tuesday) P(Blue and Blue) = P(Blue) P(Blue) =4 9 4 =16 81 9
19 Practice Questions 19.2 3. You There are nine mugs in Tom s cupboard, four blue and five green. He randomly selects one to use on Monday, washes it and replaces it. He randomly selects another on Tuesday. What is the probability that: he uses a blue mug on Monday and a green mug on Tuesday? (ii) P(Blue on Monday and Green on Tuesday) P(Blue and Green) = P(Blue) P(Green) =4 9 5 9 =20 81
19 Practice Questions 19.2 4. Shannon spins a spinner numbered 1 to 7, and tosses a coin. What is the probability she gets: an odd number on the spinner? (i) P(Odd) =4 7
19 Practice Questions 19.2 4. Shannon spins a spinner numbered 1 to 7, and tosses a coin. What is the probability she gets: an odd number on the spinner and a tail on the tail? (ii) P(Odd and Tail) = P(Odd) P(Tail) =4 7 1 =4 14 2 =2 7
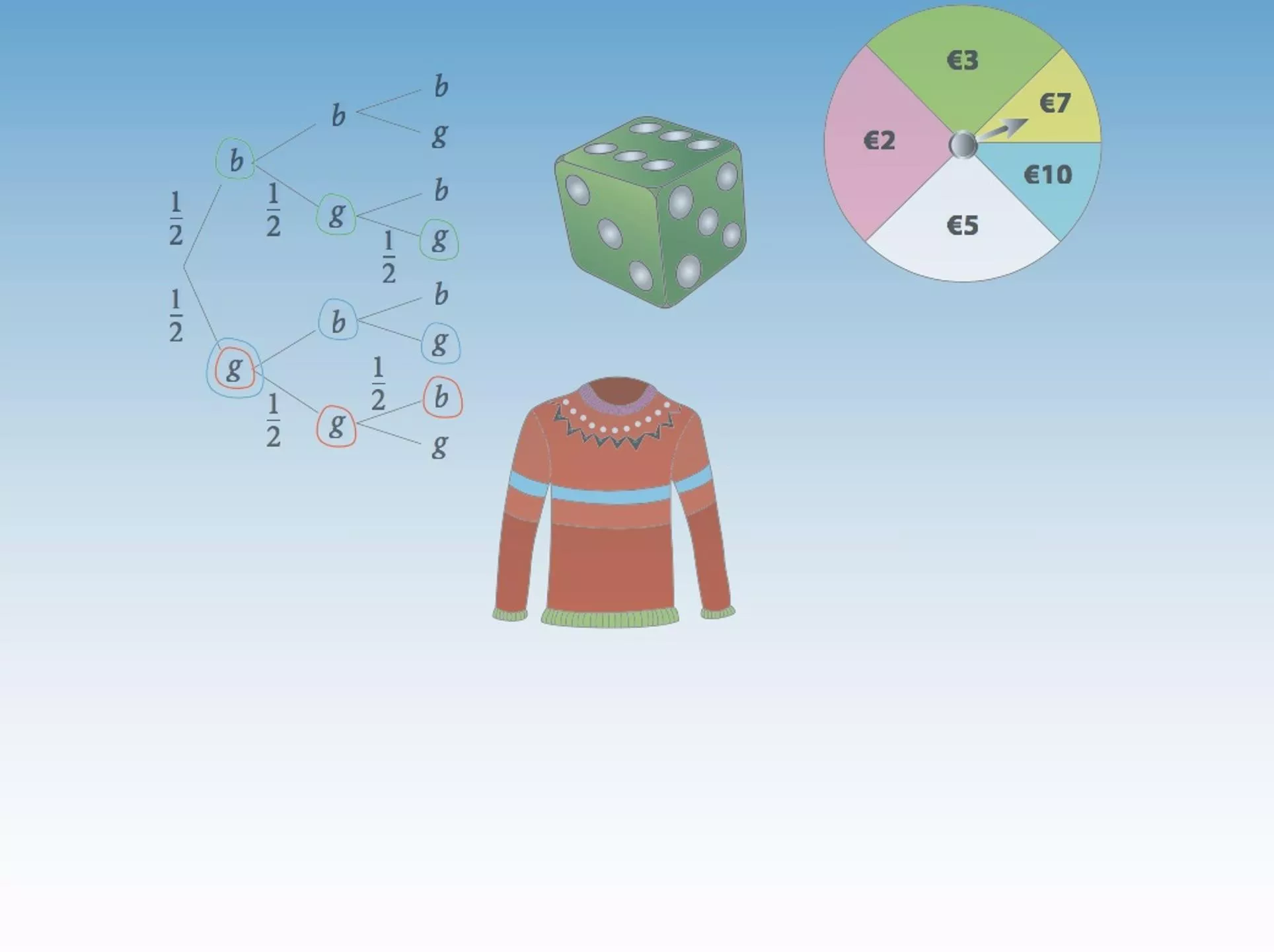
19 Practice Questions 19.2 5. You flip a coin and then roll a fair six-sided die. Draw a tree diagram to represent this situation. (i) Write the probabilities on each branch.
19 Practice Questions 19.2 5. You flip a coin and then roll a fair six-sided die. Use the tree diagram to calculate the probability that: the coin lands heads-up and the die shows a three? (ii) P(Head and 3) = P(Head) P(3) 2 1 =1 12 =1 6
19 Practice Questions 19.2 5. You flip a coin and then roll a fair six-sided die. Use the tree diagram to calculate the probability that: the coin lands tails-up and the die shows a number greater than four? (iii) P(Tail and 5, 6) = P(Tail) P(5 or 6) 2 2 =2 12 =1 6 =1 6
19 Practice Questions 19.2 5. You flip a coin and then roll a fair six-sided die. Use the tree diagram to calculate the probability that: the coin lands heads-up and the die shows an even number? (iv) P(Head and 2, 4, 6) = P(Head) P(2 or 4 or 6) 2 3 =3 12 =1 6 =1 4
19 Practice Questions 19.2 5. You flip a coin and then roll a fair six-sided die. Use the tree diagram to calculate the probability that: the coin lands heads-up and the die shows a prime number? (v) P(Head and 2, 3, 5) = P(Head) P(2 or 3 or 5) 2 3 =3 12 =1 6 =1 4
19 Practice Questions 19.2 6. The names of 9 boys and 11 girls from your class are put into a hat. Two names are selected without replacement. Draw a tree diagram to represent this situation. (i) Write the probabilities on each branch.
19 Practice Questions 19.2 6. The names of 9 boys and 11 girls from your class are put into a hat. Two names are selected without replacement. What is the probability that: the two names chosen will both be girls? (ii) There are 9 boys and 11 girls so there are 20 students in total. P(1stis a girl and 2ndis a girl) = P(Girl) P(Girl) 11 10 20 19 11 38 1 girl gone 1 person gone =
19 Practice Questions 19.2 6. The names of 9 boys and 11 girls from your class are put into a hat. Two names are selected without replacement. What is the probability that: the first name will be a boy and the second will be a girl? (iii) P(1stis a boy and 2ndis a girl) = P(Boys) P(Girls) 9 20 19 99 380 11 1 person gone =
19 Practice Questions 19.2 6. The names of 9 boys and 11 girls from your class are put into a hat. Two names are selected without replacement. What is the probability that: both names will be boys? (iv) P(1stis a boy and 2ndis a boy) = P(Boys) P(Boys) 9 20 19 8 1 boy gone 1 person gone 18 95 =
19 Practice Questions 19.2 7. A card is chosen at random from a standard deck of 52 playing cards. Without replacing it, a second card is chosen. What is the probability that the first card chosen is a queen and the second card chosen is a jack? P(1stis a Queen and 2ndis a Jack) = P(Queen) P(Jack) 4 4 51 = 52 1 card gone 16 663 =
19 Practice Questions 19.2 8. A bowl of fruit is on the table. It contains four apples, six oranges and three bananas. Alan and Kenneth come home from school and randomly grab one fruit each. What is the probability that both grab apples? There are 4 apples, 6 oranges and 3 bananas so there are 13 pieces of fruit in total. P(Apple and Apple) = P(Apple) P(Apple) 1 apple gone 1 piece of fruit less 4 3 = 13 12 1 13 =
19 Practice Questions 19.2 9. Your drawer contains 11 red socks and six blue socks. It s too dark to see which are which, but you grab two anyway. Draw a tree diagram to represent this information. Write the probabilities on each branch. (i)
19 Practice Questions 19.2 9. Your drawer contains 11 red socks and six blue socks. It s too dark to see which are which, but you grab two anyway. What is the probability that: the first sock is red and the second is blue? (ii) P(1st is Red and 2nd is Blue) = P(Red) P(Blue) 11 17 16 33 136 6 = 1 sock less =
19 Practice Questions 19.2 9. Your drawer contains 11 red socks and six blue socks. It s too dark to see which are which, but you grab two anyway. What is the probability that: the first sock is blue and the second is red? (iii) P(1st is Blue and 2nd is Red) = P(Blue) P(Red) 6 11 = 17 16 33 136 =
19 Practice Questions 19.2 9. Your drawer contains 11 red socks and six blue socks. It s too dark to see which are which, but you grab two anyway. What is the probability that: both socks are blue? (iv) P(Blue and Blue) = P(Blue) P(Blue) 6 5 = 17 16 15 136 =
19 Practice Questions 19.2 9. Your drawer contains 11 red socks and six blue socks. It s too dark to see which are which, but you grab two anyway. What is the probability that: both socks are red? (v) P(Red and Red) = P(Red) P(Red) 11 10 17 16 55 136 = =
19 Practice Questions 19.2 10. The game of backgammon uses two standard dice, each with the numbers one through six. You need to roll double twos to win the game. What is the probability you will get that result on your next roll? P(2 and 2) = P(2) P(2) 1 1 6 6 1 36 = =
19 Practice Questions 19.2 11. I roll three dice. Are these events dependent or independent? (i) These events are independent because the result of rolling each die does not influence the next die.
19 Practice Questions 19.2 11. I roll three dice. What is the probability that I get three ones? (ii) P(1 and 1 and 1) = P(1) P(1) P(1) 1 6 1 6 1 6 = 1 = 216
19 Practice Questions 19.2 12. I draw a card from a standard deck, replace it, and draw another. Are these events dependent or independent? (i) These events are independent because the card is replaced so there are the same number of cards to pick from on the second draw.
19 Practice Questions 19.2 12. I draw a card from a standard deck, replace it, and draw another. What is the probability I get two aces? (ii) P(Ace and Ace) = P(Ace) P(Ace) 4 4 = 52 16 2704 1 169 52 = =
19 Practice Questions 19.2 13. Four cards are chosen at random from a deck of 52 cards, one after the other, without replacement. Are these events dependent or independent? (i) These events are dependent because the cards are not replaced so there are fewer cards to pick from each time.
19 Practice Questions 19.2 13. Four cards are chosen at random from a deck of 52 cards, one after the other, without replacement. What is the probability of choosing a ten, a nine, an eight and a seven in that order? (ii) P(10 and 9 and 8 and 7) = P(10) P(9) P(8) P(7) =4 52 4 32 812175 51 4 50 4 49 =
19 Practice Questions 19.2 14. Henry has three black shirts and seven blue shirts in his wardrobe. Two shirts are drawn without replacement from the wardrobe. What is the probability that both of the shirts are black? There are 3 black shirts and 7 blue shirts so a total of 10 shirts. P(Black and black) = P(Black) P(Black) =3 10 2 =6 90 9 =1 15
19 Practice Questions 19.2 15. An archer always hits a circular target with each arrow shot. He hits the bullseye two out of every five shots on average. If he takes three shots at the target, calculate the probability that he hits the bullseye: every time (i) P(Bullseye and Bullseye and Bullseye) Every time : = P(Bullseye) P(Bullseye) P(Bullseye) =2 5 2 8 125 5 2 5 =
19 Practice Questions 19.2 15. An archer always hits a circular target with each arrow shot. He hits the bullseye two out of every five shots on average. If he takes three shots at the target, calculate the probability that he hits the bullseye: the first two times but not the third (ii) P(Bullseye and Bullseye and no Bullseye) = P(Bullseye) P(Bullseye) P(No Bullseye) P(No Bullseye) = 1 P(Bullseye) =1-2 5 =3 5
19 Practice Questions 19.2 15. An archer always hits a circular target with each arrow shot. He hits the bullseye two out of every five shots on average. If he takes three shots at the target, calculate the probability that he hits the bullseye: the first two times but not the third (ii) P(Bullseye and Bullseye and no Bullseye) = P(Bullseye) P(Bullseye) P(No Bullseye) P(Bullseye and Bullseye and no Bullseye) 2 5 2 5 3 5 = =12 125
19 Practice Questions 19.2 15. An archer always hits a circular target with each arrow shot. He hits the bullseye two out of every five shots on average. If he takes three shots at the target, calculate the probability that he hits the bullseye: on no occasion. (iii) P(No Bullseye and no Bullseye and no Bullseye) = P(No Bullseye) (no Bullseye) (no Bullseye) =3 5 3 =27 125 5 3 5
19 Practice Questions 19.2 16. A class has 14 boys and 16 girls. Three students are chosen at random to represent the class at a school event. What is the probability that: they are all girls? (i) There are 14 boys and 16 girls so 30 students in total. P(all girls) = P(Girl) P(Girl) P(Girl) =16 30 15 =4 29 29 14 (Dependent events) 28
19 Practice Questions 19.2 16. A class has 14 boys and 16 girls. Three students are chosen at random to represent the class at a school event. What is the probability that: they are all boys? (ii) P(all boys) = P(Boy) P(Boy) P(Boy) =14 30 13 =13 145 29 12 28
19 Practice Questions 19.2 17. Emma says that it is very difficult to get five heads in a row when you flip a coin. Brian disagrees and says he has done it loads of times. Which person do you agree with? (i) I agree with Emma as the probability is low of this happening. 1 32
19 Practice Questions 19.2 17. Emma says that it is very difficult to get five heads in a row when you flip a coin. Brian disagrees and says he has done it loads of times. Neither of them have a coin to try it so they decide to work the chances out mathematically. Show the calculations they would use to do this. (ii) P(Head and Head and Head and Head and Head) 5 heads in a row : = P(Head) P(Head) P(Head) P(Head) P(Head) =1 2 1 =1 32 2 1 2 1 2 1 2
19 Practice Questions 19.2 17. Emma says that it is very difficult to get five heads in a row when you flip a coin. Brian disagrees and says he has done it loads of times. Neither of them have a coin to try it so they decide to work the chances out mathematically. Do you want to change your answer to (i) above? Justify your answer using the calculations in (ii) above. (iii) 1 The probability of this happening is only which means once in 32 attempts. 32