Power System Fault Calculation and Protection Analysis

In this technical document, we delve into the calculation of fault current and fault apparent power in symmetrical three-phase short circuit scenarios within power systems. Through detailed equivalent circuit diagrams, reactance calculations, and per unit value derivations, the fault current and apparent power are accurately determined for the given configurations. Additionally, a practical case study involving turbo generators, transformers, and busbars is explored to analyze fault currents and circuit breaker ratings. The content provides valuable insights into power system protection and fault analysis methodologies.

• Power Systems
• Fault Calculation
• Protection Analysis
• Equivalent Circuit
• Fault Current

karter Follow

Uploaded on Apr 18, 2024 | 5 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Exercise 5 Power systems

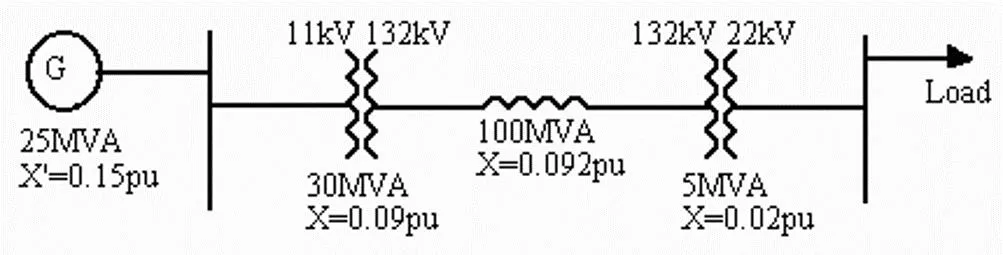
2. Question 1 A symmetrical three-phase short circuit occurs on the 22kV busbars of the circuit shown as a one-line diagram in the figure below. Calculate the fault current and the fault apparent power.

3. Un1,t2 Un2,t1 Question 1 Sn 1. Selecting base power ??= 100 MVA 2. Drawing the equivalent circuit with reactances 3. Calculating Per Unit (p.u.) values for every component 4. Calculating the ???and ??? Zk ??= 100 MVA Equivalent circuit: xt2 xg xt1 xl 2 U n Z Note: this simplifies calculation if Un2,t1 = Un1,t2 k 2 S 2 S U S X = if select ed U U n b n 2 b = = = = x Z x Z b n u . . u . . p k p k Z S S U U b N n b b S b

4. Question 1 Sn 1. Selecting base power ??= 100 MVA 2. Drawing the equivalent circuit with reactances 3. Calculating Per Unit (p.u.) values for every component 4. Calculating the ???and ??? Zk xt2 xg xt1 xl S b = x Z u . . p k S n Name Calculation Reactance Generator (??) 0.6 p.u. ?0.15 100/25 xT Transformer 1 (11/132) (??1) 0.3 p.u. ?0.09 100/30 Transformer 2 (132/22) (??2) 0.4 p.u. ?0.02 100/5 Line (??) ?0.092 100/100 0.092 p.u ??= ??+ ??1+??2+??=1.392

5. Question 1 1. Selecting base power ??= 100 MVA 2. Drawing the equivalent circuit with reactance 3. Calculating Per Unit (p.u.) values for every component 4. Calculating the ???and ??? ??= 100 MVA 2 U sc Z S Equivalent circuit: sc sc 2 = = = x z , u . , u . . T p sc p Z U XT b b S b S = = calculated we presuming that x U U U b = x T, p.u b n sc , u . T p S sc ???=?? 100 1.392= 71.8 MVA = ?? ???=?? ?? 100 = = 3 22 1.392= 1.885 kA ?? 3 ?? ??

6. Question 2 Two 100-MVA, 20-kV turbo generators (each of transient reactance 0.2 pu) are connected, each through its own 100- MVA, 0.1 pu reactance transformer, to a common 132-kV busbar. From this busbar, a 132-kV feeder, 40 km in length, supplies an 11-kV load through a 132/11-kV transformer of 200 MVA rating and reactance 0.1 pu. If a balanced three- phase short circuit occurs on the low voltage terminals of the load transformer, determine, using a 100-MVA base, the fault current in the feeder and the rating of a suitable circuit breaker at the load end of the feeder. The feeder impedance per phase is (0.035+j0.14) /km.

7. Question 2

8. Question 2 1. Selecting base power ??= 100 MVA 2. Drawing the equivalent circuit with reactance 3. Calculating Per Unit (p.u.) values for every component 4. Simplifying the circuit 5. Calculating the ???and ??? xg1 xt1 xl xt3 Equivalent circuit: xg2 xt2 ?? ?? Calculating reactance of the line (feeder):??= Line impedance ??= 40 0.035 + ?0.14 = 1.4 + ?5.6 = 5.77 < 76 Base impedance ??= ?? ??=5.77<76 2 ?? ??=1322 174.24= 0.033 < 76 ?.?. 100= 174.24 Per Unit value ??=

9. Question 2 3. Calculating Per Unit (p.u.) values for every component 4. Simplifying the circuit 5. Calculating the ???and ??? S b = x Z u . . p k S j0.1 n j0.2 Name Generator 1(??1) Calculation ?0.20 100/100 Impedance j0.2 p.u. 0.033< 76 j0.2 j0.1 j0.05 Generator2(??2) ?0.20 100/100 j0.2 p.u. Transformer1(??1) ?0.10 100/100 j0.1 p.u. Transformer2(??2) ?0.10 100/100 j0.1 p.u. j0.3 Transformer3(??3) ?0.10 100/200 j0.05 p.u. Line(feeder)(??) 0.033< 76 p.u. ??/?? j0.3 0.0824 < 84.44

10. Question 2 4. Simplifying the circuit 5. Calculating the ???and ??? j0.3 j0.3 Parallel: ???=?0.3 ?0.3 ?0.3+?0.3= ?0.15?.?. 0.0824 < 84.44 j0.15 0.0824< 84.44 ??= 0.232 < 88 ?.?. ??= 0.232?.?. ???=?? ?? 100 = = 3 11 0.232= 22.62 kA ?? 3 ?? ?? 100 0.232= 431 MVA 0.232 < 88 ???=?? = ??

11. Question 3 A single line-to-earth fault occurs in a radial transmission system. The following sequences exist between the source of supply (an infinite busbar) of voltage 1 pu to the point of the fault: Z1= (0.3+j0.6)pu, Z2= (0.3+j0.55)pu, Z0= (1+j0.78)pu. The fault path to earth has a resistance of 0.66 pu. Determine the fault current and the voltage at the point of the fault.

12. Some background first Question 3 (W 7.8) IS =0 (= IL2) IT=0 (= IL3) Inverse matrix also provides the same solution

13. Question 3 Generally: Z U1 U2 U0 3ZM ZM From previous slide: and IL1=3 I0

14. Question 3 (W 7.8) in one-phase earth fault =IL1

15. Question 3 So we can utilize the following equations: 3 + 3 1 U = = = = = . 0 649 035 . . 0 738 28 3 . I I j ( . 3 ) 1 f + 58 j . 1 93 + + 3 Z 738 Z Z Z 1 2 0 f = . 0 I pu f ( pu ) ( . 0 ) = = = = . 0 649 j . 0 35 . 0 66 43 j . 0 23 . 0 487 28 3 . U I Z pu f f f = . 0 487 U f