Geometry Formulas and Examples for Midpoint and Distance Calculations

Explore the midpoint and distance formulas in geometry with detailed explanations and examples. Learn how to find midpoints, endpoints, and distances between points on a coordinate plane. Enhance your understanding of segment bisectors and midpoint calculations through practical illustrations.

• Geometry
• Formulas
• Midpoint
• Distance
• Examples

alexia Follow

Uploaded on Aug 27, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

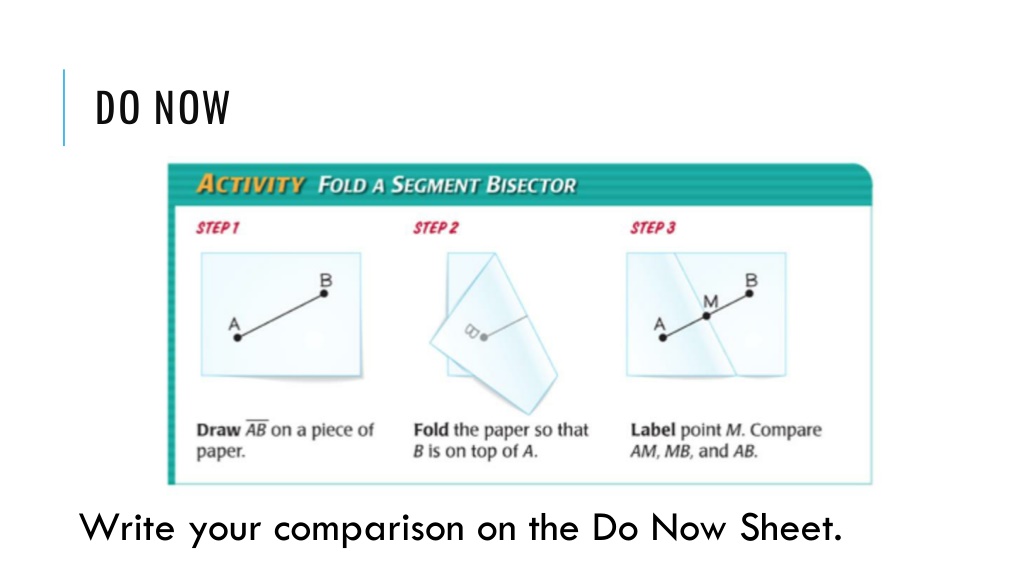
1. DO NOW Write your comparison on the Do Now Sheet.

2. SECTION 1.3-USE MIDPOINT AND DISTANCE FORMULAS Geometry

3. VOCABULARY Segment bisector: a point, ray, line, line segment, or plane that intersects the segment at its midpoint Midpoint: point that divides the segment into two congruent segments

4. EXAMPLE 1 Skateboard In the skateboard design, VWbisects XYat point T, and XT=39.9cm. Find XY. Point Tis the midpoint of XY . So, XT = TY = 39.9cm. XY = XT + TY = 39.9 + 39.9 = 79.8cm

5. EXAMPLE 2 Point Mis the midpoint of VW. Find the length of VM . STEP 1: Write and solve an equation. Use the fact that VM = MW. STEP 2: Evaluate the expression for VM when x =4. VM = 4x 1 = 4(4) 1 = 15 VM = MW 4x 1 = 3x + 3 So, the length of VMis 15. x 1 = 3 *** Do a mental check for MW. x = 4

6. THE MIDPOINT FORMULA If ? ?1,?1 and B ?2,?2 are points in a coordinate plane, then the midpoint M of ??has coordinates ?1+?2 2 2 ,?1+?2 .

7. EXAMPLE 3 (A) a.FIND MIDPOINT The endpoints ofRSare R(1, 3) and S(4, 2). Find the coordinates of the midpoint M. 3 + 2 2 1 + 4 2 5 2 1 , , M M = 2 The coordinates of the midpoint M are ? ?, ? ?.

8. EXAMPLE 3 (B) b.FIND ENDPOINT The midpoint of JKis M(2, 1). One endpoint is J(1, 4). Find the coordinates of endpoint K. STEP 1 Find x. 1+ x 2 STEP 2 Find y. 2 = 4+ y 2 4 + y = 2 1 = 1 + x = 4 x =3 y = 2 The coordinates of endpoint Kare (3, 2).

9. THE DISTANCE FORMULA If ? ?1,?1 and ? ?2,?2 are points in a coordinate plane, then the distance between A and B is ?? = ?2 ?1 2+ ?2 ?1 2.

10. EXAMPLE 4-STANDARDIZED TEST PREP Use the distance formula

11. EXAMPLE 4 CONTINUED 2+ ?2 ?1 2 ?? = ?2 ?1 4 22+ 1 32 = 22+ ( 4)2 = = 4 + 16 = 20 4.47 The correct answer is C.

12. HOMEWORK Textbook pages 19-20 # 5, 9, 12, 20, 23, 25, 32, 34