Exploring Properties of Parallelograms, Rhombuses, and Rectangles
Delve into the theorems and examples related to the properties of parallelograms, rhombuses, and rectangles. Understand concepts such as diagonals in rhombuses, perpendicular diagonals in parallelograms, and congruent diagonals in rectangles. Learn to identify these shapes based on specific criteria and explore the unique properties that define each geometric figure.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
6 6- -4 SPECIAL 4 SPECIAL PARALLELOGRAMS PARALLELOGRAMS GEOMETRY
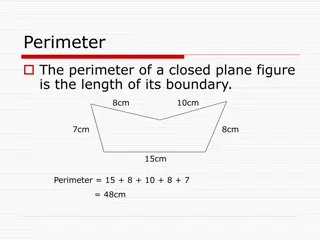
Theorem 6-9: Each diagonal of a rhombus bisects two angles of the rhombus. 1 2 3 4 5 6 7 8
Theorem 6 Theorem 6- -10: perpendicular. perpendicular. 10: The diagonals of a rhombus are The diagonals of a rhombus are ?? ??
Example 1 Example 1
Example 2 Example 2
Theorem 6 Theorem 6- -11: are congruent. are congruent. 11: The diagonals of a rectangle The diagonals of a rectangle ?? ??
Example 3 Example 3
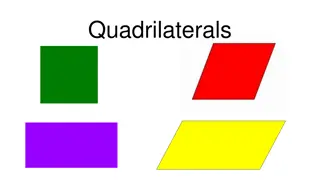
Identifying a Rhombus Identifying a Rhombus Theorem 6-12: If one diagonal of a parallelogram bisects two angles of the parallelogram, then the parallelogram is a rhombus. Theorem 6-13: If the diagonals of a parallelogram are perpendicular, then the parallelogram is a rhombus. Identifying a Rectangle Identifying a Rectangle Theorem 6-14: If the diagonals of a parallelogram are congruent, then the parallelogram is a rectangle.
Example 4 Example 4
Example 5 Example 5 Rhombus, Rectangle, or Neither? Rhombus, Rectangle, or Neither?
Property Parallelogram Rectangle Rhombus Square Opp. sides are parallel Opp. sides are congruent Opp. <'s are congruent One set of sides is congruent and parallel A diag. forms 2 congruent triangles Diags. bisect each other Diags. are congruent Diags. are perpendicular A diag. bisects two <'s All <'s are right <'s All sides are congruent