Exploring Geometric Concepts in Grade Five Mathematics
Dive into the world of geometry with Grade Five Mathematics! This module covers topics such as shapes classification, properties of two-dimensional and three-dimensional shapes, coordinate systems, polygon construction, and Van Hiele levels of geometric thought. Engage with hands-on activities, visualizations, and vocabulary development to enhance your understanding of geometry concepts.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
1 PARTNERS forMathematicsLearning GradeFive Module4 Partners forMathematicsLearning
2 BigIdeasinGeometry Shapesorgroupsofshapescanbe classifiedbytheirproperties Two-dimensionalshapesarecombined tomakethree-dimensionalshapes Area,perimeter,andvolumesare examplesofmeasurableattributesin geometry Partners forMathematicsLearning
3 BigIdeasinGeometry Shapescanbedescribedintermsoftheir locationandviewedfromdifferent perspectives;geometricfigurescanbe movedinaplanewithoutchangingtheir sizeorshape Coordinatesystemscanbeusedto describelocationsprecisely Partners forMathematicsLearning
4 Shapes&Properties Exploring Attributes ofShapes Refining Concepts Classifying Shapes Developing Vocabulary Partners forMathematicsLearning
5 VanHieleLevelsofGeometricThought Visualization(Recognition) Description/Analysis Abstract/Relational FormalDeduction(highschool geometry) Level4Rigor(collegelevelgeometry) Level0 Level1 Level2 Level3 Partners forMathematicsLearning
6 ShapesandProperties Lookatthisshape Whataresomeofitsproperties? Partners forMathematicsLearning
7 ShapesandProperties Findthesepropertiesinyourenvironment: Parallellines Rightangles Shapeswith dents (concave) Solidslikeacylinder Solidslikeapyramid Shapeswithrotationalsymmetry Partners forMathematicsLearning
8 WhatisaPolygon? AllofthesearePolygons NoneofthesearePolygons Partners forMathematicsLearning
9 WhatisaPolygon? WhichoftheseisaPolygon? Whatattributesdoesapolygonhavethat makesitapolygon? Partners forMathematicsLearning
10 ConstructingPolygons Createapolygontofiteachdescription 4sidesand4rightangles 3sidesand1rightangle 5sides 12sides 4sideswithexactly2sidesparallel 4sideswith2pairsofsidesperpendicular 3sideswith2sidesperpendicular
11 RegularandIrregularPolygons Theseareregularpolygons Theseareirregularpolygons Partners forMathematicsLearning
12 Triangles Sortthetrianglesintothreegroupssothat notwotrianglesbelonginmorethanone group Writeadescriptionofeachgroup Nowsortthetrianglesagainintothree differentgroupssothatnotrianglebelongs intwogroups Writeadescriptionofeachofthesegroups Partners forMathematicsLearning
13 Triangles Fillinthechartbelowwithasketchofa trianglethatfitsbothlabels Areanyimpossibleones? Partners forMathematicsLearning
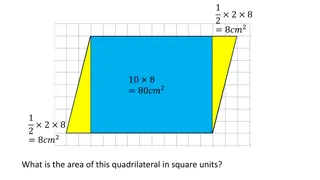
14 Quadrilaterals Findallthequadrilaterals Sortthemintogroups Arethereoverlaps? DrawaVenndiagramtosorttheshapes Canyoufindadifferentwaytosortthem? Partners forMathematicsLearning
15 DiagonalsinQuadrilaterals Drawthediagonalsineachquadrilateral \ Whatpropertiescanyouidentifyinthe diagonals? Partners forMathematicsLearning
16 DiagonalsinQuadrilaterals Parallelogram Rectangle Rhombus Square Trapezoid Kite Properties of Diagonals form congruent triangles bisecteach other are congruent Are perpendicular Bisect opposite angles Partners forMathematicsLearning
17 DiagonalsinQuadrilaterals Parallelogram Rectangle Rhombus Square Trapezoid Kite Properties of Diagonals form congruent triangles bisecteach other are congruent Are perpendicular Bisect opposite angles 1pair yes yes 2pairs (adjacen t) 2opposite pairs 2opposite pairs yes yes yes yes yes yes yes yes yes yes yes yes yes Partners forMathematicsLearning
18 DiagonalsinQuadrilaterals Howmanydiagonalsinthese quadrilaterals? Partners forMathematicsLearning
19 DiagonalsinQuadrilaterals Concavequadrilateralsdohave2 diagonals. Whatisdifferent? Partners forMathematicsLearning
20 Quadrilaterals Lookatthissetofparallelograms. Whatareitspropertiesofsides?angles? symmetry?diagonals? Partners forMathematicsLearning
21 Quadrilaterals Classifythequadrilateralsbylabelingthe partsofthisVenndiagram: Partners forMathematicsLearning
22 Quadrilaterals Partners forMathematicsLearning
23 TrueorFalse? Ifitisasquare,itisalsoarhombus Someparallelogramsarerectangles Allrectanglesaresquares Ifithasexactlytwolinesofsymmetry,then itmustbeaquadrilateral Notriangleshavediagonals Alltriangleshave3congruentsides Alltrapezoidshaveexactly2parallelsides Partners forMathematicsLearning
24 TrueorFalse? Ifitisasquare,itisalsoarhombus.T Someparallelogramsarerectangles.T Allrectanglesaresquares.F Ifithasexactlytwolinesofsymmetry,thenit mustbeaquadrilateral.F Alltriangleshavenodiagonals.T Alltriangleshave3congruentsides.F Alltrapezoidshaveexactly2parallelsides.T Partners forMathematicsLearning
25 AlgebraConnection:Diagonals Howmanydiagonalsinatriangle? aquadrilateral? Apentagon? Anypolygon? Howmanydiagonalsinapolygonwithn sides? What stherule? Partners forMathematicsLearning
26 Angles D B E A F Whatareangles? Whatmakesconceptualizingthesizeofan anglechallengingforstudents? Partners forMathematicsLearning
27 Angles Theprotractorisoneofthemostpoorly understoodmeasuringinstrumentsin school. JohnVandeWalle Whyismeasuringthesizeofanangleso difficultforstudents? Partners forMathematicsLearning
28 ChallengesofaProtractor Units(degrees)areverysmall Noanglesareshownonprotractor;only littlemarksaroundtheedge Numbersgobothclockwiseand counterclockwiseonatypicalprotractor Partners forMathematicsLearning
29 AngleSize Studentsneedtopracticetellingthe differencebetweenasmallandalarge anglepriortomeasuringangles Whatactivitiesmightprovidethis? Partners forMathematicsLearning
30 ReadingaProtractor Howdoyouhelpstudentsunderstandhow touseatypicalprotractor? Whatexperiencesneedtocomebefore studentstrytouseaprotractor? Partners forMathematicsLearning
31 AngleUnits Useastraightedgetodrawanarrowangle onyourcard Cutitout Usethewedgeasaunittomeasure angles,countingthenumberofwedges thatfitintoaparticularangle Partners forMathematicsLearning
32 MakingaProtractor Foldthepieceofwaxedpaperinhalf,creasing thefoldtightly Foldinhalfagainsothatthefoldededges match Foldalongthediagonalfromthefoldedcorner Foldagainfromthefoldedcornertobring togetherthetwosidesthatformthefolded corner Cutortearofftheedgeabout4-5inchesfrom thevertexandunfold Partners forMathematicsLearning
33 MakingaToolforMeasuringAngles Compareyourwaxedpapertooltoa traditionalprotractor Partners forMathematicsLearning
34 MeasuringAngles Makeyouranswercard Decideontheangleyouwanttouse Drawanangleontheindexcard, measuringverycarefully Plangood incorrect answers Placetheanswerchoicesinappropriate locations Partners forMathematicsLearning
35 MeasurementandGeometry Howdoesgeometryoverlapwith measuringangles? Partners forMathematicsLearning
36 Measurement Perhapsthebiggesterrorinmeasurement instructionisthefailuretorecognizeand separatetwotypesofobjectives:first, understandingthemeaningandtechnique ofmeasuringaparticularattributeunitand, second,learningaboutthestandardunits commonlyusedtomeasurethatattribute. JohnVandeWalle,TeachingStudent-CenteredMathematics Partners forMathematicsLearning
37 Triangles Useastraightedgetomakealarge triangle Placeavisibledotoneachvertex Ripoffeachoftheangles Carefullyjointheanglesatthedotsand tapeorgluethemdown Whatdoyounotice? Partners forMathematicsLearning
38 AngleSums Measureeachangleofeachpolygon Recordyourfindingsintheappropriate chart Findthesumoftheanglesforeachfigure Compareyourresultstothoseofyour classmates Whatconclusion(s)aboutthesumofthe anglesdoyoudrawfromtheresults? Partners forMathematicsLearning
39 AngleSums Thesumoftheanglemeasuresofa triangleis180 Partners forMathematicsLearning
40 AngleSums Thesumoftheanglemeasuresofany quadrilateralis360 Anyquadrilateralcanbedividedintotwo triangles,eachwithananglesumor180 Partners forMathematicsLearning
41 AngleSums Divideapentagonintotriangles Useonlydiagonallinesanddonotcrossany lines Howmanytrianglesdidyoumake? Howdoesthatimpactthesumoftheanglesof thepentagon? Dothesamewithahexagon Whatisthepattern? Partners forMathematicsLearning
42 DescribethePolygon Createa Wanted posterforoneofthe culprits below: Arightscalenetriangle Arhombus Atrapezoid Anobtuseisoscelestriangle Aregularpentagon Partners forMathematicsLearning
43 CreatingNetsfor3-DShapes Apentominoismadefrom5congruent squares,withsquarestouchingonlybya wholeside( edgetoedgeconstruction ) Makeasmanydifferentpentominoshapes asyoucan.Howmanycanyoumake? OKNotOK Partners forMathematicsLearning
44 CreatingNetsfor3-DShapes Arethesepentominoesdifferentor congruent? Partners forMathematicsLearning
45 CreatingNetsfor3-DShapes Whichofthepentominopiecescanbe foldedintoatoplessbox? Whatrectanglescanbecreatedfromthe pentominopiecesthatcanbefoldedintoa toplessbox? Partners forMathematicsLearning
46 AreaandPerimeter Whatistherelationship betweentheareaofthe T-shapednetandthesurfaceareaofthe toplessboxitcreates? Aretheperimeterofthenetandthesumof thelengthsoftheedgesofthe3-Dtopless boxalsoequivalent? Partners forMathematicsLearning
47 Volume:RectangularPrisms Usecentimetercubestocreaterectangular prisms Howmanydifferentprismscanyoumake withthesecubes: 24 32 Recordthedimensionsofeachprismthat youmakeandthetotalnumberofcubes used 36 Partners forMathematicsLearning
48 Connections Whichoftheprocessstandardsdidwe use? ProblemSolving ReasoningandProof Communication Connections Representation InGrade5whatarestrongconnections betweengeometryandmeasurement? Partners forMathematicsLearning
DPIMathematicsStaff EverlyBroadway,ChiefConsultant ReneeCunninghamKittyRutherford RobinBarbourMaryH.Russell CarmellaFairJohannahMaynor AmySmith PartnersforMathematicsLearningisaMathematics-Science PartnershipProjectfundedbytheNCDepartmentofPublicInstruction. Permissionisgrantedfortheuseofthesematerialsinprofessional developmentinNorthCarolinaPartnersschooldistricts. Partners forMathematicsLearning
PMLDisseminationConsultants SusanAllman JuliaCazin RuafikaCobb AnnaCorbett GailCotton JeanetteCox LeanneDaughtry LisaDavis RyanDougherty ShakilaFaqih PatriciaEssick DonnaGodley ShanaRunge YolandaSawyer PennyShockley PatSickles NancyTeague MichelleTucker KanekaTurner BobVorbroker JanWessell DanielWicks CarolWilliams StacyWozny CaraGordon TeryGunter BarbaraHardy KathyHarris JulieKolb ReneeMatney TinaMcSwain MarilynMichue AmandaNorthrup KayonnaPitchford RonPowell SusanRiddle JudithRucker Partners forMathematicsLearning