Chemical Kinetics and Numerical Solvers in Chemistry
Explore the principles of chemical kinetics and the use of numerical solvers to compute concentrations over time, considering explicit and implicit methods. Understand stability and positivity requirements in solvers and the importance of characteristic time scales in chemical systems. Dive into a specific example involving a CH4-CO-OH chemical system.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
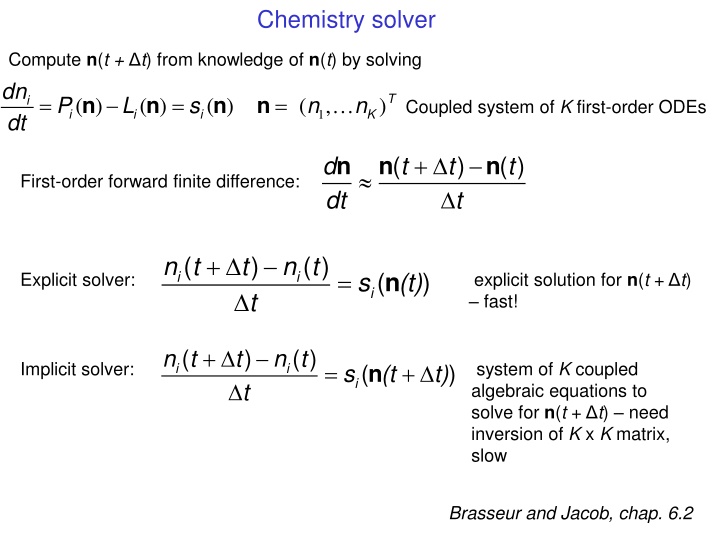
Chemistry solver Compute n(t + t) from knowledge of n(t) by solving d dt n = = = T P L s n n ( ) n ( ) n ( ) n n i ( , ) Coupled system of K first-order ODEs i i i K 1 + d dt ( t t ) t ( ) t n n n First-order forward finite difference: + n t ( t ) t n t ( ) = s ( (t) ) n i i Explicit solver: explicit solution for n(t + t) fast! i + n t ( t ) t n t ( ) Implicit solver: system of K coupled algebraic equations to solve for n(t + t) need inversion of K x K matrix, slow = + s ( (t t) ) n i i i Brasseur and Jacob, chap. 6.2
Stability and positivity in explicit and implicit solvers Consider single species with first-order decay: = dn dt kn / = k t n t n Exact solution over time step t : ( ) (0)exp[ ] but let s solve it numerically First-order implicit solution First-order explicit solution ) ) n ( t n ( ) n ( t n ( ) 0 0 = = kn ( ) kn ( t ) 0 t t n (0) k t + = k t n t n ( ) (0)(1 ) = n t ( ) 1 t k 1/ Positive only if Stable and positive for all values of t Correct asymptotic behavior n 0 as t Requires time steps shorter than shortest species lifetime Chemical systems are stiff lifetimes range over many orders of magnitude. Simulation must extend beyond longest lifetime, but explicit method would require impractically short time steps. So implicit method is required. Use higher-order approximations of dni/dt for more accuracy: Rosenbrock or Gear methods Brasseur and Jacob, chap. 6
Global cycling of carbon: todays perturbed state Preindustrial reservoirs and fluxes in black Perturbations in red: reservoirs since pre-industrial, fluxes for last decade (2009-2018)
Characteristic time scales in chemical (or other) systems d dt d dt o n How long does it take a perturbation n(0) applied at t = 0 to relax to steady state? ( ) ( = = + dt dt n Consider a system evolving as = = T for ( , n n n ( ) s n n ,... ) K 1 2 n = = with steady state solution no s(n ) 0 o d n + n d n + = s n o ) ( ) n s n J n J n o o first-order Taylor expansion s n s n where = = J with elements j i is the Jacobian matrix (KxK) of system ik n k o J has K eigenvectors ekwith eigenvalues ksuch that Jek= kek d dt d e n = = = = If , then becomes with solution If Re( k) > 0 system blows up! If Re( k) < 0 system relaxes to ss with timescale k = -1/Re( k) If Im ( k) 0) system oscillates ( ) t t k (0)exp[ ] n e J n e e e k k k k k k dt General perturbation in eigenvector basis: (0) (0) = k K decays on time scales (-1/Re( 1), -1/Re( K)) These are the K characteristic timescales of the system n e k Brasseur and Jacob, chap. 4.4.2
Example: CH4-CO-OH system 1. CH4+ OH CO + products 2. CO + OH products 3. OH + X products Loss rate constants External sources d[CH4]/dt = SCH4 -k1[CH4][OH] d[CO/dt = SCO + k1[CH4][OH] k2 [CO[OH] d[OH]/dt = SOH- k1[CH4][OH] k2 [CO[OH] - k3[X][OH] Lifetime 11 years 103 days 0.5 s J = Timescales of perturbation decay are longer than lifetimes because of positive feedbacks. CH4 causes OH Effect is big for CH4 (18 vs. 11 years): longer time scale must be used for GWP Prather, GRL9, 801-804, 1994
