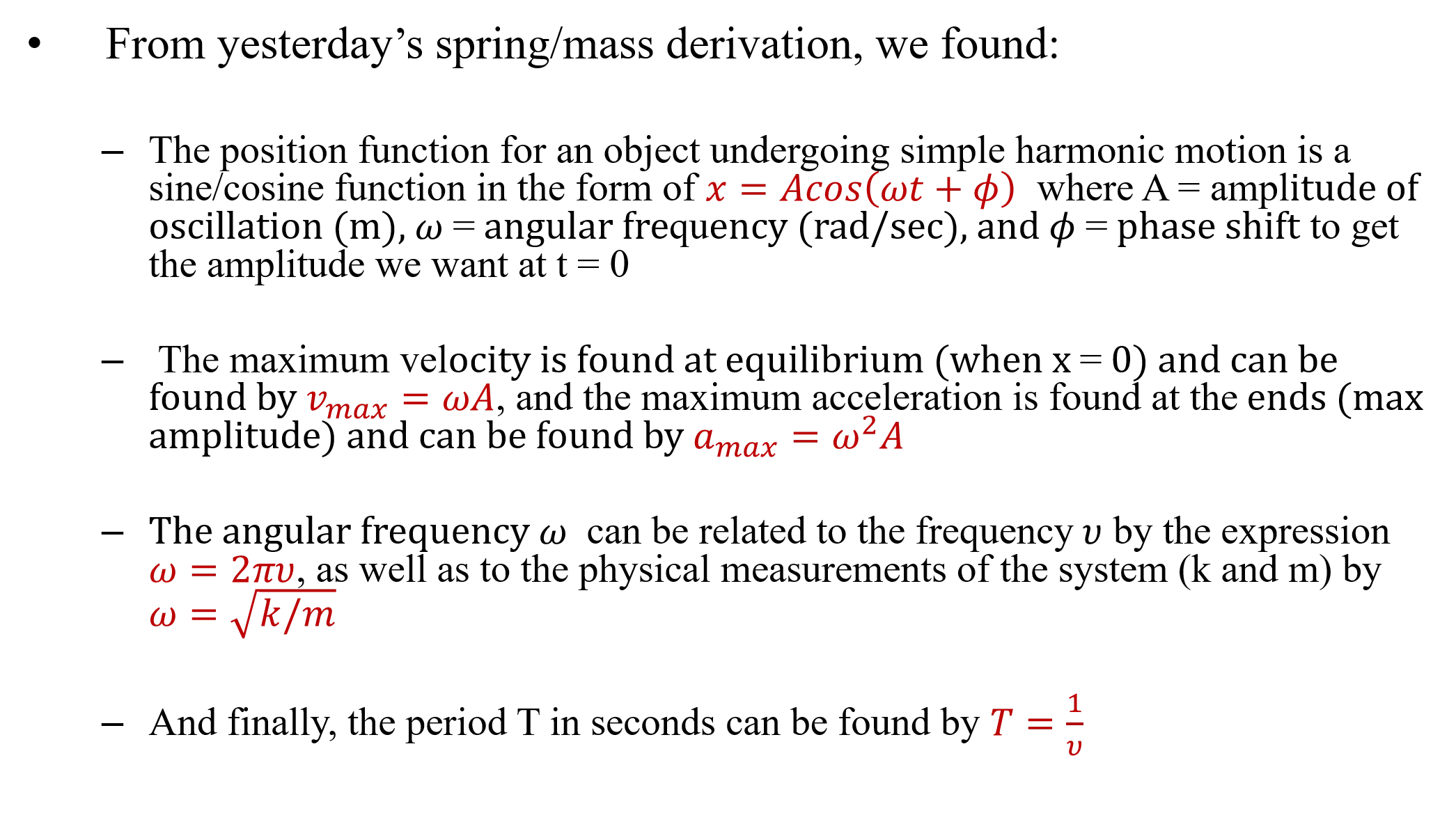Accessibility & ctcLink Open Forum - September 10, 2024

This presentation showcases the agenda for the Accessibility & ctcLink Open Forum held on September 10, 2024. It includes discussions on various topics such as HCM, CS, HCX, OKTA, OAAP, and more. Issues regarding OAAP SSN entry and College Sharing are addressed, along with updates on upcoming meetings and accessibility reviews. The session concludes with details on Service Desk Tickets/Oracle Service Requests.

• Accessibility
• ctcLink
• Forum
• OAAP
• College Sharing

dcrea Follow

Uploaded on Mar 02, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. General announcements Some reminders from yesterday: Extra Credit opportunity is posted, due by 3 pm on Wednesday, January 15 This is not a required assignment. One (1) test retake opportunity is available before the end of the semester - check your email for a survey today asking whether you plan to retake a test, which one, and which of the make up times work for you This is not a retake of the exact same test, but of the material of that unit. If you choose to retake a test, your retake score is what will count, even if it s lower. This is meant to be a chance to buckle down and master material that you had trouble with the first time, and you really should study for it and even come talk to me before the retake. This is not required. Tonight s homework: From Mr. Fletcher s Honors Physics textbook (see link on class Website under Supplementary Readings), from the Vibrations and Waves chapter, answer questions/problems 9.5, 9.6, 9.8, 9.17, and 9.21. Check your answers and correct your work in another color against his posted solutions (the same link).

2. Summing up the important equations: From yesterday s spring/mass derivation, we found: The position function for an object undergoing simple harmonic motion is a sine/cosine function in the form of ? = ???? ?? + ? oscillation (m), ? = angular frequency (rad/sec), and ? = phase shift to get the amplitude we want at t = 0 where A = amplitude of The maximum velocity is found at equilibrium (when x = 0) and can be found by ????= ??, and the maximum acceleration is found at the ends (max amplitude) and can be found by ????= ?2? The angular frequency ? can be related to the frequency ? by the expression ? = 2??, as well as to the physical measurements of the system (k and m) by ? = ?/? And finally, the period T in seconds can be found by ? =1 ?

3. Looking for patterns x F v KE PE 0 0 0 0 0 A -kA (max) 0 0 max ????= ?? 0 0 max 0 0 -A +kA (max) 0 max vint -A< x < 0 -kA < F < 0 middle middle

4. Energy in a SHO At extremes where the velocity is zero, all the energy is potential, and we know ???????=1 So at x = +/- A, Uspring = kA2 2??2 At equilibrium, x = 0 so there is no potential energy and all the energy is kinetic, and the object is moving at maximum v. So at x = 0, ?? =1 2????? =1 2 2?(??)2 Conservation of energy tells us that U + K at any point must be constant. Therefore, at any intermediate point: ????=1 2??2=1 =1 2??2+1 2 2??2 2?????

5. A Problem(13.28) Consider a position function ? ? = 0.052 ? sin(8??) a.) What is the frequency? The angular frequency is ? = 8? ???/???. Therefore we can find the frequency by ? = 2?= 4 ?? 2?=8? ? b.) What is the period? Period is the inverse of frequency, so ? =1 1 ?= 4 Hz= 0.25 s c.) What is the amplitude? The amplitude is 0.052 m (the A term in the equation) d.) When will it reach x = 0.026 meters? 0.026 ? = 0.052 ? sin 8?? 0.5 = sin 8?? ??? 10.5 = 8?? ? = 0.021 ?

6. A spring/mass problem (13.1) When a 60 kg mass is attached to a spring with a spring constant of 130 N/m. It is elongated a distance 0.13 meters from its equilibrium position. x =.13 m k =130 nt/m m = 60 kg a.) What is the force on the mass in this position? ???????= ?? = 130? 0.13 = 16.9 ? ? b.) What is the acceleration of the mass at this point? ? =??????? ? = 16.9 ? 60 ?? = 0.28 ?/?2 c.) The mass is released. What is the amplitude of the periodic motion? Amplitude is the maximum distance from equilibrium; since it was initially displaced 0.13 m, that will be the amplitude of its vibration. d.) What is the frequency of the motion? 130? 60 ??=1.47 rad/sec ? 2?=1.47 ???/??? ? = = 0.23 ?? ? ? = ?/? = 2?

7. A slightly more complex one (13.31) When a 2 kg mass is attached to a spring with a spring constant of 5 N/m. It is elongated a distance 3 meters from its equilibrium position and is released at t=0. a.) What is the force 3.5 seconds after release? b.) Through how many cycles does the body oscillate in 3.5 seconds? Hint: you need to find the function that will describe this motion See solution on class Website or notes from class

8. More with the phase shift Same problem as previous slide except this time you are told that at t = 0, the mass is at A moving away from equilibrium. What is the equation of motion? We still know amplitude and angular frequency, so we can write: ? ? = 3sin(1.58? + ?) A = 0.75(3) = 2.25 m, so we set that equal to our equation and solve for ?: 2.25 = 3sin 1.58(0) + ? 0.75 = sin? ? = ??? 1.75 = .848 ??? What does this mean? A We want to shift our t = 0 axis 0.848 rad to the right, as the sine wave is increasing its amplitude (moving away from equilibrium) A ?? -A

9. Another one What if at t = 0 the mass is at A moving towards equilibrium? The math will end up being the same, as you re still setting x = A on the left- hand side. However, you can t just blithely put +0.848 rad for your phase shift! Recognize by looking at the graph that there are two locations per cycle where the mass is at A once moving away from and once moving towards equilibrium. We want the second one so the sketch comes in really handy here: A A From the sketch, we see we want the bigger phase shift (?2). Knowing that half a cycle is ? radians, and that a sine wave is symmetrical, we can find ?2 by ? ?1. Here, ?2= ? 0.848 = 2.29. ?? -A Now we can say: ? ? = 3sin(1.58? + 2.29)

10. And lastly What if it s at A and moving away from equilibrium at t = 0? Same general procedure: set that amplitude equal to the equation, t = 0, and solve for the phase shift. You ll get a negative phase shift, and sketching it out will show that we want not the -0.848 rad directly from the calculator, but instead the bigger shift ( ? + 0.848). ?? - A Moral of the story: sketch the wave and think about what your calculator is giving you, then decide what to do about the phase shift.

11. Some interesting points Note 1: The period T of oscillation of a spring-mass system is constant no matter what the amplitude! (remember, ? = This is because the greater the amplitude, the greater the max restoring force (F = -kx = -kA) which also means a greater acceleration. The bigger amplitude means the mass has to travel more distance to get to equilibrium, but it accelerates more to do so, which evens out to keep the period the same! ?/?doesn t have an A in it ) Note 2: These same relationships work for a pendulum under certain conditions! As long as the initial angle of displacement isn t large (<~20o), a pendulum will also act as a SHO.

12. Pendulum Does a pendulum fit the same form? Let s see! A simple pendulum is shown to the right. What s the period of its motion? If we can show that this system s N.S.L. expression conforms to simple harmonic motion, we have it. As the motion is rotational, we need to sum torques about the pivot point. Torque due to tension is zero. Noting that r-perpendicular for gravity is ?????, we can write: tpin: - mg ( q L pin i T L r^= Lsinq m mg mg ) Lsinq ( )= Ipiina )d2q dt2 ( = mL2 d2q g L sinq = 0 dt2+

13. Pendulum That form isn t quite right .but if we make a small angle approximation, (that ? ) then ???? ? and: ?2? ??2+ ?2? ??2+ ? ? ? ? ???? = 0 ? = 0 This looks like simple harmonic motion! Remember, we said anything in the form acceleration + (constant)(position) = 0 is SHM, and the (constant) = ?1/2 12 g L w = Therefore, for a pendulum, we can say: ? ? 1 ?, then This also means that since ? = 2?? and ? = ? = 2? These relationships are true for any pendulum with a small angle amplitude!

14. Sample'test'ques, on'(from'Fletchs'textbook)'

15. Answers to previous slide (a) 42 N/m (b) 3.74 rad/sec (c) 0.4 m (d) .595 Hz (e) 1.68 sec (f) 3.36 J (g) 1.50 m/s (h) x = 0. (i) a = +/- 5.6 m/s2 (j) x = +/-A (k) y(t) = 0.4sin(3.74t-2.76) or y(t) = 0.4cos(3.74t+1.96)

16. Problem 13.42 disp (cm) For the sine wave shown, determine: 2 1 a.) amplitude? time (sec) 0 -1 -2 b.) period? 1 2 3 4 6 5 c.) angular frequency? d.) maximum speed? e.) maximum acceleration? f.) position as a function of time relationship using a sine function vs. a cosine function?

17. Answers to previous slide (a) 2 cm (b) 4 sec (c) 1.57 rad/sec (d) 3.14 cm/s or 0.0314 m/s (e) 4.93 cm/s2or 0.0493 m/s2 (f) x(t) = (2 cm)sin(1.57t) or x(t) = (2 cm)cos(1.57t+1.47)