Understanding the Rule of Mixtures in Composite Materials
The Rule of Mixtures (ROM) is a weighted method for predicting the properties of composite materials, such as fiber-reinforced polymers (FRP). This method relies on assumptions regarding the homogeneity and properties of fibers and matrices. By combining volume fraction and properties linearly, the
5 views • 23 slides
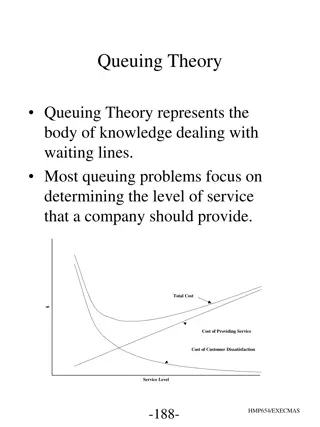
Understanding Queuing Theory and its Characteristics
Queuing Theory is the study of waiting lines and service levels in businesses. It involves analyzing customer arrival patterns, service configurations, and queuing processes such as FIFO vs. LIFO disciplines. Characteristics include the generation of customers, homogeneity of populations, and determ
1 views • 25 slides
Understanding Approximation Algorithms: Types, Terminology, and Performance Ratios
Approximation algorithms aim to find near-optimal solutions for optimization problems, with the performance ratio indicating how close the algorithm's solution is to the optimal solution. The terminology used in approximation algorithms includes P (optimization problem), C (approximation algorithm),
2 views • 10 slides
Understanding Particle-on-a-Ring Approximation in Chemistry
Delve into the fascinating world of the particle-on-a-ring approximation in chemistry, exploring concepts like quantum quantization of energy levels, De Broglie approach, Schrödinger equation, and its relevance to the electronic structure of molecules. Discover how confining particles to a ring lea
1 views • 23 slides
Exploring Binomial and Poisson Distributions in Probability Theory
Understand the fundamentals of binomial and Poisson distributions through practical examples involving oil reserve exploration and dice rolling. Learn how to calculate the mean, variance, and expected outcomes of random variables in these distributions using formulas and probability concepts.
0 views • 13 slides
Understanding Basic Concepts in Statistics
This content covers fundamental concepts in statistics such as populations, samples, models, and probability distributions. It explains the differences between populations and samples, the importance of models in describing populations, and discusses various distributions like the normal and Poisson
0 views • 42 slides
Understanding Binomial and Poisson Data Analysis
Discrete data, including Binomial and Poisson data, plays a crucial role in statistical analysis. This content explores the nature of discrete data, the concepts of Binomial and Poisson data, assumptions for Binomial distribution, mean, standard deviation, examples, and considerations for charting a
2 views • 31 slides
Understanding Probabilistic Models: Examples and Solutions
This content delves into probabilistic models, focusing on computing probabilities by conditioning, independent random variables, and Poisson distributions. Examples and solutions are provided to enhance understanding and application. It covers scenarios such as accidents in an insurance company, ge
0 views • 12 slides
Understanding Renewal Processes in Continuous Time
Renewal theory is a branch of probability theory that extends Poisson processes for various inter-arrival times. A renewal process models randomly occurring events over time, such as customer arrivals at a service station or natural phenomena like earthquakes. This article delves into the concept of
0 views • 20 slides
Understanding Overdispersed Data in SAS for Regression Analysis
Explore the concept of overdispersion in count and binary data, its causes, consequences, and how to account for it in regression analysis using SAS. Learn about Poisson and binomial distributions, along with common techniques like Poisson regression and logistic regression. Gain insights into handl
0 views • 61 slides
Understanding Invariance in Posterior Distributions
Exploring the insensitivity of posterior distributions to variations in prior distributions using a Poisson model applied to pancreas data. The analysis involves calculating posterior mean and standard deviation with different Gamma prior distributions. Results showcase minimal change in outcomes ac
0 views • 7 slides
Understanding Discrete Probability Distributions
Explore the definition of random variables, probability distributions, and three types of discrete distributions - Binomial, Hypergeometric, and Poisson. Learn about the mean, variance, and standard deviation of probability distributions, as well as the difference between discrete and continuous dis
3 views • 32 slides
Learning-Based Low-Rank Approximations and Linear Sketches
Exploring learning-based low-rank approximations and linear sketches in matrices, including techniques like dimensionality reduction, regression, and streaming algorithms. Discusses the use of random matrices, sparse matrices, and the concept of low-rank approximation through singular value decompos
0 views • 13 slides
Improved Approximation for the Directed Spanner Problem
Grigory Yaroslavtsev and collaborators present an improved approximation for the Directed Spanner Problem, exploring the concept of k-Spanner in directed graphs. The research delves into finding the sparsest k-spanner, preserving distances and discussing applications, including simulating synchroniz
0 views • 20 slides
Notch Approximation for Low-Cycle Fatigue Analysis in Structural Components
Structural components subjected to multi-axial cyclic loading can be analyzed for low-cycle fatigue using notch approximation. By transforming elastic response into an elastoplastic state, the computation time is reduced, and fatigue evaluation is done based on the Smith-Watson-Topper model. Strain-
0 views • 4 slides
Covariant Phase Space Formalism in Nonabelian Gauge Theories
The presentation focuses on the covariant phase space formalism in nonabelian gauge theories, aiming to derive the symplectic form and Poisson/Dirac brackets systematically from the Lagrangian. By applying canonical quantization methods, the structure of the infrared sector in such theories can be d
0 views • 42 slides
Functional Approximation Using Gaussian Basis Functions for Dimensionality Reduction
This paper proposes a method for dimensionality reduction based on functional approximation using Gaussian basis functions. Nonlinear Gauss weights are utilized to train a least squares support vector machine (LS-SVM) model, with further variable selection using forward-backward methodology. The met
0 views • 23 slides
Analysis of Mosquito Collection Data: Net Weight, Water Coating, and Model Fitting
This analysis includes tables detailing the weight of batch 13 nets, mean water and insecticide for coating the net, model fitting of Poisson models for mosquito count dataset, and modeling fitting of ZIP and ZINB models for trap collection data. The tables provide valuable insights into net propert
0 views • 5 slides
Approximation Algorithms for Stochastic Optimization: An Overview
This piece discusses approximation algorithms for stochastic optimization problems, focusing on modeling uncertainty in inputs, adapting to stochastic predictions, and exploring different optimization themes. It covers topics such as weakening the adversary in online stochastic optimization, two-sta
0 views • 33 slides
Understanding Geometric and Poisson Probability Distributions
Explore the geometric and Poisson probability distributions, including criteria for geometric random variables, formulas, and practical examples. Learn how to calculate probabilities using the geometric distribution and apply it in scenarios like Russian Roulette and blood donor collection. Dive int
0 views • 13 slides
Advanced NLP Modeling Techniques: Approximation-aware Training
Push beyond traditional NLP models like logistic regression and PCFG with approximation-aware training. Explore factor graphs, BP algorithm, and fancier models to improve predictions. Learn how to tweak algorithms, tune parameters, and build custom models for machine learning in NLP.
0 views • 49 slides
ACCEPT: A Programmer-Guided Compiler Framework for Practical Approximate Computing
ACCEPT is an Approximate C Compiler framework that allows programmers to designate which parts of the code can be approximated for energy and performance trade-offs. It automatically determines the best approximation parameters, identifies safe approximation areas, and can utilize FPGA for hardware
0 views • 15 slides
Regret-Bounded Vehicle Routing Approximation Algorithms
Regret-bounded vehicle routing problems aim to minimize client delays by considering client-centric views and bounded client regret measures. This involves measuring waiting times relative to shortest-path distances from the starting depot. Additive and multiplicative regret measures are used to add
0 views • 28 slides
Approximation Algorithms for Regret-Bounded Vehicle Routing
This research explores regret-bounded vehicle routing problems (VRPs) where the focus is on minimizing client delays based on their distances from the starting depot. The study introduces a client-centric view to measure regret and devises algorithms for both additive and multiplicative regret-based
0 views • 23 slides
Enhancing Processor Performance Through Rollback-Free Value Prediction
Mitigating memory and bandwidth walls, this research extends rollback-free value prediction to GPUs, achieving up to 2x improvement in energy and performance while maintaining 10% quality degradation. Utilizing microarchitecturally-triggered approximation to predict missed loads, this work focuses o
0 views • 7 slides
Birth and Death Processes in Population Dynamics
Birth and death processes in population dynamics involve the concept of how organisms reproduce and die, leading to changes in population size over time. These processes can be generalized from the Poisson process and are crucial in queuing theory and modeling dynamic systems. The differential-diffe
0 views • 20 slides
LP-Based Algorithms for Capacitated Facility Location
This research presents LP-Based Algorithms for the Capacitated Facility Location problem, aiming to choose facilities to open and assign clients to these facilities efficiently. It discusses solving the problem using metric costs, client and facility sets, capacities, and opening costs. The research
0 views • 36 slides
Iterative Root Approximation Using Natural Logarithm
The content covers iterative root approximation using natural logarithm in solving equations. It explores finding roots by iterative formulas and demonstrates calculations to reach approximate values. The process involves selecting intervals to show correct values and ensuring continuity for accurat
0 views • 14 slides
Understanding Continuous-Time Markov Chains in Manufacturing Systems
Explore the world of Continuous-Time Markov Chains (CTMC) in manufacturing systems through the lens of stochastic processes and performance analysis. Learn about basic definitions, characteristics, and behaviors of CTMC, including homogeneous CTMC and Poisson arrivals. Gain insights into the memoryl
0 views • 50 slides
Understanding Statistical Distributions in Physics
Exploring the connections between binomial, Poisson, and Gaussian distributions, this material delves into probabilities, change of variables, and cumulative distribution functions within the context of experimental methods in nuclear, particle, and astro physics. Gain insights into key concepts, su
0 views • 13 slides
Statistical Learning: Discrete Random Variables and Distributions
Explore the concepts related to discrete random variables and their corresponding probability density functions, such as Poisson Distribution and Binomial Distribution. Understand the implications of negative values in random variables, calculate expected values, and grasp the relationships between
0 views • 16 slides
Hierarchy-Based Algorithms for Minimizing Makespan under Precedence and Communication Constraints
This research discusses hierarchy-based algorithms for minimizing makespan in scheduling problems with precedence and communication constraints. Various approximation techniques, open questions in scheduling theory, and QPTAS for different settings are explored, including the possibility of beating
0 views • 25 slides
Correlation Clustering: Near-Optimal LP Rounding and Approximation Algorithms
Explore correlation clustering, a powerful clustering method using qualitative similarities. Learn about LP rounding techniques, approximation algorithms, NP-hardness, and practical applications like document deduplication. Discover insights from leading researchers and tutorials on theory and pract
0 views • 27 slides
Computational Earth Science: Solving Heat Flow in Objects with Complex Shapes Using Finite Difference Method
Explore projects involving the Finite Difference Method for solving static heat conduction problems, also known as the Poisson Equation. Topics include testing boundary conditions, symmetry of solutions, point sources, dipoles, and more. Gain insights into changing boundary conditions and understand
0 views • 20 slides
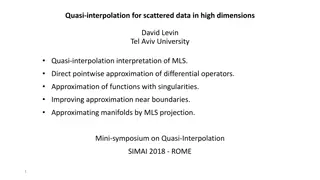
Quasi-Interpolation for Scattered Data in High Dimensions: Methods and Applications
This research explores the use of quasi-interpolation techniques to approximate functions from scattered data points in high dimensions. It discusses the interpretation of Moving Least Squares (MLS) for direct pointwise approximation of differential operators, handling singularities, and improving a
0 views • 9 slides
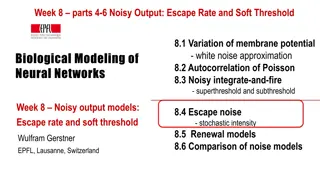
Exploring Noisy Output in Neural Networks: From Escape Rate to Soft Threshold
Delve into the intricacies of noisy output in neural networks through topics such as the variation of membrane potential with white noise approximation, autocorrelation of Poisson processes, and the effects of noise on integrate-and-fire systems, both superthreshold and subthreshold. This exploratio
0 views • 34 slides
Advanced Techniques in Multivariate Approximation for Improved Function Approximation
Explore characteristics and properties of good approximation operators, such as quasi-interpolation and Moving Least-Squares (MLS), for approximating functions with singularities and near boundaries. Learn about direct approximation of local functionals and high-order approximation methods for non-s
0 views • 9 slides
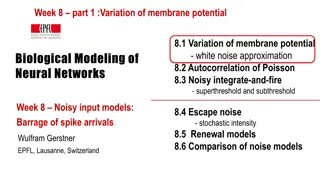
Exploring Membrane Potential Variations in Neural Networks
Delve into the dynamics of membrane potential variations in neural networks through topics like white noise approximation, autocorrelation of Poisson processes, and the Noisy Integrate-and-Fire model. Investigate how these variations manifest at different thresholds, shedding light on the biological
0 views • 63 slides
Understanding Probability Distributions in Engineering Mathematics-III
Explore the concept of random variables, types of distributions such as binomial, hypergeometric, and Poisson, and the distinction between discrete and continuous variables. Enhance your knowledge of probability distributions with practical examples and application scenarios.
0 views • 57 slides
LP-Based Approximation Algorithms for Multi-Vehicle Minimum Latency Problems
The research discusses LP-based approximation algorithms for solving Multi-Vehicle Minimum Latency Problems, focusing on minimizing waiting times for vehicles visiting clients starting from a depot. Various cases, including single- and multi-depot scenarios, are explored, and significant improvement
0 views • 28 slides