Calculating the Area of a Farmhouse Attic Floor
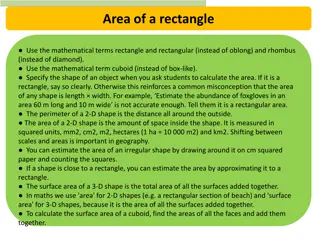
In this mathematical problem, we are tasked with finding the area of the attic floor of a farmhouse depicted as a pyramid-shaped roof. The model provided includes the measurements necessary for our calculations, such as the attic floor being a square and all edges of the pyramid having a length of 12m. By applying geometric principles and formulas, we can determine the area of the attic floor accurately.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? Here you see a photograph of a farmhouse with a roof in the shape of a pyramid. What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Next to it is a student s mathematical model of the farmhouse roof with measurements added. What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.1 Calculate the area of the attic floor ABCD.
Mathematics Unit 1: Farms What do we want to find out? Here you see a photograph of a farmhouse with a roof in the shape of a pyramid. What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Next to it is a student s mathematical model of the farmhouse roof with measurements added. What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.1 Calculate the area of the attic floor ABCD. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? Here you see a photograph of a farmhouse with a roof in the shape of a pyramid. What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Next to it is a student s mathematical model of the farmhouse roof with measurements added. What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.1 Calculate the area of the attic floor ABCD. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? Here you see a photograph of a farmhouse with a roof in the shape of a pyramid. What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? Next to it is a student s mathematical model of the farmhouse roof with measurements added. What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.1 Calculate the area of the attic floor ABCD. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? Here you see a photograph of a farmhouse with a roof in the shape of a pyramid. What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Next to it is a student s mathematical model of the farmhouse roof with measurements added. What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.1 Calculate the area of the attic floor ABCD. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.2 Calculate the length of EF, one of the horizontal edges of the block.
Mathematics Unit 1: Farms What do we want to find out? What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.2 Calculate the length of EF, one of the horizontal edges of the block. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.2 Calculate the length of EF, one of the horizontal edges of the block. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What have we learned? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.2 Calculate the length of EF, one of the horizontal edges of the block. Back to start Back to start
Mathematics Unit 1: Farms What do we want to find out? What do we want to find out? What useful information do we know? What useful information do we know? What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? What have we learned? The attic floor, ABCD in the model, is a square. The beams that support the roof are the edges of a block (rectangular prism) EFGHKLMN. E is the middle of AT, F is the middle of BT, G is the middle of CT and H is the middle of DT. All the edges of the pyramid in the model have length 12m. QUESTION 1.2 Calculate the length of EF, one of the horizontal edges of the block. Back to start Back to start