Applications of Calculus in Optimization Problems

Calculus plays a crucial role in solving optimization problems to find maximum or minimum values in various real-life scenarios. This content provides examples of optimizing for maximum profit, area, distance, and volume using calculus concepts. From finding optimal dimensions for fencing to maximizing volume within constraints, the applications are explored with step-by-step solutions.

• Calculus
• Optimization
• Applications
• Maximum
• Minimum

lgit Follow

Uploaded on Sep 20, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

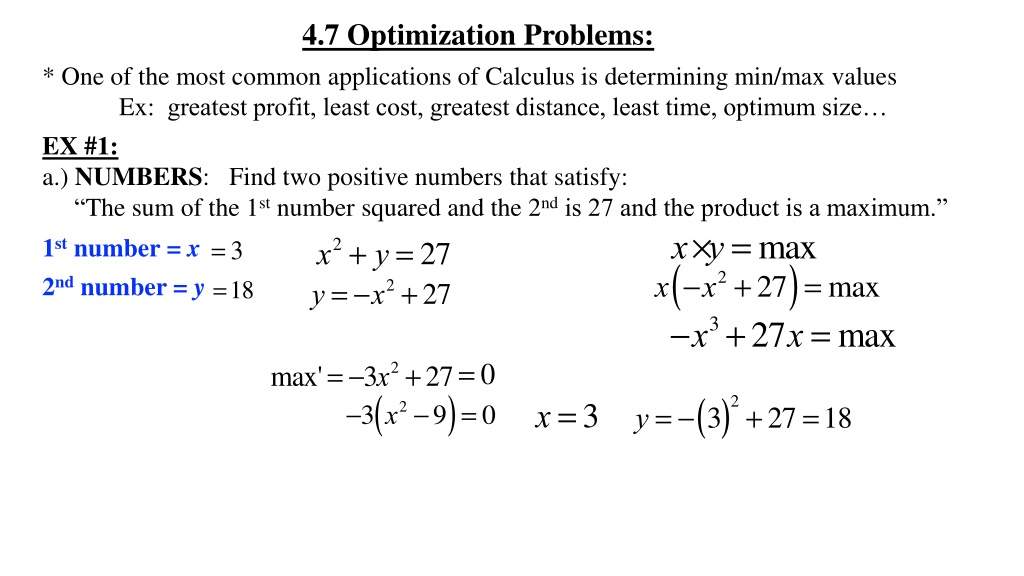
1. 4.7 Optimization Problems: * One of the most common applications of Calculus is determining min/max values Ex: greatest profit, least cost, greatest distance, least time, optimum size EX #1: a.) NUMBERS: Find two positive numbers that satisfy: The sum of the 1st number squared and the 2ndis 27 and the product is a maximum. 1st number = x 2nd number = y y = -x2+27 x y = max x -x2+27 ( -x3+27x = max x2+ y = 27 =3 =18 )= max max'= -3x2+27=0 -3 x2-9 ( )= 0 y = - 3 ( ) 2+27 =18 x =3

2. Steps to follow when optimizing: max or min Write an ____________ you want to optimize (find the _________________ of ) equation Use another _____________ (if needed) to help you rewrite with ___________ variable equation 0 or DNE a single critical numbers Calculate the ____________ and set = ____________to find ____________________ derivative relative extrema Determine if the critical number is a ______________________ Determine __________________________________________ (what x-values make sense) the reasonableness of your solutions

3. b.) A farmer has 2400 ft. of fencing and wants to fence off a rectangular field that borders a straight river. He needs no fence along the river. What are the dimensions of the field that has the largest area? Area= x y Area = x 2400-2x ( Area= 2400x-2x2 Area'=2400-4x =0 2400= 4x 600= x y x x Width = x Length = y ) river 2x+ y = 2400 y = 2400-2x y = 2400-2 600 y =1200 ( ) Width = 600 ft. Length = 1200 ft.

4. ( ) c.) DISTANCE: Which points on the graph of y = 4 x2 are closest to the point (0, 2)? = x2,y2 = x,4- x2 ( ) ( ) = x1,y1 Unknown point = (x, y) Minimum distance 3 2,5 (x, y) 2 2 2x 2x2-3 ( )= 0 ( ) ) ( ) = x2+4-4x2+ x4 2+ y1- y2 ( 2 d = x1- x2 ( 3 2 x = 0, ) ) = x4-3x2+4 4x3-6x 2 x4-3x2+4 2+ y-2 2 = x-0 d = 04-3 0 ( ) d'= x2+ 4- x2-2 ( ( 2+4 = 2 2 = 4 2 9 4-9 3 2 3 2 +4 = 2+4 ) d = -3 2 = x2+ 2- x2 2 7 4 1.3229 Less than 2 = 4 3 2=5 3 2 = y = 4- x2= 4- 2

5. d.) VOLUME: A manufacture wants to design an open box having a square base and a surface area of 108 square inches. What dimensions will produce a box w/maximum volume? VOLUME = L*W*H SURFACE AREA = 108 V = x2 y x2+4xy =108 V = x2 108- x2 4x V =108x- x3 4 V = 27x-1 4x3 x =6 OPEN y =108- x2 y 4x V '= 27-3 y =108- 6 ( ) 4x2=0 4x2 4 2 x 4 6 ( ) 24= 3 x 27 =3 3 36= x2 4 y =72 3 Dimensions should be 6 in by 6 in by 3 in

6. e.) AREA: A rectangular page is to contain 24 square inches of print. The margins at the top and bottom are to be 1 inches, and the margins on the left and right are to be 1 inch. What should the dimensions of the page be so that the least amount of paper is used? A= xy x-2 24 x-2 ( 24 x-2 ( 3x+18 ( x-2 ( 3 x2-4x+12 ( 3 x-6 ( x = 6,but not -2 ( ) y-3 ( )= 24 ( ) 1 ( ) 6x+18 ( )- 3x2+18x x-2 ) )- 3x2+18x 24 in2 x-2 ( ) A'= 3x+18 x-2 ( ( ) y y-3 ( 2 y-3= A= x ) ) ( )=0 ( 6x2+18x-12x-36-3x2-18x =0 3x2-12x-36= 0 )= 0 ) x+2 ) 6x+18 ( ( ) x-2 x-2 y = +3 ( ) ) 3x2+18x x-2 ( x ) A= ) y = 24 6-2+3= 9 Dimensions should be 6 in by 9 in ) y = ( )= 0

7. Optimization using CALC: DO THEM W/ME #1: a.) LENGTH: Two posts, one 12 feet high and the other 28 feet high, stand 30 feet apart. They are to be stayed by two wires, attached to a single stake, running from ground level to the top of each post. Where should the stake be placed to use the least amount of wire? ( x2y2+282 ( x2y2+282x2= x2y2+122y2 282x2=122y2 28x =12y 7x =3y ) ( ) 2 2 y2+282 d = x2+122+ 2x 2 x2+122+ x x2+122+ x x2+122= y2+282 2y y' 2 y2+282 y -1 ( ) y2+282= 0 y y2+282 x y2+282= y x2+122 )= y2x2+122 28 x2+122 ( ) d'= 12 y x 30 y =30- x y'= -1 7x = 3 30- x ( 10x = 90 x = 9 )=90-3x ft. from the 12 ft. post

8. b.) AN ENDPOINT MAXIMUM: Four feet of wire is to be used to form a square and a circle. How much of the wire should be used for the square and how much should be used for the circle to enclose the maximum total area? Max Area= x2+pr2 2 1- x ( p A= x2+p 4 1- x p2 A= x2+4 p A'= 2x+4 p 2 1- x p + x = 4 ft A'= 2x+8 px-8 px =8 x 2+8 p x =8 p ) 2 p=0 x A= x2+p 4x+2pr = 4 r =4-4x 2p 2x+8 ( ) ( ) 2 =2 1- x p p =8 1- x ( ) 2 p 2.24 ft 4 4x = 4 1 p +4 8 4 ( ) -1 ( ) = 2p +8= Square =2.24 ft. Circle = 4 2.24 = 1.76 ft. p +4 2+8