Polynomial Long Division Review and Practice

Slide Note

This content provides a detailed review on polynomial long division including step-by-step instructions, examples, and synthetic division practice problems. It covers topics such as descending polynomial order, solving binomial divisors, writing coefficients, determining remainders, and obtaining final answers. The included images further illustrate the processes involved in polynomial long division and synthetic division.

bwhi Follow

Uploaded on Sep 14, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

E N D

Presentation Transcript

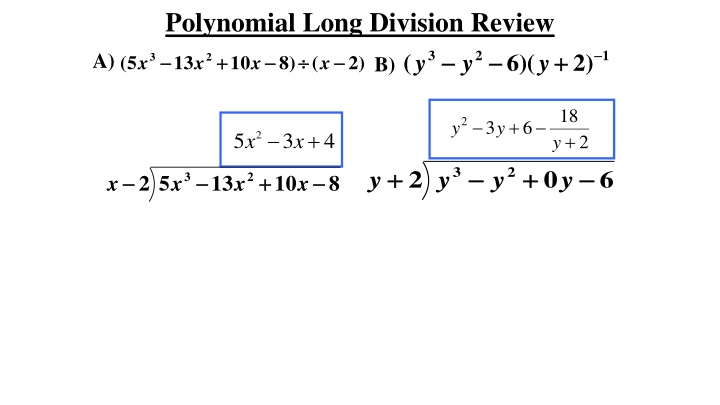
Polynomial Long Division Review + + 3 2 1 A) y y y ( 6 )( ) 2 + + 3 2 x x x x 5 ( 13 10 ) 8 ( ) 2 B) 18 + y 0 2 + 3 6 y y x + 5 3 4 2 x 2 + + + + 3 2 y y y y 2 6 + + 3 2 x x x x 2 5 13 10 8

SYNTHETIC DIVISION: STEP #1:Be sure the polynomial is in stardanrd form with no gaps Descending Polynomial + + 3 2 x x x x 5 ( 13 10 ) 8 ( ) 2 + + 3 2 Order x x x : 5 13 10 8 STEP #2: Solve the Binomial Divisor = Zero (this is just the opposite of the constant in the divisor) ; 0 2 = = x = = x 2 STEP #3: Write the ZERO-value, then all the COEFFICIENTS of Polynomial. 2 5 -13 10 -8

5 -13 10 -8 10 2 -6 8 5 -3 0 = Remainder 4 STEP #4: Last Answer is your REMAINDER Write the coefficient answers in descending order starting with a Degree ONE LESS THAN Original Degree and include NONZERO REMAINDER OVER DIVISOR at end 5 x x + + 2 3 4 5 -3 4 + + = = 3 2 x x x x 5 ( 13 10 ) 8 ( ) 2 x + + 2 x 5 3 4 SAME ANSWER AS LONG DIVISION!!!!