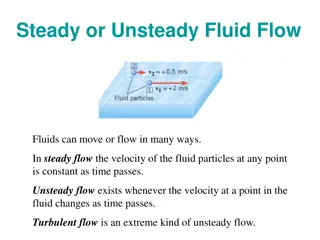
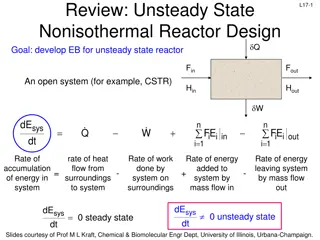
Unsteady MHD Poiseuille Flow Through a Porous Channel

This study investigates unsteady magnetohydrodynamic (MHD) Poiseuille flow through a porous channel under an oscillating pressure gradient and uniform suction/injection. The objective is to obtain numerical solutions for the velocity distribution and analyze how the velocity is affected by various parameters. The Galerkin Finite Element Method is used for numerical solution, with MATLAB software employed for computations. The governing equation, initial and boundary conditions, as well as non-dimensionalization of equations are discussed.

• Unsteady flow
• MHD
• Poiseuille flow
• Porous channel
• Galerkin method

bri_th Follow

Uploaded on Oct 10, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. UNSTEADY MHD POISEUILLE FLOW THROUGH A POROUS CHANNEL UNDER AN OSCILLATING PRESSURE GRADIENT AND UNIFORM SUCTION/INJECTION Judith N. Balkissoon1*, Sreedhara Rao Gunakala2 and Victor M. Job3 1,2,3Faculty of Science and Technology, The University of the West Indies, Trinidad 1Email: judith.balkissoon@my.uwi.edu *(Corresponding author) 2Email: Sreedhara.Rao@sta.uwi.edu 3Email: victor.job@sta.uwi.edu IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

2. INTRODUCTION Hydromagnetics/Magnetohydrodynamics Poiseuille Flow Brinkman s Equation IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

3. OBJECTIVES The objectives of this study are: 1. To obtain numerical solutions for the velocity distribution for this flow. 2. To determine how velocity is affected by: Hartmann number (??) Frequency of oscillation (?) Suction/injection parameter (S) Permeability parameter ( ) Time (?) Amplitude of the pressure gradient (?) IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

4. METHODOLOGY The Galerkin Finite Element Method was used to solve the governing equation numerically under the initial and boundary conditions. The weighted integral method was used for the spatial semi- discretization. The Crank-Nicolson scheme was used for the time discretization. The elements were assembled and the computer software MATLAB was used to solve the system of equations. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

5. DESCRIPTION OF PROBLEM Figure 1 IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

6. GOVERNING EQUATION Brinkman s Equation: ??+ ??2? ?? ??+ ?0 ?? ?? = ?? 2? ? ? ??2 ??0 ??(1) Initial and Boundary Conditions are: ? = 0 at ? = 0 ? = 0 at ? = for ? > 0 (2) IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

7. Pressure Gradient: ?? ??= ?(1 ??? ??) (3) Non-dimensionalisingequation (1): ??=?2? ?? ??+ ??? ??2 ??2+ ?2? + ? 1 ??? ?? (4) The non-dimensional initial and boundary conditions become: ? = 0 at ? = 0 and ? = 0 at ? = 1 at ? > 0 (5) IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

8. RESULTS Figure 2: Velocity profile for Poiseuille flow for different values of ?. Figure 3: Velocity profile for Poiseuille flow for different values of ? and ? = 0.00001. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

9. RESULTS Figure 4: Velocity profiles for Poiseuille flow for different values of ??. Figure 5: Effect of ?? number on time variation of ? at ? = 0. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

10. RESULTS Figure 6: Velocity profiles for Poiseuille flow for different values of ?. Figure 7: Effect of ? on time variation of ? at ? = 0. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

11. RESULTS Figure 8: Time variation of u for varying values of A with ? = 1 at ? = 0 IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

12. RESULTS Figure 10: Effect of ? on time variation of ?. Figure 9: Effect of ? on time variation of ?. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

13. According to Tosun [22] the volumetric flow rate is determined by integrating the velocity with respect to the area of the cross-section of the channel, that is perpendicular to the direction in which the fluid is flowing, i.e. ?(?)?? 1 parameter (?), the suction/injection parameter (?), Hartmann number (??) and the frequency of oscillation (?). From Fig. 10 to 13 it is observed that as ?,? and ?? decrease, the volumetric rate of flow (?) increases, however, as ? increases, ? also increases. A study done by Shit and Roy [13] found that when an external magnetic field is applied to a system, the volumetric flow rate decreases. 1 . Fig. 10 to Fig. 13 below show the effect of time variation of the permeability RESULTS Figure 11: Effect of ?? on time variation of ?. Figure 12: Effect of ? on time variation of ?. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

14. CONCLUSION The velocity increases when the permeability parameter, suction/injection parameter, Hartmann number and frequency of oscillation decrease. The velocity increases when the amplitude of the pressure gradient increases. The volumetric flow rate (?) was observed to increase as the permeability parameter, suction/injection parameter and Hartmann number decreased but it increased as the frequency of oscillation increased. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

15. REFERENCES A. A. Moniem and W. S. Hassanin, Solution of MHD flow past a vertical porous plate through a porous medium under oscillatory suction, Applied Mathematics, vol. 4, no. 04, pp. 694 694, 2013. H. A. Attia and M. A. M. Abdeen, Unsteady Hartmann flow with heat transfer of a viscoelastic fluid under exponential decaying pressure gradient, Engineering Mechanics, vol. 19, no. 5, pp. 37 44, 2012. P. A. Davidson, An Introduction to Magnetohydrodynamics. 40 West 20th Street, New York: Cambridge University Press, 2001. V. G. Gupta and A. Jain, An analysis of unsteady MHD Couette flow and heat transfer in a rotating horizontal channel with injection/suction, 2016.M. Parvazinia, V. Nassehi, R. J. Wakeman, and M. H. R. Ghoreishy, Finite element modelling of flow through a porous medium between two parallel plates using the brinkman equation, Transport in porous media, vol. 63, no. 1, pp. 71 90, 2006. V. K. Verma and A. K. Gupta, Mhd flow in a porous channel with constant suction/injection at the walls, International Journal of Pure and Applied Mathematics, vol. 118, no. 1, pp. 111 123, 2018. IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago

16. Questions? THANK YOU! IConETech-2020, Faculty of Engineering, The UWI, St. Augustine, Trinidad and Tobago