Unraveling the Mysteries of Ancient Babylon and Polygons
Delve into the intriguing history of ancient Babylon and its influence on our numerical systems, from the concept of polygons to the origin of 360 degrees in a circle. Explore the significance of base 60, Babylonian writing methods, and the enduring legacy of Babylonian scribes. Uncover how Babylon's innovations shaped modern calculations and measurements.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Puzzling Polygons Rebecca Klemm, PhD September 14, 2015
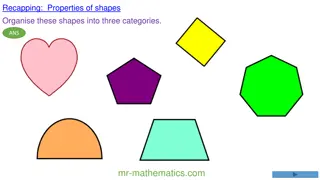
What is a Polygon?
A closed shape created with straight lines
Must all of the sides of a polygon be the same length?
No. Only equilateral polygons have equal length sides.
Why are there 360 degrees in a circle?
The circle dates back to Babylon whose numeric system was a base 60 system.
Thanks to Babylon, we have 60 seconds in a minute and 60 seconds in an hour. The year was believed to have 360 days.
Where in the world is the location of ancient Babylon?
1, 2,3,4,5,6, 10, 15, 30, 60
What type of writing did the scribes of Babylon use?
Babylonian (Sumerian) Clay Tablet and Stylus
From Clay Tablet/Stylus to ModernTablet/Stylus modern tablet and stylus.
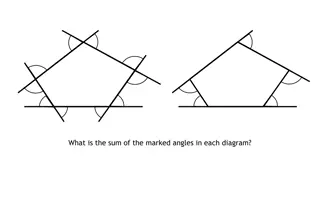
Interior and Exterior Angles of Polygons
Triangles3 sides; 3 angles
Triangles3 sides; 3 angles 60 + 60 +60 = 180
Triangles3 sides; 3 angles
Quadrilaterals4 sides; 4 angles 45 45 45 45
Quadrilaterals4 sides; 4 angles 90 +90 +90 +90 = 360
Quadrilaterals4 sides; 4 angles Sum of interior angles still 360 degrees?
Each angle if equilateral polygon 60 Type of polygon # Interior angles sides Triangle Quadrilat eral 3 180 4 360 90 Pentagon 5 540 108 Hexagon Heptagon (or Septagon) Octagon Nonagon 6 720 120 7 900 128.57... 8 9 1080 1260 135 140
General Pattern of Sum of Interior Angles of any Polygon with n sides Each angle if equilateral polygon Type of polygon # Interior angles sides Any Polygon n (n-2) 180 (n-2) 180 / n
Number Links: Animals 0- glass lizard 1- narwhal 2- camel 3- three-toed sloth 4- kitten 5- starfish 6- bee in honeycomb 7- ladybug 8- octopus 9- nine-spotted moth
Number Links: Musical Instruments 0- drum (North America) 1- ektara (India) 2- ehru (China) 3- balalaika (Russia) 4- ukelele (Hawaii) 5- banjo (orig. Africa) 6- guitar (Spain) 7- guqin (China) 8- mandolin (Italy) 9- kelstone (Belgium)
*The Numbers Lady Challenge Prove: For any Equilateral Polygon of n sides, you can deconstruct it into k polygons of n sides.