Understanding Upper and Lower Bounds in Measurements
Explore the concept of upper and lower bounds in measurements through examples involving rounding numbers and calculating boundaries for different units like centimeters, millimeters, and meters. Learn how to determine the range within which a rounded number falls for both discrete and continuous data.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
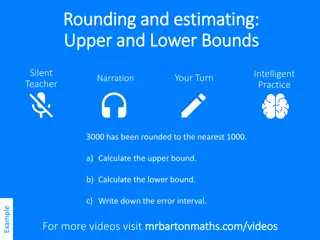
12 September 2024 Upper and lower bounds of rounded numbers LO: To determine the boundaries of a rounded number. www.mathssupport.org
Measuring with a ruler in centimetres It s 11 cm long It s 11 cm long The scale of the ruler is in centimetres. We approximate the length of the fish by recording the value at the nearest centimetre mark, that both fish are 11 cm long. So, a fish 11 cm long could be 10.5 cm to 11.5 cm long. www.mathssupport.org
Measuring with a ruler in millimetres It s 10.6 cm long Which is 106 mm long A measurement is accurate to half of the smallest division on the scale The measuring device is accurate to the nearest half mm The actual length is in the range 105.5 mm to 106.5 mm Which is in between 10.55 cm to 10.65 cm 10.55 cm < l < 10.65 cm It s 11.5 cm long Which is 115 mm long The actual length is in the range 114.5 mm to 115.5 mm Which is in between 11.45 cm to 11.55 cm 11.45 cm < l < 11.55 cm www.mathssupport.org
Upper and lower bounds for continuous data The height of the Eiffel Tower is 324 meters to the nearest meter. What is the least this measurement could be? The least this measurement could be before being rounded up is: 323.5 m What is the most this measurement could be? The most this measurement could be before being rounded down is up to but not including: 324.5 m www.mathssupport.org
Upper and lower bounds for continuous data Here are some more examples of upper and lower bounds Units metres centimetres litres kilograms hours minutes Size 21 m 7 cm 36 litres 56 kg 180 hours 53 minutes 52.5 minutes 53.5 minutes Lower B. 20.5 m 6.5 cm 35.5 litres 55.5 kg 179.5 hours Upper B. 21.5 m 7.5 cm 36.5 litres 56.6 kg 180.5 hours www.mathssupport.org
Upper and lower bounds for calculations A rectangular block of wood was measured as 10 cm by 4 cm 10 cm What are the boundary values for its perimeter? 4 cm Size 10 cm 4 cm Lower B. 9.5 cm 3.5 cm Upper B. 10.5 cm 4.5 cm Lower Boundary of the Perimeter 2 x 9.5 + 2 x 3.5 = 26 cm Upper Boundary of the Perimeter 2 x 10.5 + 2 x 4.5 = 30 cm The perimeter is between 26 cm and 30 cm, which is 28 2cm www.mathssupport.org
Upper and lower bounds for calculations A rectangular block of wood was measured as 10 cm by 4 cm 10 cm What are the boundary values for its actual area? 4 cm Size 10 cm 4 cm Lower B. 9.5 cm 3.5 cm Upper B. 10.5 cm 4.5 cm Lower Bound Area 9.5 x 3.5 = 33.25 cm2 Upper Bound Area 10.5 x 4.5 = 47.25 cm2 The Area is between 33.25 cm2 and 47.25 cm2. This could also be represented as 33.25 + 47.25 47.25 - 33.25 2 2 which is 40.25 7 cm2 cm2 www.mathssupport.org
Upper and lower bounds for calculations What are the boundary values for the actual volume? 8 cm 6 cm 20 cm Size 20 cm 6 cm 8 cm Lower B. 19.5 cm 5.5 cm 7.5 cm Upper B. 20.5 cm 6.5 cm 8.5 cm Lower Boundary of the Volume 19.5 x 5.5 x 7.5 = 804.375 cm3 Upper Boundary of the Volume 20.5 x 6.5 x 8.5 = 1132.625 cm3 The Volume is between 804.375 cm3 and 1132.625 cm3. This could 804.375 + 1132.625 2 1132.625 804.375 2 cm3 also be represented as which is 968.5 164.125 cm3 www.mathssupport.org
Thank you for using resources from A close up of a cage Description automatically generated For more resources visit our website https://www.mathssupport.org If you have a special request, drop us an email info@mathssupport.org www.mathssupport.org